A disc A of radius R suspended by an elastic threat between two stationary plane ( figure ) performs torsional oscillations about its axis OO′ . The moment of inertia of the disc relative to that axis is equal to I, the clearance between the disc and each of the planes is equal to h, with h<<R. Find the viscosity of the gas surrounding the disc A if the oscilaltion period of the disc equals T and the logarithmic damping decrement, λ.
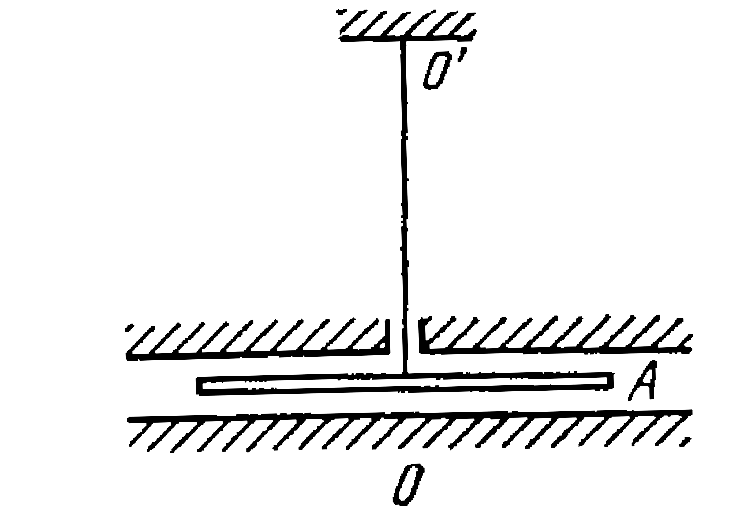
From the law of viscosity, force per unit are =ηdvdx
so when the disc executes torsional oscillations the resistive couple on it is
=∫R0η.2πr.rφh.r.dr×2=ηπR4h.φ
( factor 2 for the two sides of the disc, see the figure in the blook )
where φ is torsion. The equation of motion is
I..φ+ηπR4h.φ+cφ+0
Comparing with ..φ+2β.φ+ω20=0 we get
β=ηπR4/2hI
Now the logarithmic decrement λ is given by λ=βT,T= time period
Thus η=2λI/πR4T
Topper's Solved these Questions
- BOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - OSCILLATIONS AND WAVESEXERCISE - Electric Oscillations56videos
OSCILLATIONS AND WAVES
- BOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - OSCILLATIONS AND WAVESEXERCISE - Elastic Waves39videos
OSCILLATIONS AND WAVES
- BOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - OPTICSEXERCISE - Exercise2videos
OPTICS
- BOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - PHYSICAL FUNDAMENTALS OF MECHANICSEXERCISE - Relativistic Mechanics49videos
PHYSICAL FUNDAMENTALS OF MECHANICS
Similar Questions
Moment of inertia of a disc about its own axis is I. Its moment of inertia about a tangential axis in its plane is
View SolutionThe mass of a thin circular plate is M and its radius is R. About an axis in the plane of plate at a perpendicular distance R/2 from centre of plate, its moment of inertia is
View Solution
Knowledge Check
- Question 1 - Select One
The moment of inertia of a circular disc about an axis passing through the circumstances perpendicular to the plane of the disc is
A(MR2B32MR[^(2)CMR22D54MR2 - Question 2 - Select One
Moment of inertia of a disc about its own axis is I. Its moment of inertia about a tangential axis in its plane is
A52IB3IC32ID2I - Question 3 - Select One
The moment of inertia of a copper disc, rotating about an axis passing through its centre and perpendicular to its plane
Aincreases if its temperature is increasedBchanges if its axis of rotation is changedCincreases if its angular velocity is increasedDboth (a) and (b) are correct
A uniform disc of radius R=20cm has a round cut as shown in figure. The mass of the remaining (shaded) portion of the disc equals m=7.3kg. Find the moment of inertia of such a disc relative to the axis passing through its centre of inertia and perpendicular to the plane of the disc.
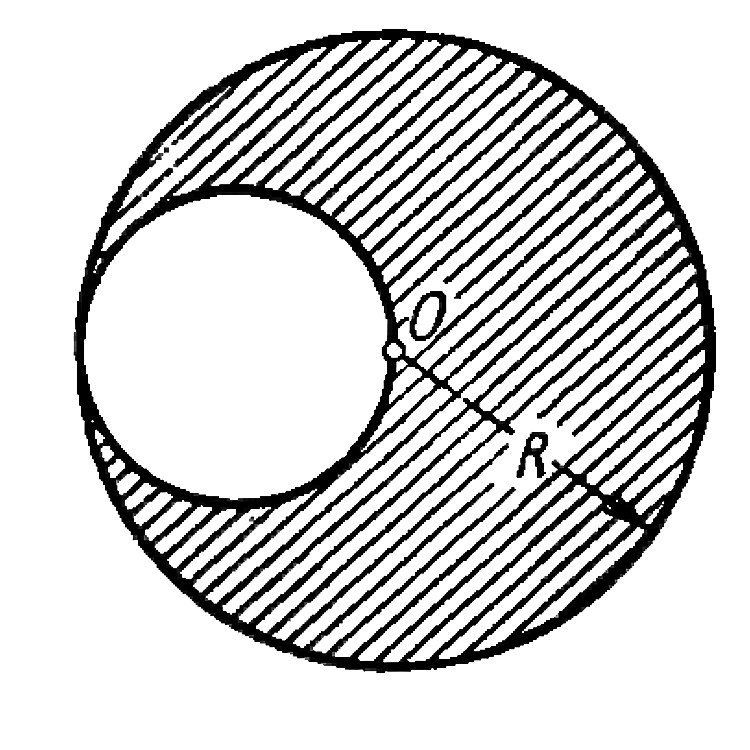 View Solution
View SolutionThe moment of inertia of a disc of mass M and radius R about a tangent to its rim in its plane is
View SolutionThe moment of inertia of a disc of mass M and radius R about an axis. Which is tangential to sircumference of disc and parallel to its diameter is.
View SolutionThe moment of inertia of a uniform circular disc of radius R and mass M about an axis passing from the edge of the disc and normal to the disc is.
View SolutionThe moment of inertia of a uniform circular disc of radius R and mass M about an axis passing from the edge of the disc and normal to the disc is
View Solution

