A uniform rod of length l and mass 2 m rests on a smooth horizontal table. A point mass m moving horizontally at right angles to the rod with velocity v collides with one end of the rod and sticks it. Then
16mv2
13mv2
mv2
mv25
The correct Answer is:A
To solve the problem step by step, we will analyze the scenario of a point mass colliding with a uniform rod and calculate the loss in kinetic energy.
Step 1: Understand the System
We have a uniform rod of length l and mass 2m resting on a smooth horizontal table. A point mass m is moving horizontally with velocity v and collides with one end of the rod.
Step 2: Apply Conservation of Linear Momentum
Before the collision, the linear momentum of the system is only due to the point mass m:
pinitial=mv
After the collision, the point mass sticks to the rod, and the system (rod + point mass) will move with some angular velocity ω. The total mass after the collision is 2m+m=3m.
Using conservation of linear momentum:
mv=(3m)vfinal
This gives us:
vfinal=v3
Step 3: Calculate the Moment of Inertia
The moment of inertia I of the rod about its center is:
Irod=13(2m)l2=2ml23
The moment of inertia of the point mass m at a distance l from the center of the rod is:
Ipoint mass=m⋅l2
Thus, the total moment of inertia Itotal of the system after the collision is:
Itotal=2ml23+ml2=5ml23
Step 4: Relate Linear and Angular Velocity
The linear velocity vfinal is related to the angular velocity ω by:
vfinal=l2ω
Substituting vfinal=v3:
v3=l2ω⟹ω=2v3l
Step 5: Calculate the Kinetic Energy After Collision
The total kinetic energy KE of the system after the collision is the sum of translational and rotational kinetic energy:
KE=12(3m)v2final+12Itotalω2
Substituting vfinal=v3 and Itotal=5ml23:
KE=12(3m)(v3)2+12(5ml23)(2v3l)2
Calculating each term:
1. Translational kinetic energy:
KEtrans=12(3m)(v29)=mv26
2. Rotational kinetic energy:
KErot=12(5ml23)(4v29l2)=10mv254=5mv227
Thus, the total kinetic energy after the collision is:
KE=mv26+5mv227
Step 6: Calculate the Initial Kinetic Energy
The initial kinetic energy of the point mass m before the collision is:
KEinitial=12mv2
Step 7: Calculate the Loss in Kinetic Energy
The loss in kinetic energy ΔKE is:
ΔKE=KEinitial−KEfinal=12mv2−(mv26+5mv227)
Calculating KEfinal:
KEfinal=mv26+5mv227=9mv254+10mv254=19mv254
Thus, the loss in kinetic energy becomes:
ΔKE=27mv254−19mv254=8mv254=4mv227
Final Result
The loss in kinetic energy due to the impact is:
ΔKE=4mv227
We have a uniform rod of length l and mass 2m resting on a smooth horizontal table. A point mass m is moving horizontally with velocity v and collides with one end of the rod.
Before the collision, the linear momentum of the system is only due to the point mass m:
pinitial=mv
After the collision, the point mass sticks to the rod, and the system (rod + point mass) will move with some angular velocity ω. The total mass after the collision is 2m+m=3m.
mv=(3m)vfinal
This gives us:
vfinal=v3
The moment of inertia I of the rod about its center is:
Irod=13(2m)l2=2ml23
The moment of inertia of the point mass m at a distance l from the center of the rod is:
Ipoint mass=m⋅l2
Thus, the total moment of inertia Itotal of the system after the collision is:
Itotal=2ml23+ml2=5ml23
The linear velocity vfinal is related to the angular velocity ω by:
vfinal=l2ω
Substituting vfinal=v3:
v3=l2ω⟹ω=2v3l
The total kinetic energy KE of the system after the collision is the sum of translational and rotational kinetic energy:
KE=12(3m)v2final+12Itotalω2
Substituting vfinal=v3 and Itotal=5ml23:
KE=12(3m)(v3)2+12(5ml23)(2v3l)2
Calculating each term:
1. Translational kinetic energy:
KEtrans=12(3m)(v29)=mv26
2. Rotational kinetic energy:
KErot=12(5ml23)(4v29l2)=10mv254=5mv227
Thus, the total kinetic energy after the collision is:
KE=mv26+5mv227
The initial kinetic energy of the point mass m before the collision is:
KEinitial=12mv2
The loss in kinetic energy ΔKE is:
ΔKE=KEinitial−KEfinal=12mv2−(mv26+5mv227)
Calculating KEfinal:
KEfinal=mv26+5mv227=9mv254+10mv254=19mv254
Thus, the loss in kinetic energy becomes:
ΔKE=27mv254−19mv254=8mv254=4mv227
The loss in kinetic energy due to the impact is:
ΔKE=4mv227
Topper's Solved these Questions
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - RIGID BODY DYNAMICS 2EXERCISE - Integer7videos
RIGID BODY DYNAMICS 2
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - RIGID BODY DYNAMICS 2EXERCISE - Fill In The Blanks7videos
RIGID BODY DYNAMICS 2
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - RIGID BODY DYNAMICS 2EXERCISE - Multiple Correct26videos
RIGID BODY DYNAMICS 2
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - RIGID BODY DYNAMICS 1EXERCISE - Integer11videos
RIGID BODY DYNAMICS 1
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - SOUND WAVES AND DOPPLER EFFECTEXERCISE - Integer16videos
SOUND WAVES AND DOPPLER EFFECT
Similar Questions
A uniform rod of length l and mass 2m rests on a smooth horizontal table. A point mass m moving horizontally at right angles to the rod with initial velocity v collides with one end of the rod and sticks to it.
Determine the position of the point on the rod which remains stationary immediately after collision.View SolutionConsider the uniForm rod oF mass M=4m and length l pivoted about its centre. A mass m moving with velocity v making angle θ=π4 to the rod's long axis collides with one end oF the rod and sticks to it. The angular oFIGURE the rod-mass system just aFIGUREter the collision is:
View SolutionA uniform rod of length 8 a and mass 6 m lies on a smooth horizontal surface. Two point masses m and 2 m moving in the same plane with speed 2 v and v respectively strike the rod perpendicular at distances a and 2a from the mid point of the rod in the opposite directions and stick to the rod. The angular velocity of the system immediately after the collision is
View SolutionA uniform rod of length L lies on a smooth horizontal table. The rod has a mass M. A particle of mass m moving with speed v strikes the rod perpendicularly at one of the ends of the rod sticks to it after collision.
Find the velocity of the particle with respect to C before the collisionView SolutionA uniform rod of mass m and length L is at rest on a smooth horizontal surface. A ball of mass m, moving with velocity v0, hits the rod perpendicularly at its one end and sticks to it. The angular velocity of rod after collision is
 View Solution
View SolutionA homogenous rod of length l=ηx and mass M is lying on a smooth horizontal floor. A bullet of mass m hits the rod at a distance x from the middle of the rod at a velocity v0 perpendicular to the rod and comes to rest after collision. If the velocity of the farther end of the rod just after the impact is in the opposite direction of v0, then:
View SolutionA uniform rod of mass m and length l rests on a smooth horizontal surface. One of the ends of the rod is struck in a horizontal direction at right angles to the rod. As a result the rod obtains velocity v0. Find the force with which one-half of the rod will act ont he other in the process of motion.
View SolutionA uniform rod of mass m and length / is hinged at upper end. Rod is free to rotate in vertical plane. A bail of mass m moving horizontally with velocity vo collides at lower end of rod perpendicular to it and sticks to it. The minimum velocity of the ball such that combined system just completes the vertical circle will be
View SolutionA uniform rod is resting freely over a smooth horizontal plane. A particle moving horizontally strikes at one end of the rod normally and gets stuck. Then
View SolutionThe rod of length l and mass m lies on a smooth floor. A ball of mass m moving horizotally with a speed u0 striked one end of rod and stick to it. After the collision, the point on the rod that moves translationally ( without any rotation) in the previous direction of motion of the ball.
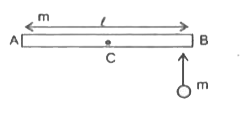
A. Is the centre of C of the rod.
B. Is the left end A of the rod.
C. Lies at a distance l/4 to the left of point C
D. Lies at a distance l/4 to the right of point C.View Solution
