Question

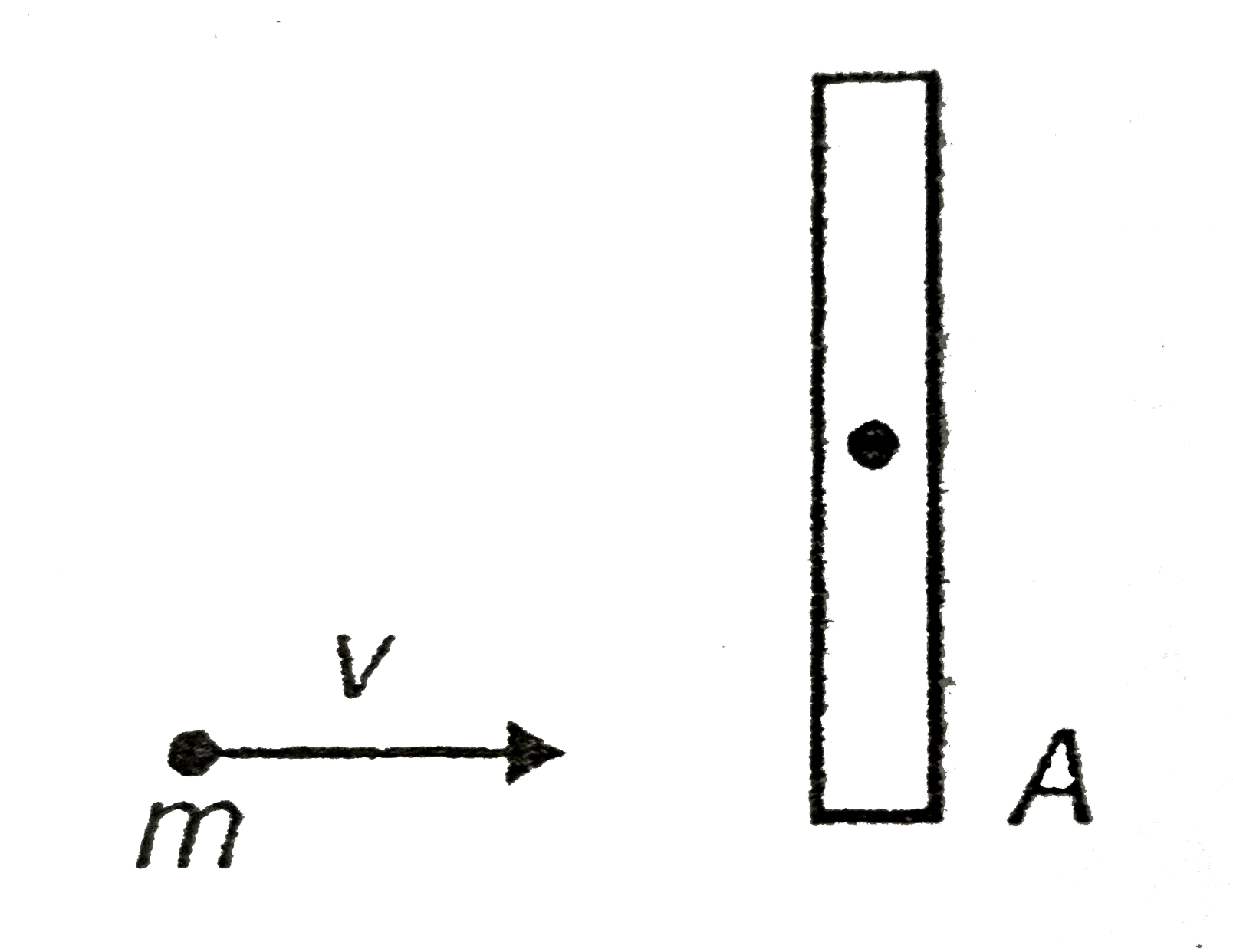
Consider the uniForm rod oF mass M=4m and length l pivoted about its centre. A mass m moving with velocity v making angle $\theta =\frac{\pi }{4}$ to the rod's long axis collides with one end oF the rod and sticks to it. The angular oFIGURE the rod-mass system just aFIGUREter the collision is:

Options

A. `(3sqrt2)/(7)(v)/(l)`
B. `(3)/(7)(v)/(l)`
C. `(3)/(7sqrt2)(v)/(l)`
D. `(4)/(7sqrt2)(v)/(l)`

Answer

`A`

Explanation

To solve the problem, we will use the principles of conservation of angular momentum and the moment of inertia. Here’s a step-by-step solution:

Step 1: Understand the System
We have a uniform rod of mass \( M = 4m \) and length \( l \) pivoted at its center. A mass \( m \) is moving with velocity \( v \) at an angle \( \theta = \frac{\pi}{4} \) with respect to the rod's long axis. The mass collides with one end of the rod and sticks to it.

Step 2: Calculate the Components of Velocity
The velocity \( v \) can be resolved into two components:
- The component along the direction of the rod (x-axis):
\( v_x = v \cos(\theta) = v \cos\left(\frac{\pi}{4}\right) = \frac{v}{\sqrt{2}} \)
- The component perpendicular to the rod (y-axis):
\( v_y = v \sin(\theta) = v \sin\left(\frac{\pi}{4}\right) = \frac{v}{\sqrt{2}} \)

Step 3: Calculate the Angular Momentum Before Collision
The angular momentum \( L_i \) of the mass \( m \) about the pivot point (center of the rod) just before the collision is given by:
\( L_i = m v_x \cdot r \)
where \( r \) is the distance from the pivot to the point of collision (which is \( \frac{l}{2} \)):
\( L_i = m \left(\frac{v}{\sqrt{2}}\right) \left(\frac{l}{2}\right) = \frac{mv l}{2\sqrt{2}} \)

Step 4: Calculate the Moment of Inertia After Collision
After the collision, the system consists of the rod and the mass \( m \). The moment of inertia \( I \) of the rod about its center is:
\( I_{\text{rod}} = \frac{1}{12} M l^2 = \frac{1}{12} (4m) l^2 = \frac{ml^2}{3} \)
The moment of inertia of the mass \( m \) at a distance \( \frac{l}{2} \) from the pivot is:
\( I_{\text{mass}} = m \left(\frac{l}{2}\right)^2 = m \frac{l^2}{4} \)
Thus, the total moment of inertia \( I_f \) after the collision is:
\( I_f = I_{\text{rod}} + I_{\text{mass}} = \frac{ml^2}{3} + m \frac{l^2}{4} \)
Finding a common denominator (12):
\( I_f = \frac{4ml^2}{12} + \frac{3ml^2}{12} = \frac{7ml^2}{12} \)

Step 5: Apply Conservation of Angular Momentum
According to the conservation of angular momentum:
\( L_i = L_f \)
where \( L_f = I_f \cdot \omega \) (angular momentum after the collision):
\( \frac{mv l}{2\sqrt{2}} = \frac{7ml^2}{12} \cdot \omega \)
Cancelling \( m \) from both sides:
\( \frac{v l}{2\sqrt{2}} = \frac{7l^2}{12} \cdot \omega \)

Step 6: Solve for Angular Velocity \( \omega \)
Rearranging the equation to solve for \( \omega \):
\( \omega = \frac{v l}{2\sqrt{2}} \cdot \frac{12}{7l^2} = \frac{6v}{7l\sqrt{2}} \)

Final Answer
Thus, the angular velocity \( \omega \) of the rod-mass system just after the collision is:
\( \omega = \frac{6v}{7l\sqrt{2}} \)