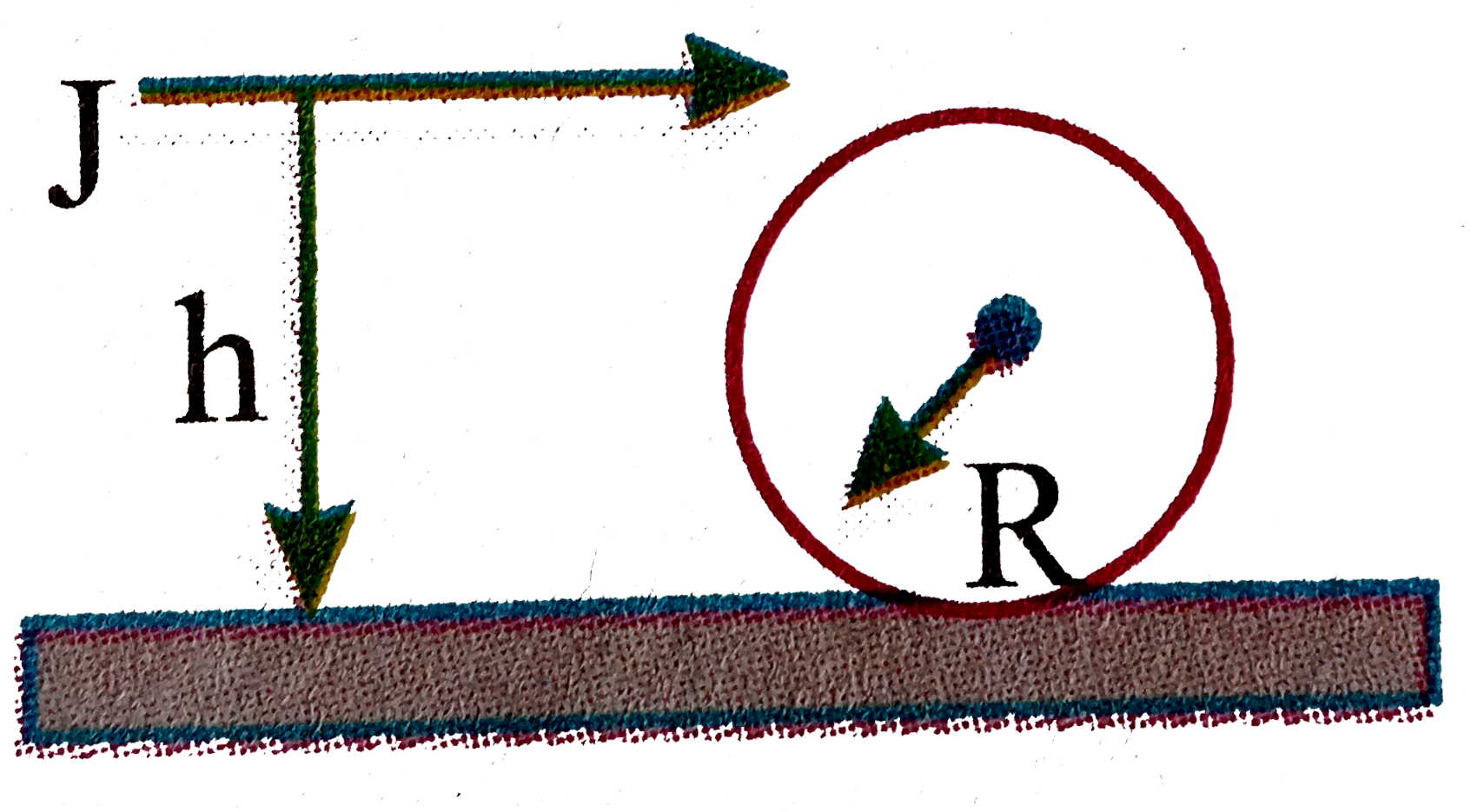
A uniform sphere of mass $m$ and radius $R$ is placed on a rough horizontal surface. The spher is struck horizontally at a height h from the floor. Show that the sphere rolls without slipping with a constant velocity, when $h = 7 R / 5$ .

Video Solution

Text Solution

Verified by Experts

Consider the diagram where a sphere of m and radius ,R, struck horizontally at height h above the floor

the sphere will roll without sipping when $ω = \frac{v}{R}$ , where v is linear velocity and $ω$ is angular velocity of the sphere
Now , angular momentum of sphere , about centre of mass
( [We are applying conservation of angular momentum just momentum just before and after struck ]
$m v (h - R) = I ω = (\frac{2}{5} m R^{2}) (\frac{v}{R})$
$\Rightarrow m v (h - R) = \frac{2}{5} m v R$
$h - R = \frac{2}{5} R \Rightarrow h = \frac{7}{5} R$ Therefore the sphere eill roll without sipping with a constant velocity and hence , no loss of energy ,so $(D) \to (i)$
Torque due to applied force ,F about centre of mass
For $τ = 0, h = R$ ,sphere will have only translational motion . it would lose energy by friction
hence , $(B) \to (i v)$
the sphere will spin clokwise when $τ > 0 \Rightarrow h > R$
therefore , $(C) \to (i i)$
the sphere will spin anti- clockwise when $τ < 0 \Rightarrow h < R, (a) \to (i i i)$

Updated on:21/07/2023