A uniform steel rod of cross- sectional area A and L is suspended so that it hangs vertically. The stress at the middle point of the rod is
12ρgL
14ρgL
ρgL
None of these
The correct Answer is:A
To find the stress at the middle point of a uniform steel rod of cross-sectional area A and length L that is suspended vertically, we can follow these steps:
Step 1: Understand the Concept of Stress
Stress (σ) is defined as the force (F) applied per unit area (A). Mathematically, it is expressed as:
σ=FA
Step 2: Identify the Force at the Middle Point
The force acting on the middle point of the rod is due to the weight of the portion of the rod that is below the middle point. Since the rod is uniform, we can consider the mass of the lower half of the rod.
Step 3: Calculate the Mass of the Lower Half of the Rod
The mass (m) of the lower half of the rod can be calculated using the formula:
m=Volume×Density
The volume of the lower half of the rod is given by:
Volume=A×L2
Thus, the mass can be expressed as:
m=A×L2×ρ
where ρ is the density of the material (steel in this case).
Step 4: Calculate the Force Due to Gravity
The force due to gravity acting on this mass is:
F=m×g=(A×L2×ρ)×g
Step 5: Substitute the Force into the Stress Formula
Now we substitute the expression for force into the stress formula:
σ=FA=(A×L2×ρ×g)A
Step 6: Simplify the Expression
Since the area A cancels out, we have:
σ=L2×ρ×g
Step 7: Final Result
Thus, the stress at the middle point of the rod is:
σ=12ρgL
Conclusion
The correct answer is 12ρgL, which corresponds to option 1.
---
Stress (σ) is defined as the force (F) applied per unit area (A). Mathematically, it is expressed as:
σ=FA
The force acting on the middle point of the rod is due to the weight of the portion of the rod that is below the middle point. Since the rod is uniform, we can consider the mass of the lower half of the rod.
The mass (m) of the lower half of the rod can be calculated using the formula:
m=Volume×Density
The volume of the lower half of the rod is given by:
Volume=A×L2
Thus, the mass can be expressed as:
m=A×L2×ρ
where ρ is the density of the material (steel in this case).
The force due to gravity acting on this mass is:
F=m×g=(A×L2×ρ)×g
Now we substitute the expression for force into the stress formula:
σ=FA=(A×L2×ρ×g)A
Since the area A cancels out, we have:
σ=L2×ρ×g
Thus, the stress at the middle point of the rod is:
σ=12ρgL
The correct answer is 12ρgL, which corresponds to option 1.
Topper's Solved these Questions
- ELASTICITYBOOK - DC PANDEY ENGLISHCHAPTER - ELASTICITYEXERCISE - Level 2 More Than One Correct
8 Videos
- ELASTICITYBOOK - DC PANDEY ENGLISHCHAPTER - ELASTICITYEXERCISE - Level 2 Comprehension Based
6 Videos
- ELASTICITYBOOK - DC PANDEY ENGLISHCHAPTER - ELASTICITYEXERCISE - Level 1 Subjective
8 Videos
- CURRENT ELECTRICITYBOOK - DC PANDEY ENGLISHCHAPTER - CURRENT ELECTRICITYEXERCISE - All Questions
469 Videos
- ELECTROSTATICSBOOK - DC PANDEY ENGLISHCHAPTER - ELECTROSTATICSEXERCISE - Integer
17 Videos
Similar Questions
A steel bar of cross-sectional area A and length l is magnetised to develop an intensity of magnetisation l . The pole strength of the bar is
View SolutionA uniform heavy rod of weight W, cross sectional area a and length L is hanging from fixed support. Young modulus of the material of the rod is Y. Neglect the lateral contraction. Find the elongation of the rod.
View Solution
Knowledge Check
A liquid of density ρ0 is filled in a wide tank to a height h. A solid rod of length L, cross-section A and density ρ is suspended freely in the tank. The lower end of the rod touches the base of the tank and h=L/n (where n gt 1). Then the angle of inclination θ of the rod with the horizontal in equilibrium position is
Asin−1(√ρ0ρ)Bsin−1(n√ρ0ρ)Csin−1(1n√ρ0ρ)Dsin−1(1n√ρρ0)
A rod of uniform cross sectional area 5mm2 weighing5kg and length 1m is suspended vertically from a fixed support the elongation produced in the rod if [Young's modulus of material, Y=2x1011Nm2 and g=10ms−2]
View Solution
A uniform rod AB of length L and mass m is suspended freely at A and hangs vertically at rest when a particle of same mass m is fired horizontally with speed v to strike the rod at its mid point. If the particle is brought to rest after the impact. Then the impulsive reaction at A is horizontal direction is
View SolutionA copper rod of cross sectional area A carries a uniform current I through it. At temperature T if the volume charge density of the rod is ρ how long will the charges take to travel a distance d?
View SolutionA metal rod of length 'L' and cross-sectional area 'A' is heated through 'T'∘C What is the force required to prevent the expansion of the rod lengthwise ?
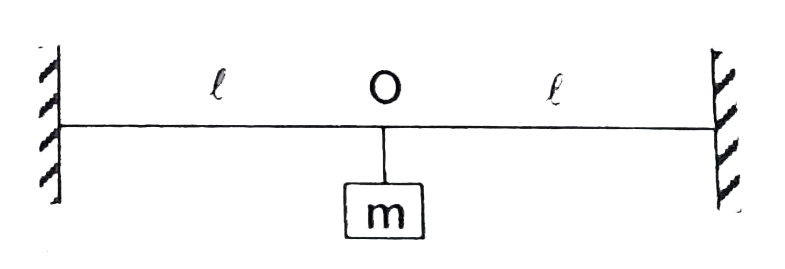
View SolutionA steel wire of negligible mass, length 2I, cross sectional area 'A' and Young's modulus 'Y' is held between two rigid walls. A small body of mass 'm' is suspended from its middle point 'O' as shown. The vertical descent of the middle point 'O' of the wire is
View SolutionA stell rod of length 2l corss sectional area A and mass M is set rotating in a horizotnal plane about an axis passing through its cnetre and perpendicular to its length with constant angular velcoty ω. If Y is the Young's modulus for steel, find the extension in the lenght of the rod. (Assume the rod is uniform.)
View SolutionTwo cylindrical rods of same cross-section area and same length are connected in series to an ideal cell as shown.The resistivity of left rod is ρ and that if right rod is 2ρ Then the variation of potential and electric field at any point P distant x from left end of combined rod system are given by
View Solution
