A water tank is constructed on the top of a building. With what speed will the water come out of a tap 6.0 m below the water level in the tank? Assume steadyy flow and that the pressure above the water level is equal to the atmospheric pressure
To solve the problem of determining the speed at which water will come out of a tap located 6.0 m below the water level in a tank, we can use Torricelli's theorem. This theorem states that the speed v of efflux of a fluid under the influence of gravity through an orifice is given by the equation:
v=√2gh
where:
- g is the acceleration due to gravity (approximately 9.81m/s2, but we can use 10m/s2 for simplicity),
- h is the height of the fluid column above the opening (in this case, 6.0m).
1. Identify the values:
- Height h=6.0m
- Acceleration due to gravity g=10m/s2
2. Substitute the values into the formula:
v=√2gh=√2×10m/s2×6.0m
3. Calculate the product inside the square root:
2×10×6.0=120
4. Take the square root:
v=√120
5. Calculate the square root:
v≈10.95m/s
6. Round the answer:
The speed of the water coming out of the tap is approximately 11m/s.
Final Answer:
The speed at which the water will come out of the tap is approximately 11m/s.
---
- g is the acceleration due to gravity (approximately 9.81m/s2, but we can use 10m/s2 for simplicity),
- h is the height of the fluid column above the opening (in this case, 6.0m).
- Height h=6.0m
- Acceleration due to gravity g=10m/s2
v=√2gh=√2×10m/s2×6.0m
2×10×6.0=120
v=√120
v≈10.95m/s
The speed of the water coming out of the tap is approximately 11m/s.
The speed at which the water will come out of the tap is approximately 11m/s.
Topper's Solved these Questions
- FLUID MECHANICSBOOK - HC VERMACHAPTER - FLUID MECHANICSEXERCISE - Worked Out Examples
11 Videos
- FLUID MECHANICSBOOK - HC VERMACHAPTER - FLUID MECHANICSEXERCISE - Short Answer
16 Videos
- CIRCULAR MOTIONBOOK - HC VERMACHAPTER - CIRCULAR MOTIONEXERCISE - Exercises
30 Videos
- FRICTIONBOOK - HC VERMACHAPTER - FRICTIONEXERCISE - Exercises
31 Videos
Similar Questions
What is the water potential of pure water at atmospheric pressure?
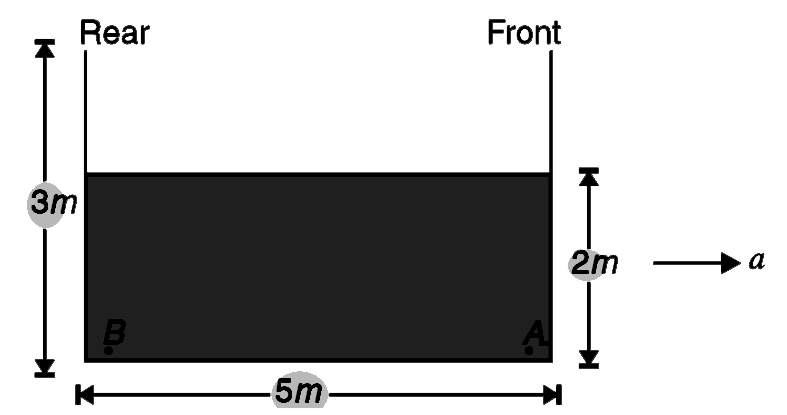
View SolutionAn open rectangular tank 5mxx4mxx3m in dimension is containing water up to a height of 2m the tank is accelerated horizontally along the longer side. Assuming water to be and ideal liquid find-
(a) the maximum acceleration with which the tank can be moved so that water does not fall from the rear side.
(b) the gauge pressure at the bottom of the front and back of the tank (points A and B) if the tank is closed at the top and is then acclerated horizontally at 9m/s2. Assume that the top cover has a small hole at the right side of the tank so taht pressure of air inside the tank is maintained at atmospheric pressure. Gauge pressure at a point is difference in absolute pressure at the point and atmospheric pressure.
View Solution
Knowledge Check
If the pressure at half the depth of a tank is equal to 23 the pressure at the bottom of the tank, then the height of the water column in the tank is
(Atmospheric pressure =105N/m2)A10mB15mC20mD25mA boat floating in a tank is carrying passengers. If the passengers drink water, the water level of the tank
ArisesBfallsCremains unchangedDdepends upon the atmospheric pressureA tank is filled to a height H. The range of water coming out of a hole which is a depth H/4 from the surface of water level is
A2H√3B√3H2C√3HD3H4
A boat flaoting in a water tank is carrying a number of large stones. If the stones are unloaded into the water, what will happen to the water level in the tank
[ hint : Assume the initial level of the tank to be K and the length of the boat immersed in water to be h. Apply the law of floatition. find the volume of water in th tank. repeat the process after a plece of stone is unloaded. the stone will sink to the bottom .
assume the new height to be H' and h' respectively.
make use of the fact that the volume of water remains constant .
H−H=VA(PsPw−1)
where V = volume of stone , A = area of cross - section of tank , Ps = density of stone and Pw = desnsity of water .]
View SolutionA water tank is 20 m deep . If the water barometer reads 10 m at the surface , then what is the pressure at the bottom of the tank in atmosphere ?
View SolutionWater flows out of a small hole in the wall of a large tank near its bottom. What is the speed of efflux of water when height of water level in the tank is 5.0 m ?
View SolutionA rectangular tank is 225 m by 162 m at the base. With what speed must the water flow into it through an aperture 60 cm by 45 cm that the level may be raised 20 cm in 5 hours.
View SolutionTo fill a rectangular tank of area 700m2,140m3 of water is required . What will be the height of the water level in the tank ?
View Solution
