A wide hose pipe is held horizontally by fireman.It delivers water through a nozale at one lirte per second. On increasing the pressure, this increass to two litres per second.The fireman has now to
push forward twice as hard
pulsh forward four times as hard
push backward four times as hard
push backward twice as hard
The correct Answer is:B
To solve the problem, we need to analyze the situation involving the flow of water through a hose pipe and how the force exerted by the fireman changes when the flow rate of water increases.
1. Understanding the Initial Flow Rate:
- The hose pipe initially delivers water at a rate of 1 liter per second. This can be denoted as Q1=1L/s.
2. Understanding the Increased Flow Rate:
- After increasing the pressure, the flow rate increases to 2 liters per second. This can be denoted as Q2=2L/s.
3. Relating Flow Rate to Force:
- The force exerted by the fireman can be related to the flow rate of water. The force F required to maintain the flow can be expressed as:
F∝Q2
- This means that the force is proportional to the square of the flow rate.
4. Calculating the Ratio of Forces:
- Using the relationship F1/F2=Q21/Q22:
F1/F2=(12)/(22)=1/4
- Therefore, F2=4×F1.
5. Conclusion:
- Since the force exerted by the fireman must increase to maintain the increased flow rate, the fireman must push forward 4 times as hard to counteract the increased reaction force from the water flow.
Final Answer:
The fireman has to push forward four times as hard.
- The hose pipe initially delivers water at a rate of 1 liter per second. This can be denoted as Q1=1L/s.
- After increasing the pressure, the flow rate increases to 2 liters per second. This can be denoted as Q2=2L/s.
- The force exerted by the fireman can be related to the flow rate of water. The force F required to maintain the flow can be expressed as:
F∝Q2
- This means that the force is proportional to the square of the flow rate.
- Using the relationship F1/F2=Q21/Q22:
F1/F2=(12)/(22)=1/4
- Therefore, F2=4×F1.
- Since the force exerted by the fireman must increase to maintain the increased flow rate, the fireman must push forward 4 times as hard to counteract the increased reaction force from the water flow.
The fireman has to push forward four times as hard.
Topper's Solved these Questions
- SOLVD PAPERS 2017 NEET, AIIMS & JIPMERBOOK - DC PANDEY ENGLISHCHAPTER - SOLVD PAPERS 2017 NEET, AIIMS & JIPMEREXERCISE - Solved paper 2018(NEET)
22 Videos
- SOLVD PAPERS 2017 NEET, AIIMS & JIPMERBOOK - DC PANDEY ENGLISHCHAPTER - SOLVD PAPERS 2017 NEET, AIIMS & JIPMEREXERCISE - Solved paper 2018(AIIMS)
28 Videos
- SOLVD PAPERS 2017 NEET, AIIMS & JIPMERBOOK - DC PANDEY ENGLISHCHAPTER - SOLVD PAPERS 2017 NEET, AIIMS & JIPMEREXERCISE - Solved Papers 2017(AIIMS)
26 Videos
- SIMPLE HARMONIC MOTIONBOOK - DC PANDEY ENGLISHCHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - Integer type questions
14 Videos
- SOUND WAVESBOOK - DC PANDEY ENGLISHCHAPTER - SOUND WAVESEXERCISE - Exercise 19.7
4 Videos
Similar Questions
A fat hose pipe is held horizontally by a fireman. It delivers water through a constricting nozzle at 1 litre/sec. If by increasing the pressure, the water is delivered at 2 litre/sec, the fireman now has to
View SolutionA transverse sinusoidal wave is generted at one end of long, horizontal string by a bar that moves up and down through a distance of 1.00cm. The motion is continuous and is repreated regularly 120 times per second. The string has linear density 90gm/m and is kept under a tension of 900N. Find :
The maximum value of the transverse component of the tension (in newton)
View SolutionA transverse sinusoidal wave is generted at one end of long, horizontal string by a bar that moves up and down through a distance of 1.00cm. The motion is continuous and is repreated regularly 120 times per second. The string has linear density 90gm/m and is kept under a tension of 900N. Find :
What is the transverse displacement y(in cm) when the minimum power transfer occurs
[Leave the answer in terms of π wherever it occurs]
View SolutionFrom a tap of inner radius 0.75cm, water flows at the rate of 7m per second. Find the volume in litres of water delivered by the pipe in one hour.
View SolutionFrom a tap of inner radius 0.75cm, water flows at the rate of 7m per second. Find the volume in litres of water delivered by the pipe in one hour.
View SolutionTwo wires are in unison. If the tension in one of the wires is increased by 2%, 5 beats are produced per second. The initial frequency of each wire is
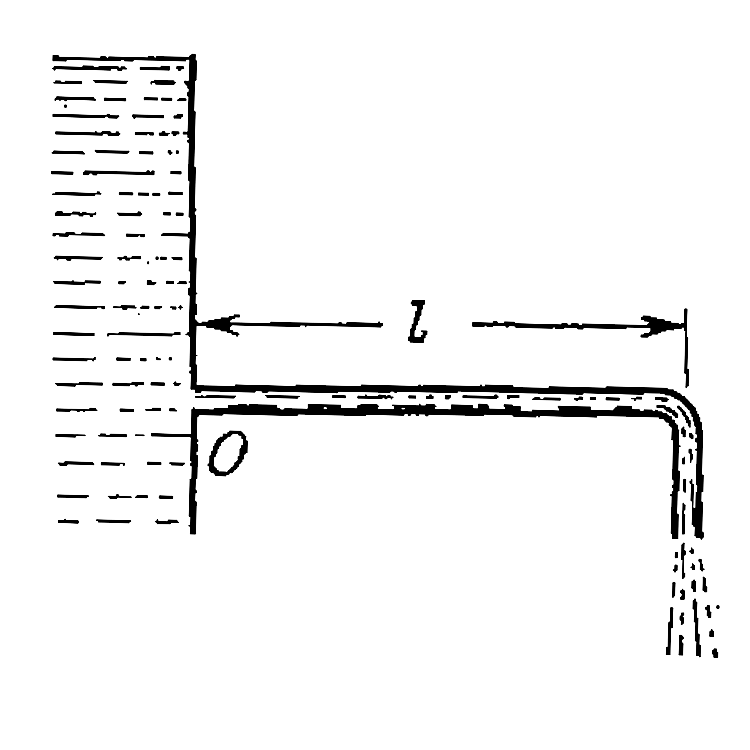
View SolutionWater flows out of a big tank along a tube bent at right angles, the inside radius of the tube is equal to r (figure). The length of the horizontal section of the tube is equal to l. The water flow rate is Q litres per second. Find the moment of reaction forces of flowing water, acting on the tube's walls, relative to the point O.
View SolutionA transverse sinusoidal wave is generated at one end of a long horizontal string by a bar that moves with an amplitude of 1.12 cm . The motion of the bar is continuous and is repeated regularly 120 times per second. The string has linear density of 117g/m. The other end of the string is attached to a mass 4.68 kg. The string passes over a smooth pulley and the mass attached to the other end of the string hangs freely under gravity.
The maximum magnitude of the transverse speed is
View SolutionA transverse sinusoidal wave is generated at one end of a long horizontal string by a bar that moves with an amplitude of 1.12 cm . The motion of the bar is continuous and is repeated regularly 120 times per second. The string has linear density of 117g/m. The other end of the string is attached to a mass 4.68 kg. The string passes over a smooth pulley and the mass attached to the other end of the string hangs freely under gravity.
The maximum magnitude of the transverse component of tension in the string is
View SolutionThe human ciculatory system can be thought of as a closed system of interconnecting pipes through which fluid is continuously circulated by two pumps the two pumps the right and left verticles of the heart, work as simple two-stroke force pumps. The muscles of the heart regulate the force by contracting and relaxing. the contraction (systole) lasts about 0.2s and a complete systole/diastole (contraction/relaxation) cycle lasts about 0.8s. For flood pressures and speeds in the normal range. the volume flow rate of blood through a blood vessel is directly proportional tot he pressure difference over a length of the vessel and to the fourth power of the radius of the vessel. The total mechanical energy per unit volume of blood just as it leaves the heart is E/V=ρgh+P+ρv2
Q. Why is diastolic blood pressure muchh lower than systolic pressure?
A. because the heart exerts more force on the blood during diastole
B. Because the heart exerts no force on the blood during diastole
C. Because the radii of the blood vessels increase during diastole while the force exerted by the heart on the blood remains the same.
D. Because the radii of the blood vessels increase during diastole while the force exerted by the heart on the blood remains the same.
View Solution
