A wire loop enclosing a semicircle of radius R islocated on the boundary of a uniform magnetic field B. At the moment t=0, the loop is set into rotation with constant angular acceleration α about an axis O. The clockwise emf direction is taken to be positive.
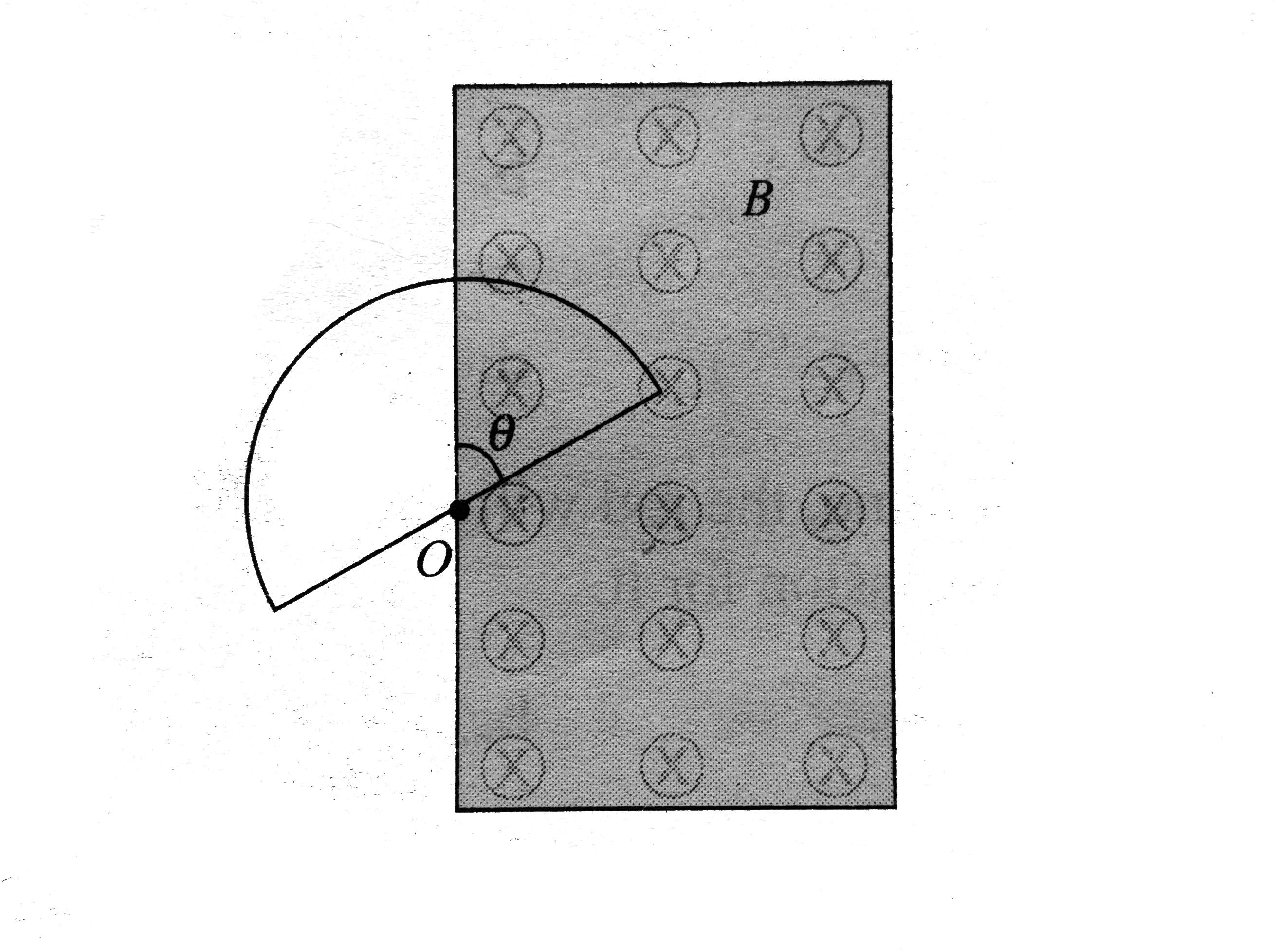
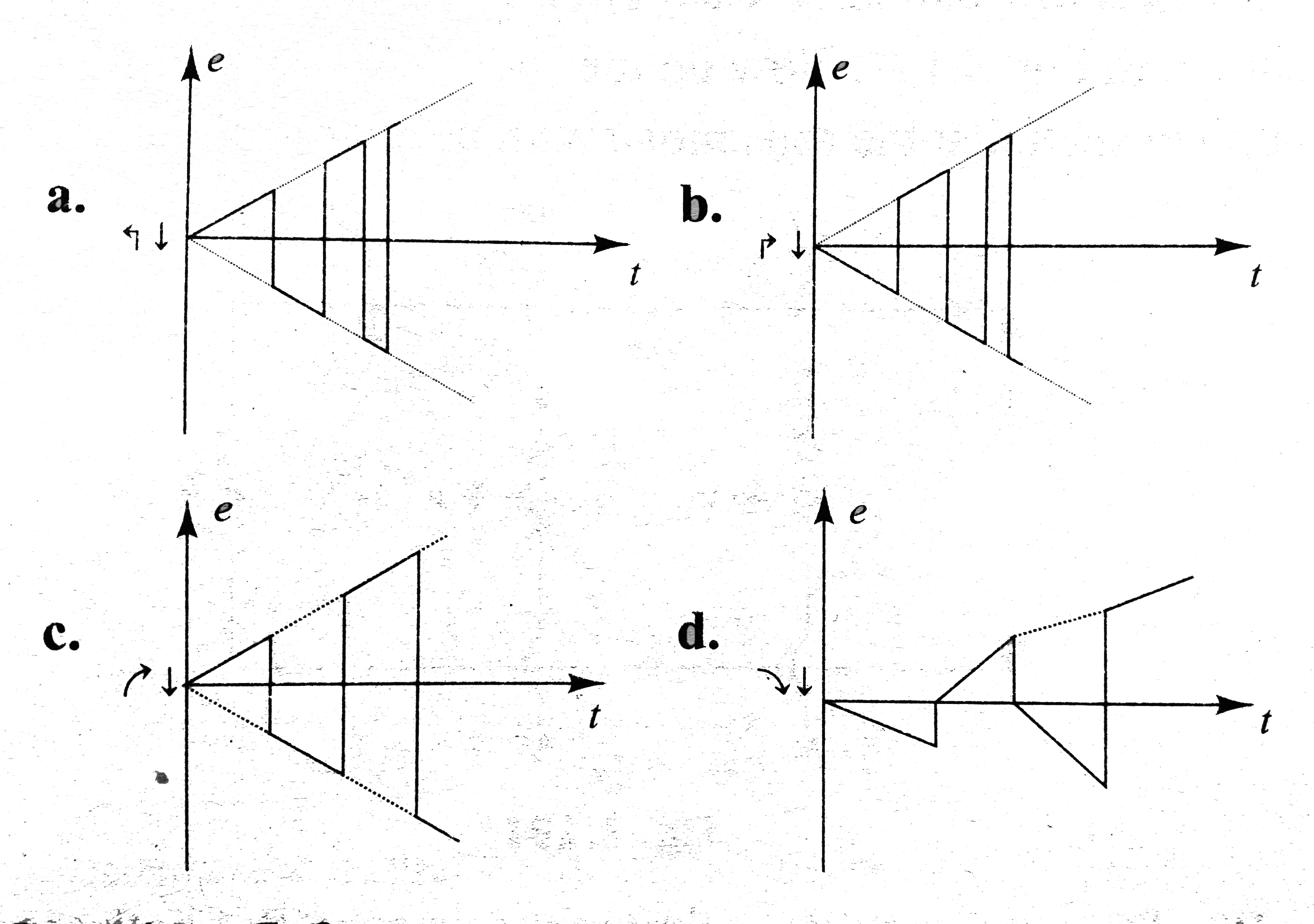
The variation of emf as a function of time is
(a) 
(b) 
( c) 
(d) 
The correct Answer is:B
(b)emf is induced in the loop because area inside the magnetic field is continually changing.
From θ=0→π, 2π→3π, 4π→5π, the loop beings to enter the magnetic field. Thus the magnetic field passing through the loop is increasing.Hemce, current in the loop is anticlockwise, and for θ=π→2π, 3π→4π, 5pi to 6pi,etc.mag≠ticfieldpassingthroughthel∞pisdecreasing.Hencecurrent∈thel∞pisclockwise.Letatanytime,∠rotatedistheta,thentheta = (1)/(2) alphat^(2)<br>Area∈sicemag≠ticfield.A = (1)/(2)R^(2)theta = (1)/(2)R^(2)((1)/(2) alphat^(2))=14R2αt2
Flux in the loop: ϕ=BA=B4R2αt2
emf: e=−dϕdt=−B2R2αtrarre∝t
Time taken to complete first half circle: t1=√2πα
When within magnetic field:
A=12R2(2π−θ)=πR2−R2θ2
emf e=−dϕdt=B2R2αt
e=−BR22=αtrarre∝t
Time taken to complete second half revolution
t2=√4πα=√2πα
We see that t2<t1
We can write induced emf as e=(−1)n[12BR2αt]
Where n=1,2,3,...... is the number of half revolution completed by loop Smaller time will be taken to complete the second half revolution as compared to teh previous half revolution.


Topper's Solved these Questions
- ELECTROMAGNETIC INDUCTIONBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - Exercises Integer
9 Videos
- ELECTROMAGNETIC INDUCTIONBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - Archives Fill In The Blanks
3 Videos
- ELECTROMAGNETIC INDUCTIONBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - Exercises Asserton - Reasoning
8 Videos
- ELECTRICAL MEASURING INSTRUMENTSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - ELECTRICAL MEASURING INSTRUMENTSEXERCISE - M.C.Q
2 Videos
- ELECTRON,PHONTS,PHOTOELECTRIC EFFECT & X-RAYSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - ELECTRON,PHONTS,PHOTOELECTRIC EFFECT & X-RAYSEXERCISE - dpp 3.3
15 Videos
Similar Questions
A wire loop enclosing a semicircle of radius R islocated on the boundary of a uniform magnetic field B. At the moment t=0, the loop is set into rotation with constant angular acceleration α about an axis O. The clockwise emf direction is taken to be positive.
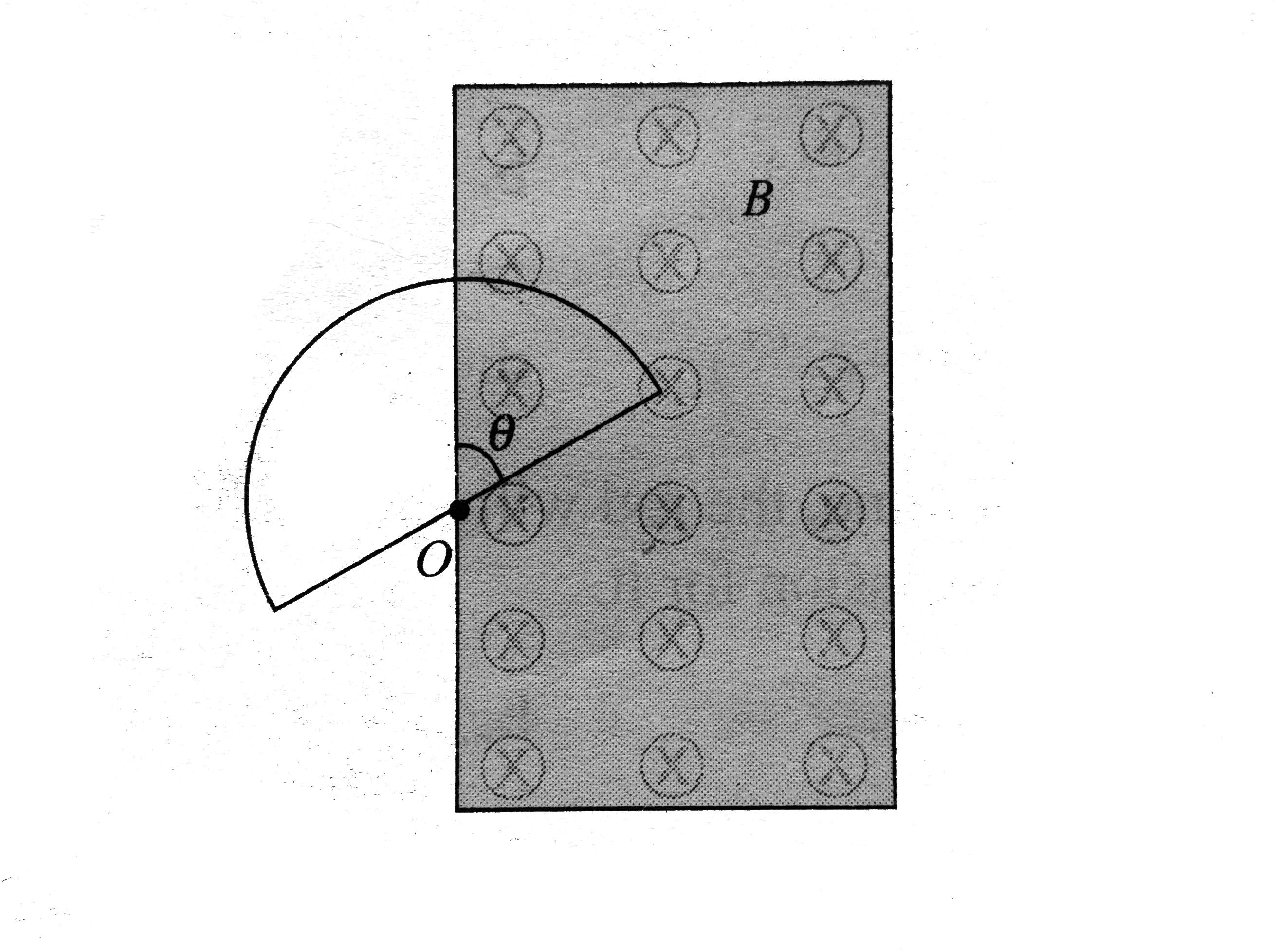
The variation of emf as function of time is
View SolutionA wire loop enclosing as semicircle of radius R is located on the boudary of uniform magnetic field B. At the moment t=0, the loop is set into rotation with a costant angular acceleration α about an axis O coinciding with a line of vector B on the boundary. Find the emf induced in the loop as a function of time. Draw the approximate plot of this function.The arrow in the figure shows the emf direction taken to be positive.
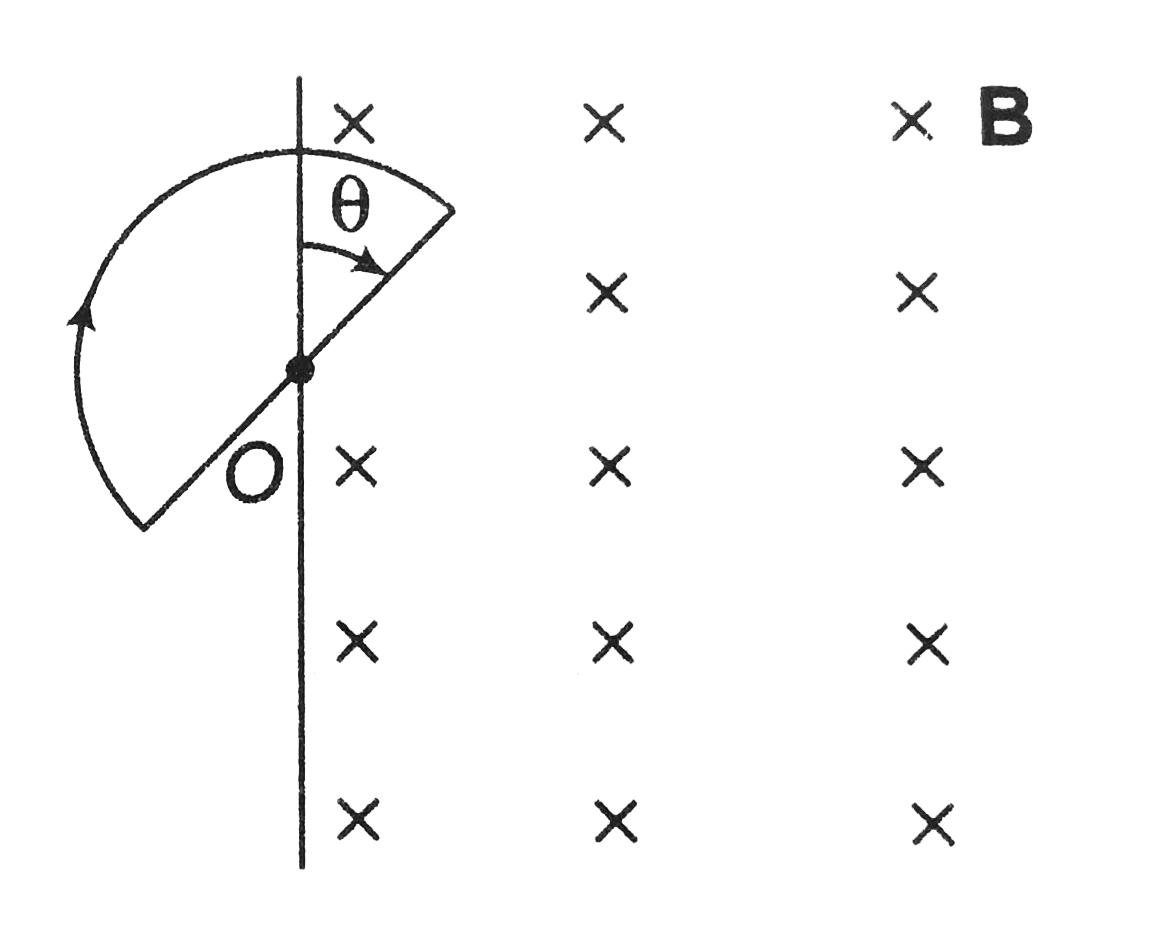
View SolutionA wire loop enclosing a semi-circle of radius a=2cm is located on the boundary of a uniform magnetic field of induction B=1T (figure).At the moment t=0 the loop is set into rotation with a constant angular acceleration β=2rad/sec2 about an axis O coinciding with a line of vector B on the boundary.The emf induced in the loop as a function of time t is [x×10−4(−1)n×t]V, where n=1,2,... is the number of the half-revolution taht the loop performs at the given moment t.Find the value of x.(The arrow in the figure shows the emf direction taken to be positive, at t=0 loop was completely outside)
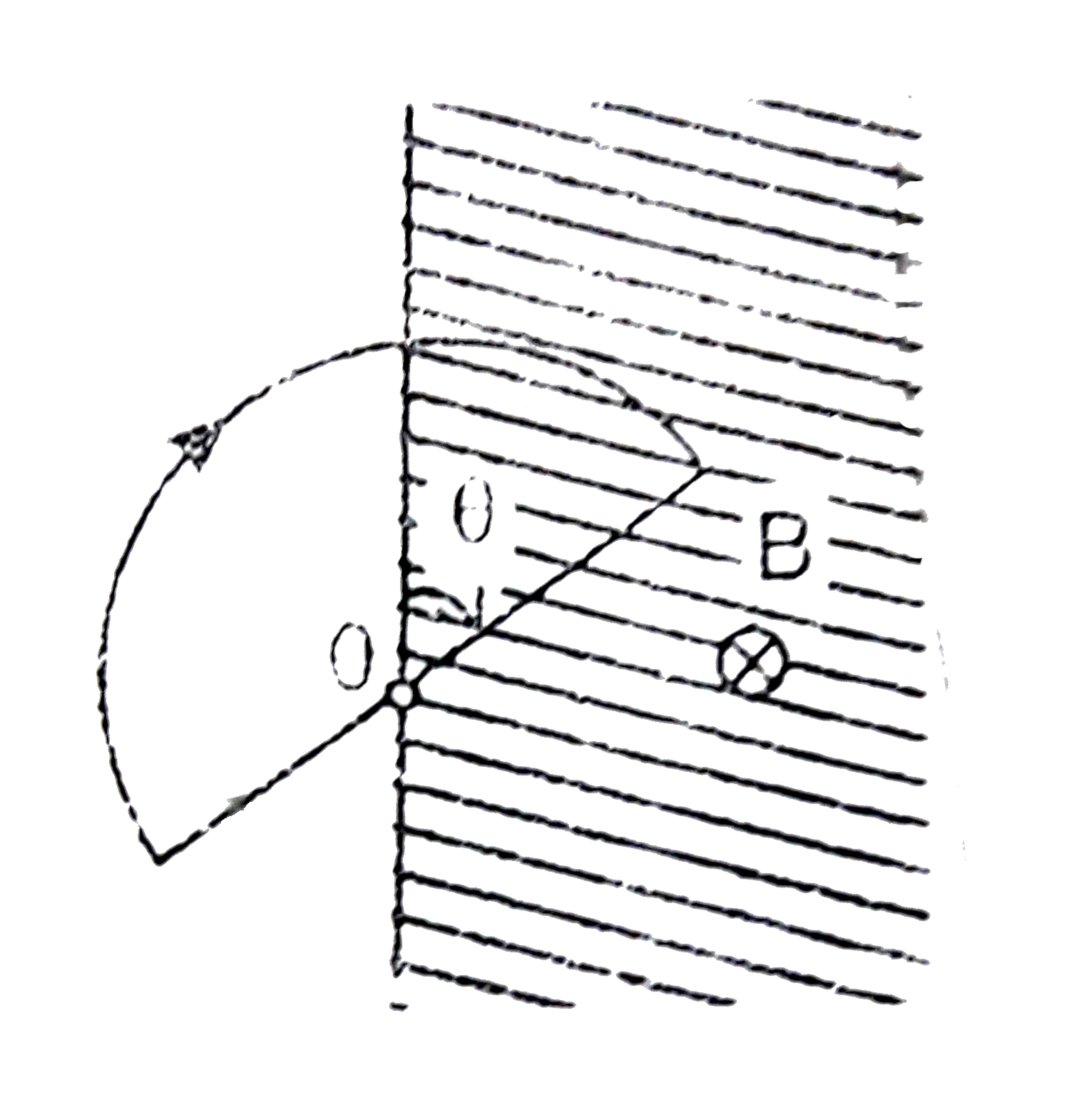
View SolutionFor the L shaped conductor in a uniform magnetic field B shown in figure the emf across its ends when it rotates with angular velocity ω about an axis through one its ends O and normal to its plane will be
View SolutionA semi-circular loop of radius R is placed in a uniform magnetic field as shown. It is pulled with a constant velocity. The induced emf in the loop is
View SolutionA wire loop confined in a plane is rotated in its open plane with some angular velocity. A uniform magnetic field exists in the region. Find the emf induced in the loop.
View SolutionFind the ratio of magnetic dipole moment and magnetic field at the centre of a disc. Radius of disc is R and it is rotating at constant angular speed o about its axis. The disc is insulating and uniformly charged
View SolutionA circular wire loop of radius r is placed in a region of uniform magnetic field B such that the plane of the loop makes an angle θ with the direction of B. In which of the following conditions, will no emf be induced in the loop?
View SolutionA uniform circular loop of radius a and resistance R palced perpendicular to a uniform magnetic field B. One half of the loop is rotated about the diameter with angular velocity ω as shown in Fig. Then, the current in the loop is

View SolutionA uniform circular loop of radius a and resistance R palced perpendicular to a uniform magnetic field B. One half of the loop is rotated about the diameter with angular velocity ω as shown in Fig. Then, the current in the loop is

View Solution
