An electron accelerated by a potnetial difference V=1.0kV moves in a unifrom magentic field at angle α=30∘ to the vector B whose modulus is B=29mT. Find the pithch of the helical trajectroy of the electron.
T=eV=12mv2
(The given potential difference is not large enough to cause significant deviations from the nonletivistic formula).
Thus,. v=√2eVm
So, v∣∣=√2eVmcosα,v⊥=√2eVmsinα
Now, mv2⊥r=Bev⊥ or, r=mv⊥Be,
and T=2πv⊥=2πmBe
Pitch p=v∣∣T=2πmBe√2eVmcosα=2π√2mVeB2cosα
Topper's Solved these Questions
- BOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - ELECTRODYNAMICSEXERCISE - Electromagnetic Induction84videos
ELECTRODYNAMICS
- BOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - ELECTRICITY AND MAGNETISMEXERCISE - All Questions6videos
ELECTRICITY AND MAGNETISM
- BOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - ELECTROMAGNETISMEXERCISE - All Questions24videos
ELECTROMAGNETISM
Similar Questions
An electron accelerated by a potential difference V=1.0kV moves in a uniform magnetic field at an angle α=30∘ to the vector B whose modulus is B=29mT. Find the pitch of the helical trajectory of the electron.
View SolutionAn electron accelerated by a potential difference V=3 volt first enters into a uniform electric field of a parallel plate capacitor whose plates extend over a length l=6cm in the direction of initial velocity. The electric field is normal to the direction of initial velocity and its strength varies with time as E=αt, where α=3600Vm−1s−1. Then the electron enters into a uniform magnetic field of induction B=π×10−9T. Direction of magnetic field is same as that of the electric field. Calculate pitch of helical path traced by the electron in the magnetic field. (Mass of electron, m=9×10−31kg)
View Solution
Knowledge Check
- Question 1 - Select One
An electron of mass 'm' is accelerated through a potential difference of V and then it enters a magnetic field of inductionB. Normal to the lines of force. Then the radius of the circular path is
A√2eVmB√2VmeB2C√2VmeBD√2Vme2B - Question 2 - Select One
Electrons accelerated by a potential differnece V enter a uniform magnetic field of flux density B at right angles to the field. They describe a circular path of radius 'r'. If now 'V' is doubled and B is also doubled, the radius of the new circular path is
A4rB2rC2√2rDr/√2
An electron accelerated in an electric field of 20 kV enters a uniform magnetic field with induction 0.1 T. Its velocity vector makes an angle of 75∘ with the magnetic field vector. Find the shape of the path.
View SolutionA proton accelarted by a potential differnce V=500kV fieles through a unifrom transverse magnetic filed the induction B=0.54T. The field occupies a region of space d=10cm in thickness (Fig). Find the angle α through which the proton deviates from the initial direction of its motion.
 View Solution
View SolutionElectrons which have been accelerated by a potential difference of 1000 V enter co-terminus electric and magnetic fields of strengths 20V/cm and 1 G respectively as in the Thomson's experiment. Estimate the e/m and the speed fo the electrons if they move undeviated through this system.
View SolutionAn electron emitted by heated cathode and accelerated through a potential difference of 2.0 kV, enters a region with uniform magnetic field of 0.15 T. Determine the trajectory of the electron if the field (a) is transverse to its initial velocity , (b) makes an angle of 30∘ with the initial velocity.
View SolutionAn electron emmited by a heated cathode and accelerated through a potential difference of 2⋅0kV enters a region with a uniform magnetic field of 0⋅15T. Determine the trajectory of the electron if the field (a) is transverse to its initial velocity (b) makes an angle of 30∘ with the initial velocity.
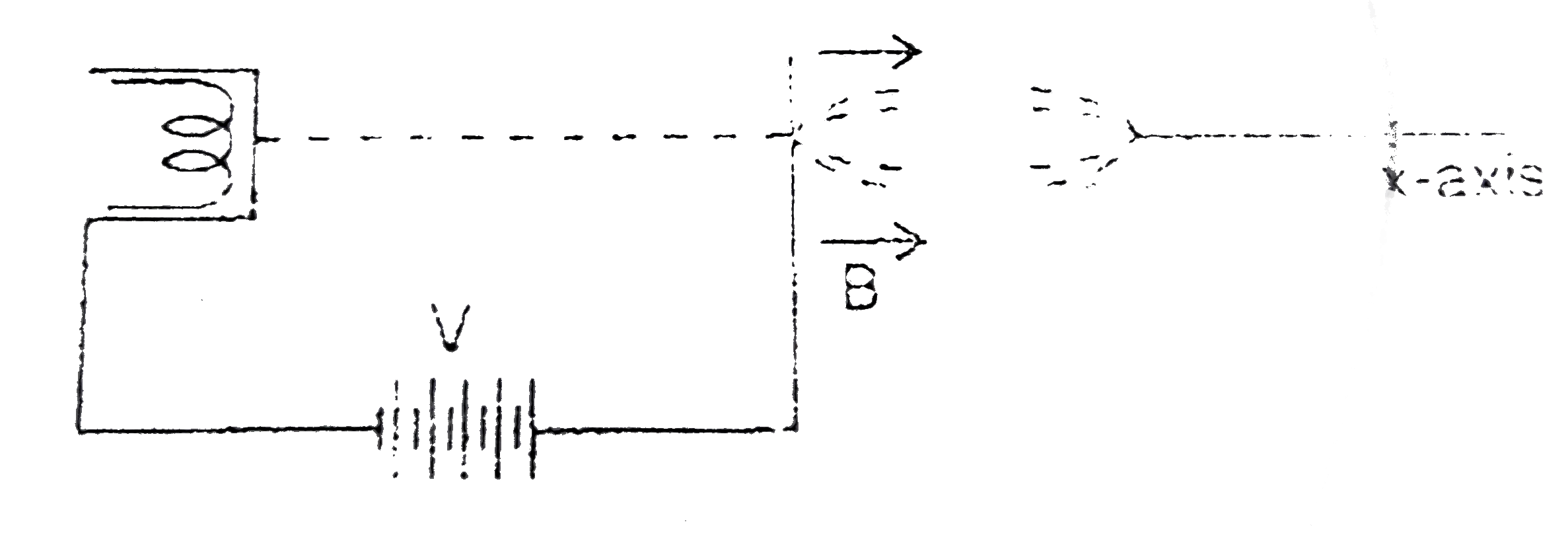
View SolutionElectrons emitted with negligible speed from an electron gun are accelerated through a potential difference V along X-axis.These electrons emerges from a narrow hole into a unifrom magnetic field B directed along the axis.However, some of the electrons emerging from the hole make slightly divergent angles as shown in figure.These paraxial electrons meet for second time on the X-axis at a distance √Nπ2mVeB2.Then find value of N.(Neglect interaction between electrons)
 View Solution
View Solution

