An object of mass m, initially at rest under the action of a constant force F attains a velocity v in time t. Then, the average power supplied to mass is
mv22t
Fv2
Both are correct
Both are wrong
The correct Answer is:C
To solve the problem of finding the average power supplied to an object of mass m that is initially at rest and attains a velocity v under the action of a constant force F in time t, we can follow these steps:
1. Understand the Concept of Average Power:
Average power Pavg is defined as the work done W divided by the time t:
Pavg=Wt
2. Determine the Work Done:
The work done on the object is equal to the change in kinetic energy. Since the object starts from rest, the initial kinetic energy is 0. The final kinetic energy when the object reaches velocity v is given by:
KE=12mv2
Therefore, the work done W is:
W=12mv2
3. Substitute Work Done into the Average Power Formula:
Now, substituting the expression for work done into the average power formula:
Pavg=Wt=12mv2t
This simplifies to:
Pavg=mv22t
4. Relate Force to Acceleration:
From Newton's second law, we know that the force F acting on the object is related to its mass and acceleration a by:
F=ma
Since the object accelerates from rest to velocity v in time t, we can express acceleration as:
a=vt
5. Substitute Acceleration into the Force Equation:
Substituting a into the force equation gives:
F=m(vt)
6. Express Average Power in Terms of Force:
Now, we can express the average power in terms of the force:
Pavg=mv22t=Fv2
Final Result:
Thus, the average power supplied to the mass is:
Pavg=mv22torPavg=Fv2
Average power Pavg is defined as the work done W divided by the time t:
Pavg=Wt
The work done on the object is equal to the change in kinetic energy. Since the object starts from rest, the initial kinetic energy is 0. The final kinetic energy when the object reaches velocity v is given by:
KE=12mv2
Therefore, the work done W is:
W=12mv2
Now, substituting the expression for work done into the average power formula:
Pavg=Wt=12mv2t
This simplifies to:
Pavg=mv22t
From Newton's second law, we know that the force F acting on the object is related to its mass and acceleration a by:
F=ma
Since the object accelerates from rest to velocity v in time t, we can express acceleration as:
a=vt
Substituting a into the force equation gives:
F=m(vt)
Now, we can express the average power in terms of the force:
Pavg=mv22t=Fv2
Thus, the average power supplied to the mass is:
Pavg=mv22torPavg=Fv2
Topper's Solved these Questions
- WORK, ENERGY AND POWERBOOK - DC PANDEY ENGLISHCHAPTER - WORK, ENERGY AND POWEREXERCISE - MEDICAL ENTRANCE SPECIAL QUESTIONS
16 Videos
- WORK, ENERGY AND POWERBOOK - DC PANDEY ENGLISHCHAPTER - WORK, ENERGY AND POWEREXERCISE - MATCH THE COLUMNS
7 Videos
- WORK, ENERGY AND POWERBOOK - DC PANDEY ENGLISHCHAPTER - WORK, ENERGY AND POWEREXERCISE - CHECK POINT 6.3
10 Videos
- WORK, ENERGY & POWERBOOK - DC PANDEY ENGLISHCHAPTER - WORK, ENERGY & POWEREXERCISE - Level 2 Comprehension Based
2 Videos
- WORK, POWER AND ENERGYBOOK - DC PANDEY ENGLISHCHAPTER - WORK, POWER AND ENERGYEXERCISE - E Integer Type Questions
11 Videos
Similar Questions
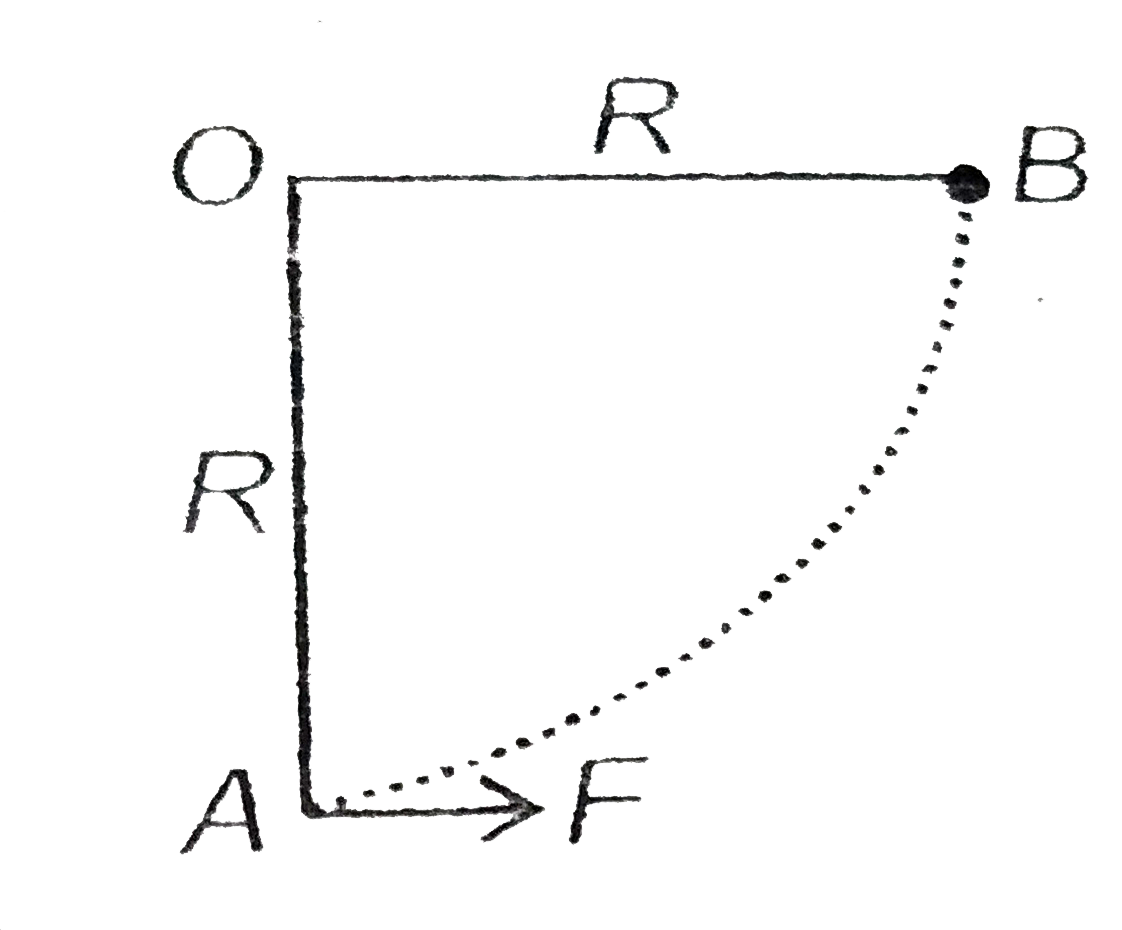
A block of mass m is taken from A to B under the action of a constant force F. Work done by this force is
View SolutionUnder the action of a constant force, A particle is experiencing a constant acceleration. The power is
View SolutionA particle of mass m moves from rest under the action of a constant force F which acts for two seconds. The maximum power attained is
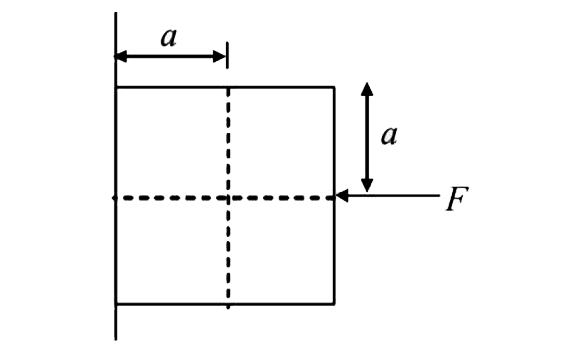
View SolutionA block of mass m is at rest under the action of force F against a wall as shown in figure. Which of the following statement is incorrect?
View SolutionA car of mass M is accelerating on a level smooth road under the action of a single force F acting along the direction of motion. The power delivered to the car is constant and equal to p. If the velocity of the car at an instant is v, then after travelling a distance of 7Mv33p the velocity become kv where k is__________.
View SolutionAn automobile of mass m accelerates from rest. If the engine supplies a constant power P, the velocity at time t is given by -
View SolutionA particle of mass 2kg moves in the xy plane under the action of a constant force →F where →F=ˆi−ˆj. Initially the velocity of the particle is 2ˆj. The velocity of the particle at time t is
View SolutionAn object of mass 2 kg at rest at origin starts moving under the action of a force
→F=(3t2ˆi+4tˆj)N The velocity of the object at t = 2 s will be -
View SolutionAn object of mass 8 kg starts to move from rest under the action of a variable force F=3xN where x is the distance (in m) covered by the object. If initially, the position of the object is x = 2 m, then find its speed (in m s−1) when it crosses x = 10 m.
View SolutionA body moves under the action of a constant force along a straight line. The instantaneous power developed by this force with time t is correctly represented by.
View Solution
