Assertion : Two spherical shells have masses $m_{1} and m_{2}$ . Their radii are $r_{1} and r_{2}$ . Let $r$ be the distance of a point from centre. Then gravitational field strength and gravitational potential both are equal to zero for $O < r < r_{1}$

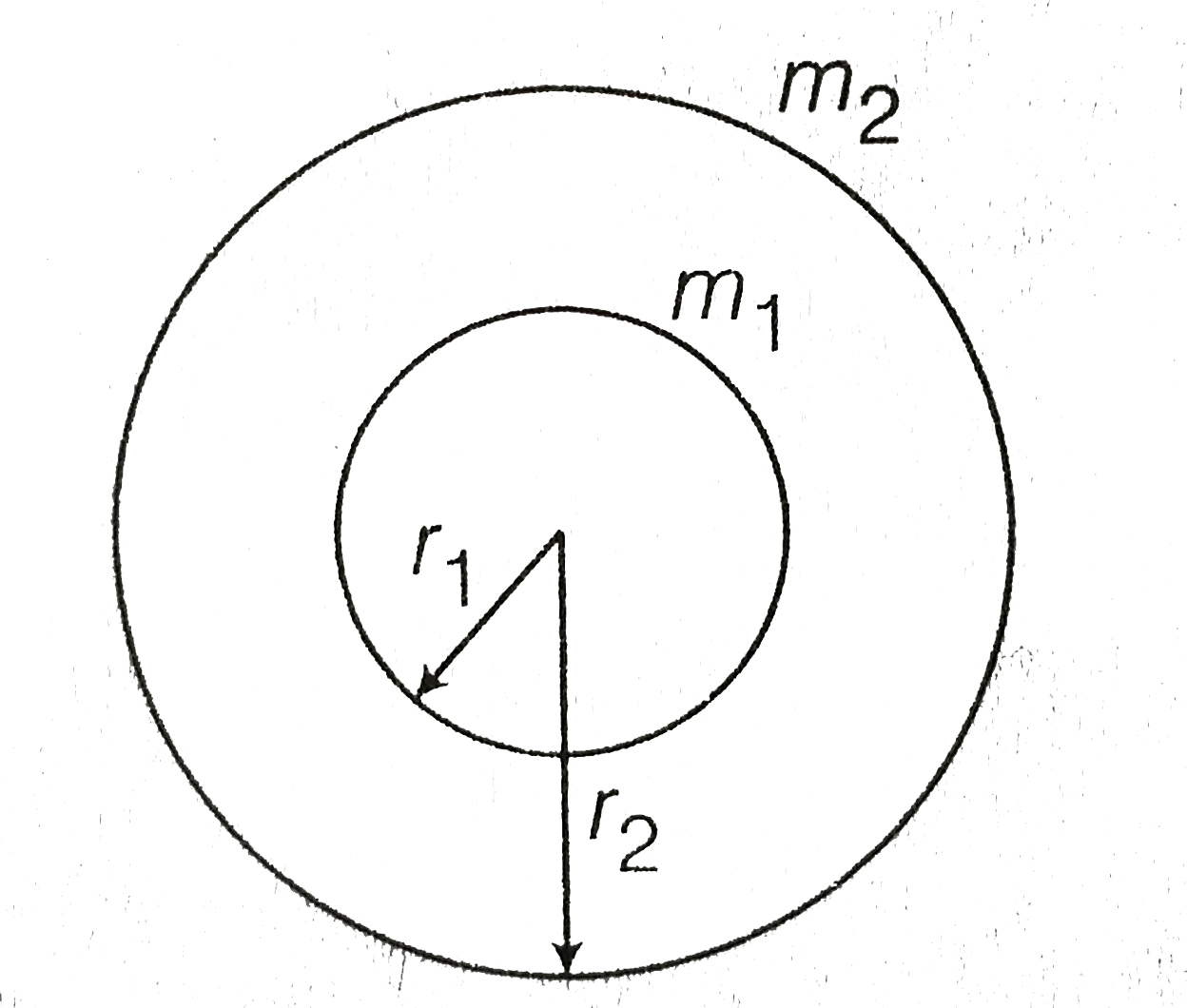
Reason : In the region $r_{1} < r < r_{2}$ , gravitational field strength due to $m_{2}$ is zero. But gravitational potential due to $m_{2}$ is constant (but non-zero).

A

If both Assertin and Reason are correct and Reason is the correct explanation of Assertion

B

If both Assertion and Reason are correct but Reason is not the correct explanation of Assertion

C

If Assertion is true but Reason is false

D

If Assertion is false but Reason is true

Video Solution

Text Solution

Verified by Experts

The correct Answer is:D

In the region $0 < r < r_{1}$
F = 0 but $v$ = constant.

|

Updated on:21/07/2023

Class 11 PHYSICS GRAVITATION

Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One
Two concentric shells of masses $M_{1}$ and $M_{2}$ are having radii $r_{1}$ and $r_{2}$ . Which of the following is the correct expression for the gravitational field on a mass $m$ ?

A $F = \frac{G (M_{1} + M_{2})}{r^{2}}$ , for $r < r_{1}$
B $F = \frac{G (M_{1} + M_{2})}{(r^{2})}$ , for $r < r_{2}$
C $F = \frac{G M_{2}}{r^{2}}$ , for $r_{1} < r < r_{2}$
D $F = G \frac{M_{1}}{r^{2}}$ , for $r_{1} < r < r_{2}$
Question 2 - Select One
Two bodies of masses $M_{1}$ and $M_{2}$ , are placed at a distance $R$ apart. Then at the position where the gravitational field due to them is zero, the gravitational potential is
A $- G \frac{{\sqrt{M}}_{1}}{R}$
B $- G \frac{{\sqrt{M}}_{2}}{R}$
C $- {({\sqrt{M}}_{1} + {\sqrt{M}}_{2})}_{1}^{2} \frac{G}{R}$
D $- {({\sqrt{M}}_{1} - {\sqrt{M}}_{2})}_{1}^{2} \frac{G}{R}$
Question 3 - Select One
Two concentric shells have masses $M$ and $m$ and their radii are $R$ and $r$ , respectively, where $R > r$ . What is the gravitational potential at their common centre?
A $- \frac{G M}{R}$
B $- \frac{G M}{r}$
C $- G [\frac{M}{R} - \frac{m}{r}]$
D $- G [\frac{M}{R} + \frac{m}{r}]$

DC PANDEY-GRAVITATION-(B) Chapter Exercises

Home
Ask Doubt
Profile