At the instant θ=θ1 in the fig the boy's center of mass G has speed vG, Determine the rate of increase in his speed and the tension in each of the two supporting cords of the swing at this instant. The boy has a weight W. Neglect his size and the mass of the seat and cords. Given m=20kg θI=53∘,vG=3 m/s l=2m
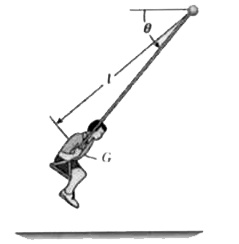
The correct Answer is:6m/s2,125N
Step by step video & image solution for At the instant theta=theta_(1) in the fig the boy's center of mass G has speed v_(G), Determine the rate of increase in his speed and the tension in each of the two supporting cords of the swing at this instant. The boy has a weight W. Neglect his size and the mass of the seat and cords. Given m=20kg theta_(I)=53^(@), v_(G)=3 m/s l=2m by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.
Topper's Solved these Questions
- BOOK - RESNICK AND HALLIDAYCHAPTER - CIRCULAR MOTIONEXERCISE - PRACTICE QUESTIONS(SINGLE CORRECT CHOICE TYPE)40videos
CIRCULAR MOTION
- BOOK - RESNICK AND HALLIDAYCHAPTER - CIRCULAR MOTIONEXERCISE - PRACTICE QUESTIONS(MORE THAN ONE CORRECT CHOICE TYPE)6videos
CIRCULAR MOTION
- BOOK - RESNICK AND HALLIDAYCHAPTER - CIRCULAR MOTIONEXERCISE - CHECK POINTS6videos
CIRCULAR MOTION
- BOOK - RESNICK AND HALLIDAYCHAPTER - CIRCUITSEXERCISE - Practice Questions (Integer Type)3videos
CIRCUITS
- BOOK - RESNICK AND HALLIDAYCHAPTER - CURRENT AND RESISTANCEEXERCISE - Practice Questions (Integer Type)3videos
CURRENT AND RESISTANCE
Similar Questions
The pendulum bob B of mass M is released from rest when θ=0∘. Determine the intitial tension in the cord and also at the instant the bob reaches point D,θ=θ1. Neglect the size of the bob. Give M=3kg,θ1=45∘,L=2m,g=9.81m/s2.
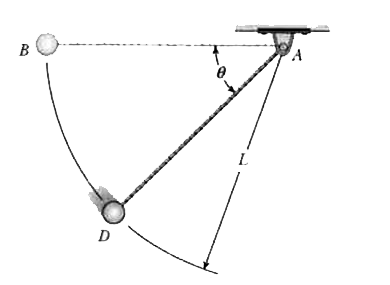 View Solution
View SolutionThe bob of a simple pendulum of length 1 m has mass 100 g and a speed of 1.4 m/s at the lowest point in its path. Find the tension in the stirng at this instant.
View Solution
Knowledge Check
- Question 1 - Select One
A simple pendulum of length L swings in a vertical plane. The tension of the string when it makes an angle θ with the vertical and the bob of mass m moves with a speed v is (g is the gravitational acceleration)
Amv2/LBmgcosθ+mv2/LCmgcosθ−mv2/LDmgcosθ - Question 2 - Select One
A man running has half the kinetic energy of a boy of half his mass. The man speeds up by 1 m/s and then has kinetic energy as that of the boy. What were the original speeds of man and the boy ?
A√2m/s:2√2m/sB(√2−1)m/s:2(√2−1)m/sC(√2+1)m/s:2(√2+1)m/sD1√2m/s,√2m/s
If the crest of the hill has a radius of curvature p determine the maximum constnat speed at which the car can travel over it without leaving the surface of the road. Neglect the size of the car in the calculation. The car has mass m. Given p=40m, m=1700kg, g=10m/s2
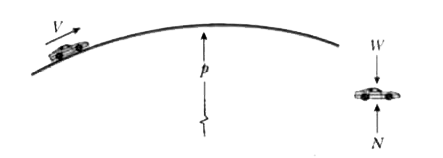 View Solution
View SolutionThe man has weight W and lies against the cushion for which the coefficient of static friction is μs. Determine the resultant normal and frictional forces the cushion exerts on him if due to rotation about the z axis, he has constant speed v. Neglect the size of the man. Given, W=150, Ib,μs=0.5,v=20fts,θ=60∘,d=8ft,g=32ft/s2
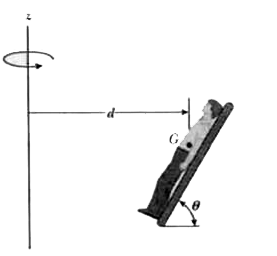 View Solution
View SolutionA uniform rod of length 1m and mass 2kg is suspended. Calculate tension T (in N) in the string at the instant when the right string snaps (g=10m/s2).
 View Solution
View SolutionA particle of mass m is projected with a speed v0 at an angle of projection θ with horizontal. Find the torque of the weight of the particle about the point of projection after time v0sinθg
View SolutionSuppose the bob of the previous problem has a speed of 1.4 m/s when the string makes an angle of 0.20 radian with the vertical. Find the tension at this instant. You can use cosθ=1−θ22andsinθ=θ for small theta.
View SolutionA small ball of mass m is attached to a string of lengh R and moves in a vertical circle with a speed v m/s about a fixed point O as illustrated in fig. Find the tension in the string, when it makes an angle θ with the vertical, in terms of v,θ and g.
View Solution

