Charge q2 of mass m revolves around a stationary charge q1 in a circulare orbit of radius r. The orbital periodic time of q2 would be
[4π2mr3kq1q2]1/2
[kq1q24π2mr3]1/2
[4π2mr4kq1q2]1/2
[4π2mr2kq1q2]1/2
The correct Answer is:A
To find the orbital periodic time of charge q2 revolving around a stationary charge q1, we can follow these steps:
Step 1: Identify the forces acting on the charge q2
The charge q2 experiences an electrostatic force due to the charge q1, which acts as the centripetal force required for circular motion.
Step 2: Write the expression for the electrostatic force
The electrostatic force F between two point charges is given by Coulomb's law:
F=k⋅|q1⋅q2|r2
where k is Coulomb's constant, q1 and q2 are the magnitudes of the charges, and r is the distance between them.
Step 3: Set the electrostatic force equal to the centripetal force
For circular motion, the centripetal force Fc required to keep the charge q2 in orbit is given by:
Fc=m⋅ω2⋅r
where m is the mass of the charge q2 and ω is the angular velocity.
Setting the electrostatic force equal to the centripetal force, we have:
k⋅|q1⋅q2|r2=m⋅ω2⋅r
Step 4: Rearrange the equation to solve for ω2
Rearranging the equation gives:
ω2=k⋅|q1⋅q2|m⋅r3
Step 5: Find ω
Taking the square root of both sides, we find:
ω=√k⋅|q1⋅q2|m⋅r3
Step 6: Calculate the time period T
The time period T of one complete revolution is related to the angular velocity ω by the formula:
T=2πω
Substituting the expression for ω:
T=2π√k⋅|q1⋅q2|m⋅r3
Step 7: Simplify the expression for T
This can be simplified as follows:
T=2π√m⋅r3k⋅|q1⋅q2|
Final Expression
Thus, the orbital periodic time T of charge q2 is:
T=2π√m⋅r3k⋅|q1⋅q2|
The charge q2 experiences an electrostatic force due to the charge q1, which acts as the centripetal force required for circular motion.
The electrostatic force F between two point charges is given by Coulomb's law:
F=k⋅|q1⋅q2|r2
where k is Coulomb's constant, q1 and q2 are the magnitudes of the charges, and r is the distance between them.
For circular motion, the centripetal force Fc required to keep the charge q2 in orbit is given by:
Fc=m⋅ω2⋅r
where m is the mass of the charge q2 and ω is the angular velocity.
k⋅|q1⋅q2|r2=m⋅ω2⋅r
Rearranging the equation gives:
ω2=k⋅|q1⋅q2|m⋅r3
Taking the square root of both sides, we find:
ω=√k⋅|q1⋅q2|m⋅r3
The time period T of one complete revolution is related to the angular velocity ω by the formula:
T=2πω
Substituting the expression for ω:
T=2π√k⋅|q1⋅q2|m⋅r3
This can be simplified as follows:
T=2π√m⋅r3k⋅|q1⋅q2|
Thus, the orbital periodic time T of charge q2 is:
T=2π√m⋅r3k⋅|q1⋅q2|
Topper's Solved these Questions
- ELECTROSTATICSBOOK - DC PANDEY ENGLISHCHAPTER - ELECTROSTATICSEXERCISE - Assertion and Reason
15 Videos
- ELECTROSTATICSBOOK - DC PANDEY ENGLISHCHAPTER - ELECTROSTATICSEXERCISE - Match the columns
5 Videos
- ELECTROSTATICSBOOK - DC PANDEY ENGLISHCHAPTER - ELECTROSTATICSEXERCISE - Check point 1.5
20 Videos
- ELECTROSTATIC POTENTIAL AND CAPACITORSBOOK - DC PANDEY ENGLISHCHAPTER - ELECTROSTATIC POTENTIAL AND CAPACITORSEXERCISE - (C) Chapter exercises
50 Videos
- GRAVITATIONBOOK - DC PANDEY ENGLISHCHAPTER - GRAVITATIONEXERCISE - All Questions
135 Videos
Similar Questions
A particle of mass m carrying charge '+q1' is revolving around a fixed charge '−q2' in a circular path of radius r. Calculate the period of revolution.
View SolutionA particle of mass m carrying a charge −q1 starts moving around a fixed charge +q2 along a circulare path of radius r. Find the time period of revolution T of charge −q1.
View Solution
Knowledge Check
The additional kinetic energy to be provided to a satellite of mass m revolving around a planet of mass M, to transfer it forms a circular orbit of radius R1 to another of radius R2(R2>R1) is
AGmM(1R21−1R22)BGmM(1R1−1R2)C2GmM(1R1−1R2)D12GmM(1R1−1R2)
An isolated triple star systerm consists of two identical stars, each of mass m and f fixed star of mass M. They revolve around the central star in the same circular orbit to radius r. The orbiting stars are always at opposite ends of a diameter of the orbit. The time period of revolution of each star around the fixed is equal to:

View SolutionTwo satellites of masses M and 16 M are orbiting a planet in a circular orbitl of radius R. Their time periods of revolution will be in the ratio of
View SolutionA positive point charge +Q is fixed in space .A negative point charge −q of mass m revolves around a fixed charge in elliptical orbits .The fixed charge +Qis at one focus of the ellipse.The only force acting on negative charge is the electrostatic force due to positive charge is the electrostatic force due to positive charge.Then which of the following statement is true
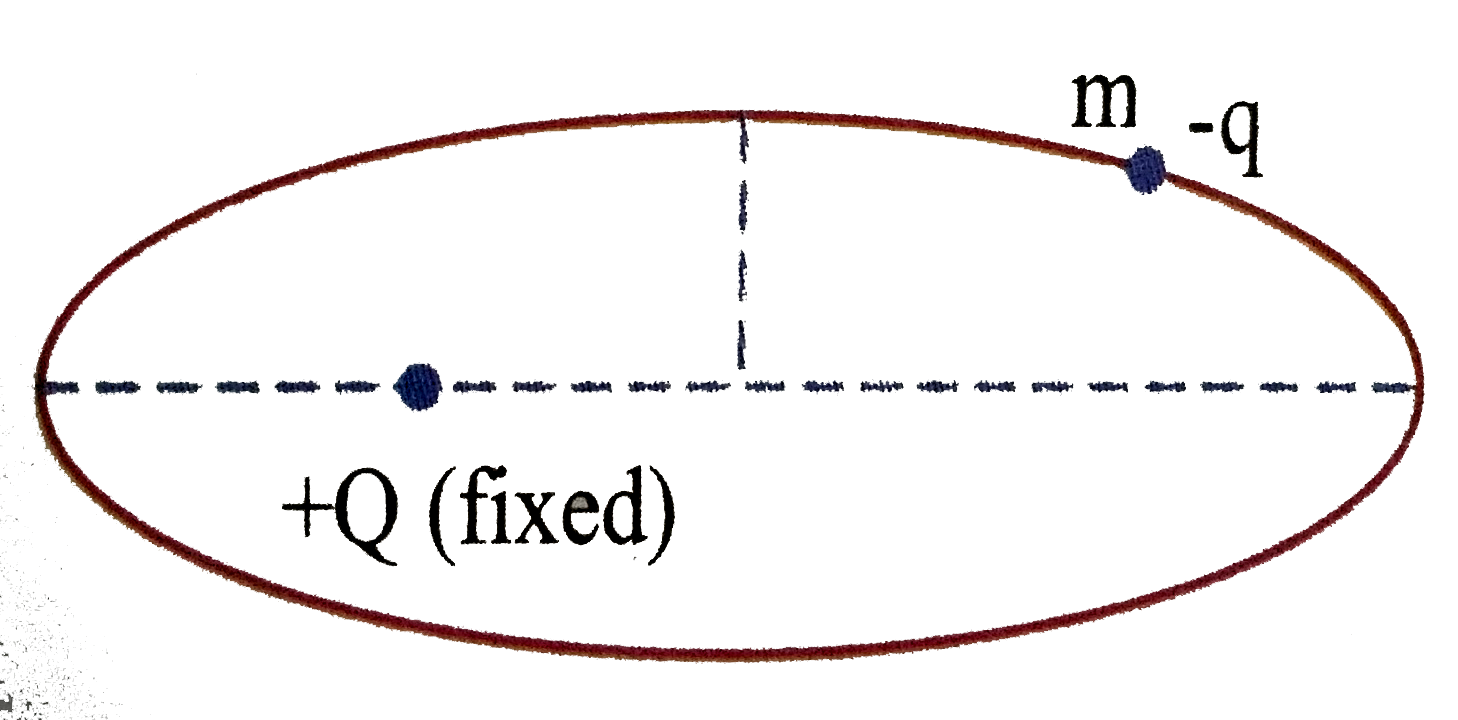
View SolutionThe satellite of mass m revolving in a circular orbit of radius r around the earth has kinetic energy E. then, its angular momentum will be
View SolutionA particle of mass m and charge -q circulates around a fixed charge q in a circle radius under electrostatic force. The total energy of the system is (k= (14πε0))
View SolutionIf an electron revolves around a nucleus in a circular orbit of radius R with frequency n, then the magnetic field produced at the center of the nucleus will be
View SolutionIf L is the angular momentum of a satellite revolving around earth is a circular orbit of radius r with speed v , then
View Solution
