Consider a solid cylinder of the density $ρ_{s}$ cross section area A and h ploating in a liquid of density $ρ_{l}$ as shown in figure $(ρ_{l} > ρ_{s})$ . It is depressed sligtly and allowed to oscillation.

Video Solution

Text Solution

Verified by Experts

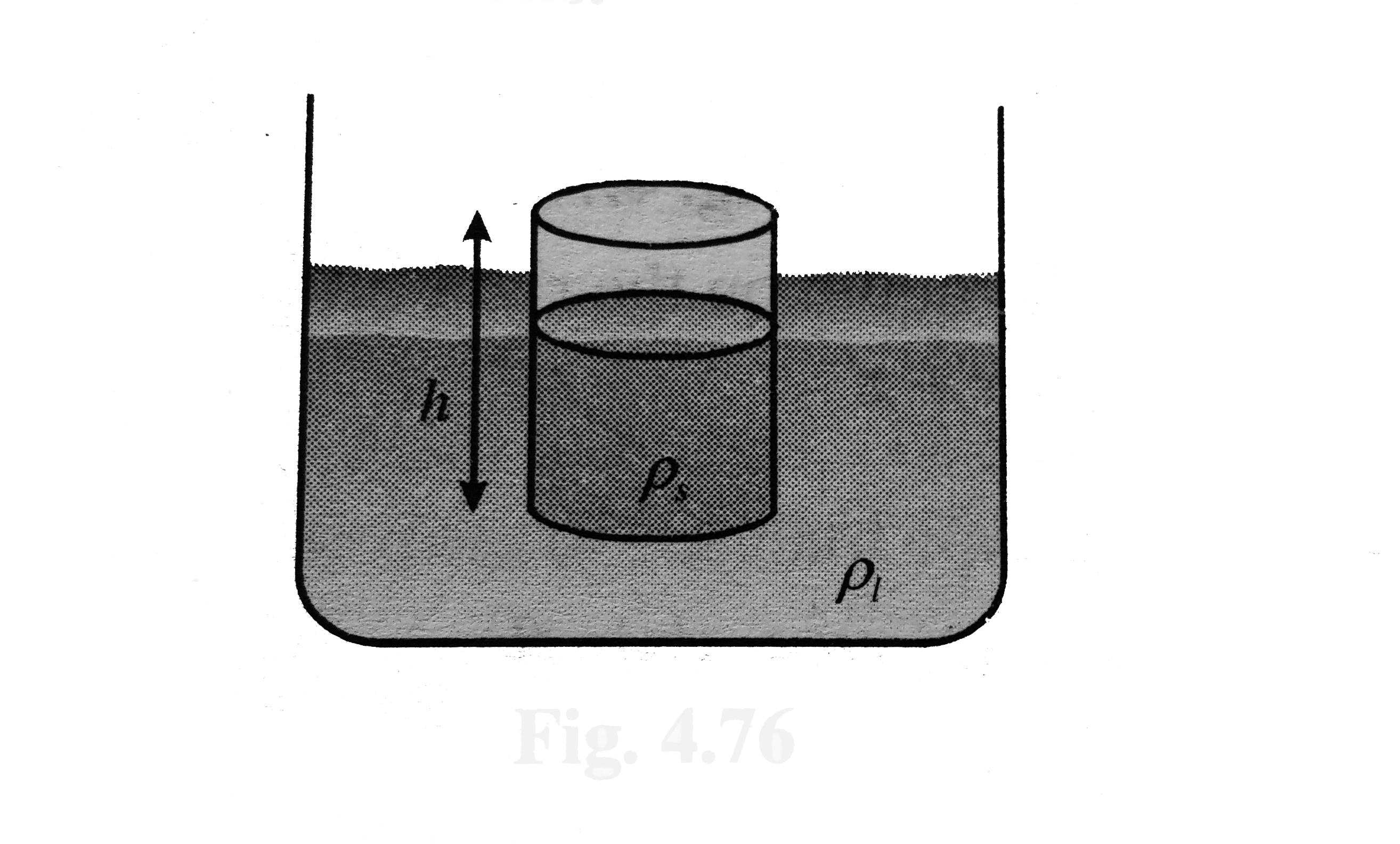
At equilibrium the net force on the cylinder is zero in the vertical direction.
$F_{net} = B - W = 0, B =$ the buoyancy and W = the weight of the cylinder direction.
When the cylinder is depresed slightly by x, the buoyancy increases from $B \to B + σ B$ , where $σ B = | x | ρ_{l} A g$
The equation of motion is, therefore.
$ρ, A h \frac{d^{2} x}{{d t}^{2} = - \frac{ρ_{l} g}{ρ_{s} h}}$
and the angular frequency, $ω$ , is
$ω = \sqrt{\frac{g ρ_{l}}{h ρ_{s}}}$

Updated on:21/07/2023

An object with uniform density ρ is attached to a spring that is known to stretch linearly with applied force as shown below.
When the spring-object system is immersed in a liquid of density $ρ_{1}$ as shown in the figure, the spring stretches by an amount $x_{1} (ρ > ρ_{1})$ . When the experiment is repeated in a liquid of density $ρ_{2} > ρ_{1}$ , the spring stretches by an amount $x_{2}$ . Neglecting any buoyant force on the spring, the density of the object is

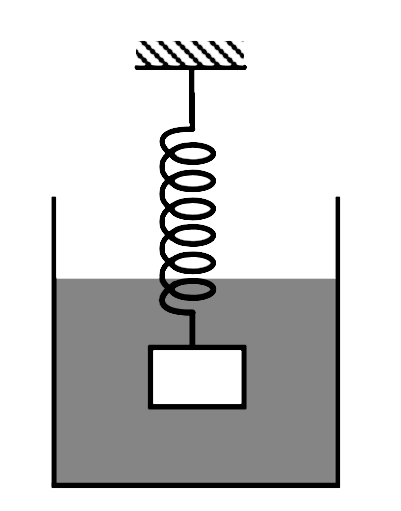A $ρ = \frac{ρ_{1} x_{1} - ρ_{2} x_{2}}{x_{1} - x_{2}}$
B $ρ = \frac{ρ_{1} x_{2} - ρ_{2} x_{1}}{x_{2} - x_{1}}$
C $ρ = \frac{ρ_{1} x_{2} + ρ_{2} x_{1}}{x_{2} + x_{1}}$
D $ρ = \frac{ρ_{1} x_{2} + ρ_{2} x_{1}}{x_{1} + x_{2}}$
The speed of transverse waves in a wire of length L , density $ρ$ , cross - sectional area A and stretched with a tension T is given by
A $\sqrt{\frac{T L}{ρ A}}$
B $\sqrt{\frac{T}{A ρ}}$
C $\sqrt{\frac{T}{ρ L}}$
D $\sqrt{\frac{T ρ}{A L}}$