Consider two rings of copper wire. One ring is scaled up version of the other, twice large in all regards (radius, cross sectional radius). If current around the rings are driven by equal voltage source then choose the CORRECT alternative(s). Assume that cross-sectional radius is very small as compared to radius of rings :
Resistance of larger ring is half of the smaller ring
Current in the larger ring is two times that in the smaller ring
Magnetic field at their centres are same
Magnetic field at centre oflarger ring is twice as that at the centre of smaller ring
Step by step video & image solution for Consider two rings of copper wire. One ring is scaled up version of the other, twice large in all regards (radius, cross sectional radius). If current around the rings are driven by equal voltage source then choose the CORRECT alternative(s). Assume that cross-sectional radius is very small as compared to radius of rings : by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.
Topper's Solved these Questions
- BOOK - PHYSICS GALAXY - ASHISH ARORACHAPTER - MAGNETIC EFFECTS OF CURRENT AND MAGNETISMEXERCISE - U.N.P79videos
MAGNETIC EFFECTS OF CURRENT AND MAGNETISM
- BOOK - PHYSICS GALAXY - ASHISH ARORACHAPTER - MAGNETIC EFFECTS OF CURRENT AND MAGNETISMEXERCISE - Numerical MCQ61videos
MAGNETIC EFFECTS OF CURRENT AND MAGNETISM
- BOOK - PHYSICS GALAXY - ASHISH ARORACHAPTER - Kinetic Theory of Gases and Gas LawsEXERCISE - Unsolved Numerical Problems for Preparation of NSEP, INPhO & IPhO64videos
Kinetic Theory of Gases and Gas Laws
- BOOK - PHYSICS GALAXY - ASHISH ARORACHAPTER - NUCLEAR PHYSICS AND RADIOACTIVITYEXERCISE - Concptual MCQs48videos
NUCLEAR PHYSICS AND RADIOACTIVITY
Similar Questions
A metal ring of initial radius r and cross-sectional area A is fitted onto a wooden disc of radius R > r. If Youngs modules of metal is Y then tension in the ring is
View SolutionA uniform ring of radius R and made up of a wire of cross - sectional radius r is rotated about its axis with a frequency f. If density of the wire is ρ and young's modulus is Y . Find the fractional change in radius of the ring .
View Solution
Knowledge Check
- Question 1 - Select One
A metal ring of initial radius r and cross-sectional area A is fitted onto a wooden disc of radius R > r. If Youngs modules of metal is Y then tension in the ring is
AAYRrBAY(R−r)rCYA(R−rr)DYrAR - Question 2 - Select One or More
A unifrom metallic ring of mass m, radisus R, cross sectional area 'a' and young'/s modulus Y kis kept on a smooth cone of radius 2R and semivertex angle 45∘ as shown. [Assume that extension in the ring is small]
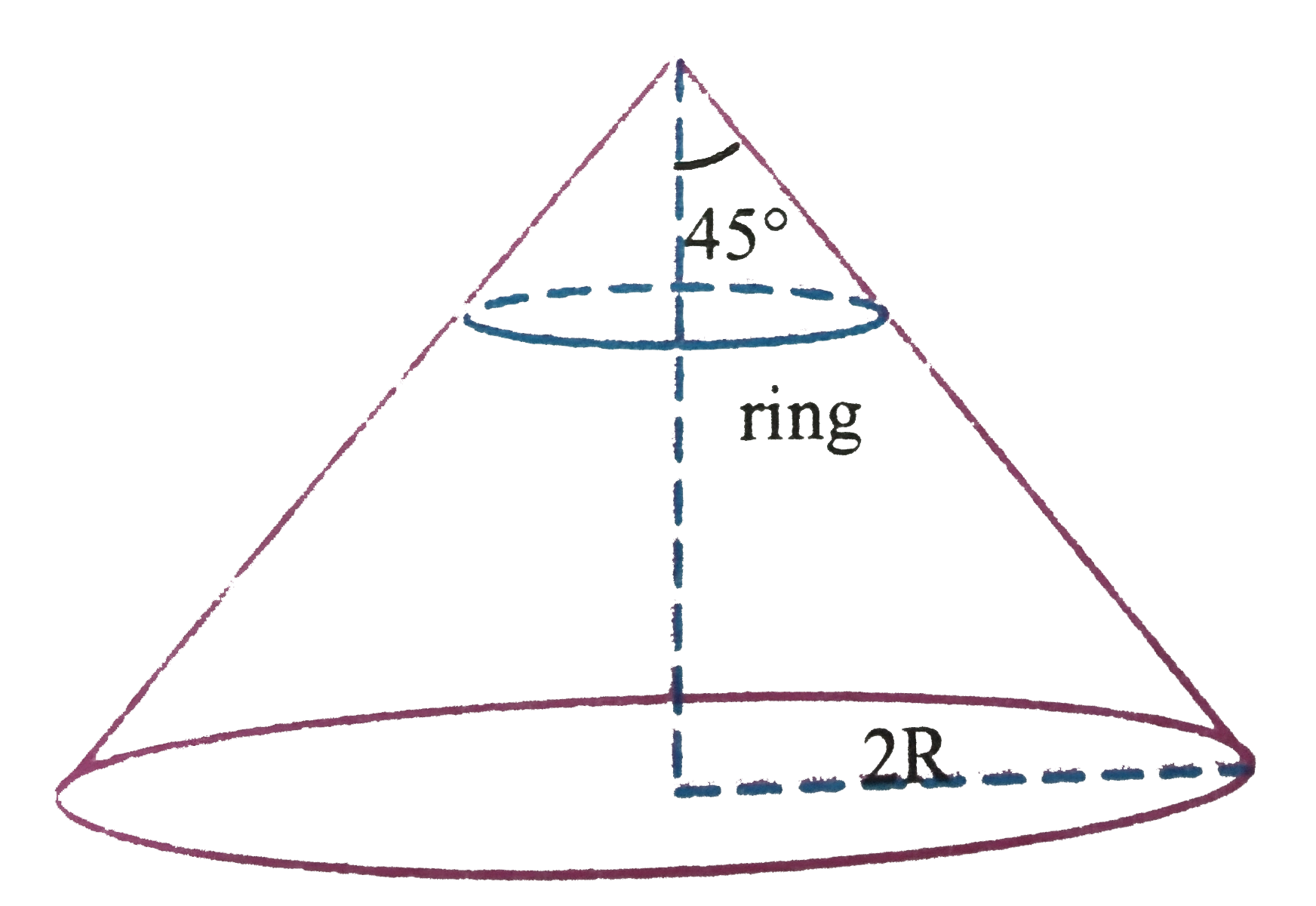 AThe tension in the ring will be same through outBThe tension in the ring will be independent of radius of ring.CThe extension in the ring will be mgRaYDElastic potential energy should in the ring will be m2g2R8πYa
AThe tension in the ring will be same through outBThe tension in the ring will be independent of radius of ring.CThe extension in the ring will be mgRaYDElastic potential energy should in the ring will be m2g2R8πYa - Question 3 - Select One
A steel ring of radius r and cross section area A is fitted on to a wooden disc of radius R(R>r). If Young's modulus be E, then the force with which the steel ring is expanded is
AAERrBAE(R−rr)CEA(R−rA)DErAR
A thin wire ring of radius a and resitance r is located inside a long solenoid is equal to l, its cross-sectional radius , to b. At a certain moment the solenoid was connected to a source of a constant voltage V. The total resistance of the circuit is equal to R. Assuming the the radial force acting per unit length of the ring.
View SolutionThe figure shows a long cylinder and its cross section. There are N (N is a large number) wire on the curved surface of the cylinder at uniform spacing and paral- lel to its axis. Each wire has current I and cross sectional radius of wires are small compared to radius R of the cyl- inder. Find magnetic field at a distance x from the axis of the cylinder for (a)x<R (b) x>R
 View Solution
View SolutionA ring of mass m and radius R rests in equilibrium on a smooth cone of semi-vertical angle 45∘ as shown. The radius of the cone is 2R. the radius of circular cross section of the ring is r(r<<R).
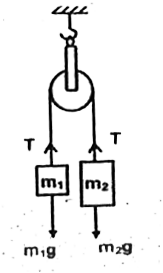
What will be the speed of transverse wave on the ring?View SolutionA ring of mass m and radius R rests in equilibrium on a smooth cone of semi-vertical angle 45∘ as shown. The radius of the cone is 2R. the radius of circular cross section of the ring is r(r<<R).
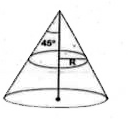
What will be the tension in the ring?View SolutionTwo conducting wires A and B are made of same material. If the length of B is twice that of A and the radius of circular cross-section of A is twice that of B, then resistances RA and RB are in the ratio
View Solution

