Demonstrate that Maxwell's equations ∇×E=−∂B/∂t and ∇.B=0 are compatible, i.e., the first one does not contradict the secound one.
From →∇×→E=∂→B∂t
we get on taking divergence
0=−∂∂t div →B
This is compatible with div →B=0
Topper's Solved these Questions
- BOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - ELECTRODYNAMICSEXERCISE - Motion Of Charged Particle In Magnetic Field36videos
ELECTRODYNAMICS
- BOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - ELECTRODYNAMICSEXERCISE - Constant Magnetic Fiels - Magnetics69videos
ELECTRODYNAMICS
- BOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - ELECTRICITY AND MAGNETISMEXERCISE - All Questions6videos
ELECTRICITY AND MAGNETISM
- BOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - ELECTROMAGNETISMEXERCISE - All Questions24videos
ELECTROMAGNETISM
Similar Questions
Knowledge Check
- Question 1 - Select One
Maxwell’s equation ∮→E.→dl=d→B.→Adt is a statement of-
AAmpere’s lawBFaraday’s law of inductionCGauss’s law of electricityDGauss’s law of magnetism - Question 2 - Select One
In 1931, Pauling defined the electronegativity of an atom as the tendency of the atom to attract electrons to itself when combined in a compound. The implication is that when a covalent bond is formed, the electrons used for bonding need not be shared equally by both atoms. If the bonding electrons spend more time around one atom, that atom will have a ∂−charge, and consequently the other atom will have a ∂+ charge. In the extreme case, where the bonding electrons are round one atom all of the time, the bond is ionic. Pauling and others have attempted to relate the electronegativity difference between two atoms to the amount of ionic character in the bond between them.
Mulliken
In 1934, Mulliken suggested an alternative approach to electronegativity based on the ionization energy and electron affinity of an atom. Consider two atoms A and B. If an electron is transferred from A to B, forming ions A+ and B−, then the energy change is the ionization energy of atom A(IA) minus the electron affinity of atom B(EB), that is IA−EB. Alternatively, if the electron was transferred the other way to give B+ and A− ions, then the energy changed would be IB−IA. If A+ and B− are actually formed, then this process require less energy , and
(IA−EB)<(IB−EA)
Rerranging (IA+EA)<(IA−EB)
Now with respect to electronegativity for the same change, E.NA<E.NB.
Thus Mulliken suggested that electronegativity is proportional to I.E+E.A and could be regarded as the average of the ionization energy and the electron affinity of an atom.
Electronegativity =(I+E)2
Mulliken used I and E values measured in electron volts, and the values were about 2.8 times alrger than the Pauling values. It is to be noted that I.E. and E.A values are defined for singular gaseous atoms.
For a reaction A(g)+B(g)rarAB(S) the enthalpy change for the recation will be [assuming AB(s) to be an ionic compound] If I.EA+E.AA<I.EB+E.ABAΔHI.E.A+ΔHegA+ΔHI.EAB(s)BΔHegA+ΔHI.EB+ΔHL.EAB(s)CI.EA+E.AB+ΔHL.EABsDI.EB+E.AB+ΔL.EAB(s) - Question 3 - Select One
In 1931, Pauling defined the electronegativity of an atom as the tendency of the atom to attract electrons to itself when combined in a compound. The implication is that when a covalent bond is formed, the electrons used for bonding need not be shared equally by both atoms. If the bonding electrons spend more time around one atom, that atom will have a ∂−charge, and consequently the other atom will have a ∂+ charge. In the extreme case, where the bonding electrons are round one atom all of the time, the bond is ionic. Pauling and others have attempted to relate the electronegativity difference between two atoms to the amount of ionic character in the bond between them.
Mulliken
In 1934, Mulliken suggested an alternative approach to electronegativity based on the ionization energy and electron affinity of an atom. Consider two atoms A and B. If an electron is transferred from A to B, forming ions A+ and B−, then the energy change is the ionization energy of atom A(IA) minus the electron affinity of atom B(EB), that is IA−EB. Alternatively, if the electron was transferred the other way to give B+ and A− ions, then the energy changed would be IB−IA. If A+ and B− are actually formed, then this process require less energy , and
(IA−EB)<(IB−EA)
Rerranging (IA+EA)<(IA−EB)
Now with respect to electronegativity for the same change, E.NA<E.NB.
Thus Mulliken suggested that electronegativity is proportional to I.E+E.A and could be regarded as the average of the ionization energy and the electron affinity of an atom.
Electronegativity =(I+E)2
Mulliken used I and E values measured in electron volts, and the values were about 2.8 times alrger than the Pauling values. It is to be noted that I.E. and E.A values are defined for singular gaseous atoms.
We have X atoms of A. if all the atoms gain one electron each the energy released is aeV. If Y atoms of A lose one electron each, then the energy absorbed is beV. Then the electronegativity of A on the Mulliken scale will be:Aa+b2B12(aX+bY)C12(a+b)×NaD12(aX+bY)×Na
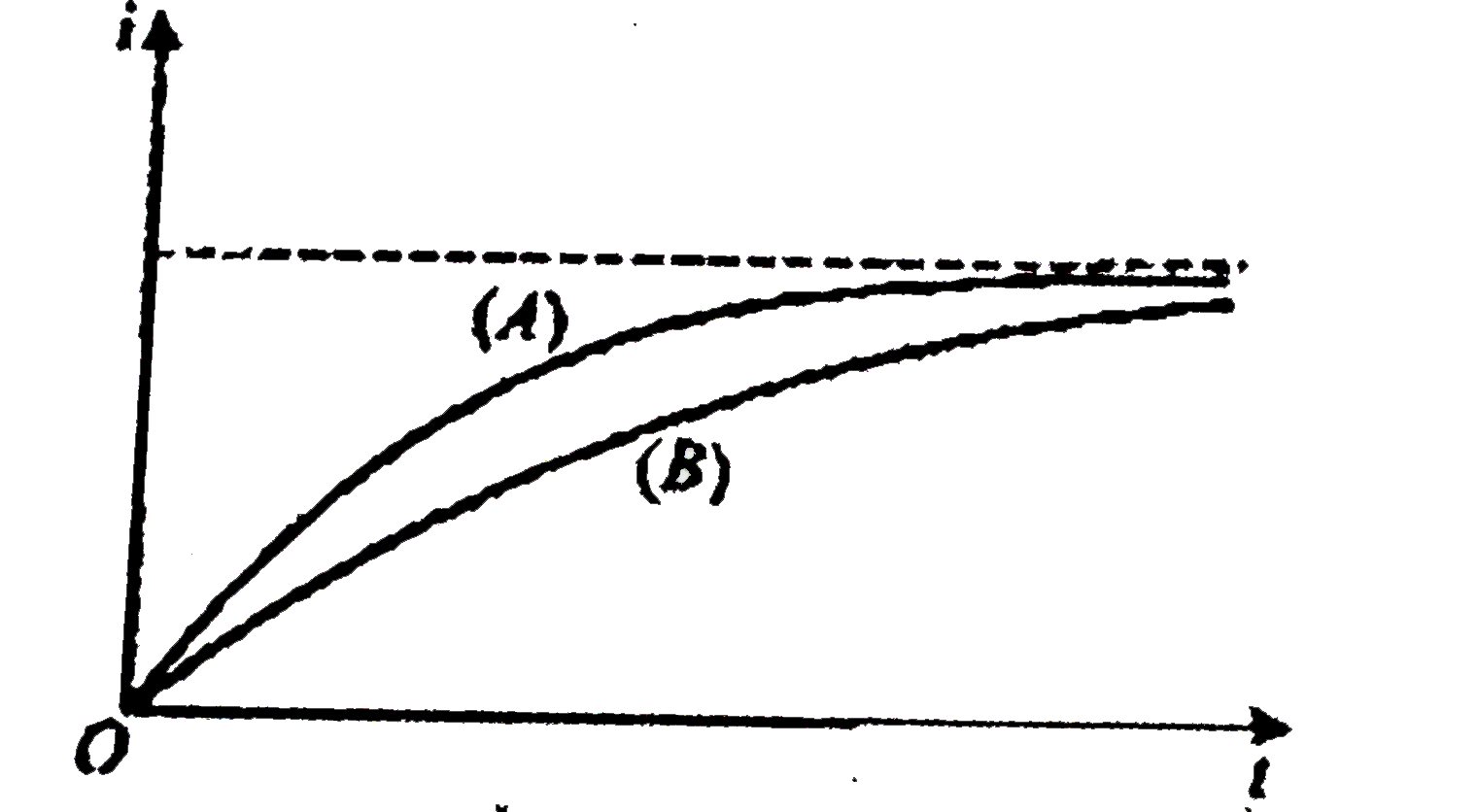
A circuit consisting of a constant EMF E, a self-inductance L and a resistance R in sereis is closed at time t=0. The relation between the current i in the circuit and the time t is as shown by the curve. A in the figure-5.321. when one or more of parameters E,R and L are changed, the curve B is obtained. Then it is possible that:
 View Solution
View SolutionIf fandg are one-one functions, then (a)f+g is one one (b)fg is one one (c)fog is one one (d)noneofthese
View SolutionIn 1931, Pauling defined the electronegativity of an atom as the tendency of the atom to attract electrons to itself when combined in a compound. The implication is that when a covalent bond is formed, the electrons used for bonding need not be shared equally by both atoms. If the bonding electrons spend more time around one atom, that atom will have a ∂−charge, and consequently the other atom will have a ∂+ charge. In the extreme case, where the bonding electrons are round one atom all of the time, the bond is ionic. Pauling and others have attempted to relate the electronegativity difference between two atoms to the amount of ionic character in the bond between them.
Mulliken
In 1934, Mulliken suggested an alternative approach to electronegativity based on the ionization energy and electron affinity of an atom. Consider two atoms A and B. If an electron is transferred from A to B, forming ions A+ and B−, then the energy change is the ionization energy of atom A(IA) minus the electron affinity of atom B(EB), that is IA−EB. Alternatively, if the electron was transferred the other way to give B+ and A− ions, then the energy changed would be IB−IA. If A+ and B− are actually formed, then this process require less energy , and
(IA−EB)<(IB−EA)
Rerranging (IA+EA)<(IA−EB)
Now with respect to electronegativity for the same change, E.NA<E.NB.
Thus Mulliken suggested that electronegativity is proportional to I.E+E.A and could be regarded as the average of the ionization energy and the electron affinity of an atom.
Electronegativity =(I+E)2
Mulliken used I and E values measured in electron volts, and the values were about 2.8 times alrger than the Pauling values. It is to be noted that I.E. and E.A values are defined for singular gaseous atoms.
For the reaction A(g)+e−→A+(g)ΔHr=ΔHeg. The enthalpy change will be equal to (magnitude wise):View SolutionWrite the dimensions of a×bin the relation E=b−x2at, where E is the energy , x is the displacement , and t is the time.
View SolutionA circuit consisting of a constant EMF E, a self-inductance L and a resistance R in sereis is closed at time t=0. The relation between the current i in the circuit and the time t is as shown by the curve. A in the figure-5.321. when one or more of parameters E,R and L are changed, the curve B is obtained. Then it is possible that:
 View Solution
View Solution

