Density of a liquid varies with depth as ρ=αh. A small ball of density ρ0 is released from the free surface of the liquid. Then
the ball will execute SHM of amplitude ρ0α
the mean position of the ball will be at a depth ρ02α
the ball will sink to a maximum depth of ρ0α
The ball sink to a maximum depth of ρ0α
The correct Answer is:A, C, D
To solve the problem of a small ball released in a liquid with varying density, we can follow these steps:
Step 1: Understand the Density Variation
The density of the liquid varies with depth according to the equation:
ρ=αh
where ρ is the density of the liquid at depth h, and α is a constant.
Step 2: Identify the Forces Acting on the Ball
When the ball of density ρ0 is released from the surface, two main forces act on it:
1. The gravitational force (weight) acting downward:
Fg=mg=ρ0Vg
where V is the volume of the ball.
2. The buoyant force (upthrust) acting upward, which is given by:
Fb=ρVg
Here, ρ is the density of the liquid at the depth where the ball is submerged.
Step 3: Establish the Condition for Equilibrium
The ball will reach an equilibrium position when the gravitational force equals the buoyant force:
Fg=Fb
Substituting the expressions for the forces:
ρ0Vg=ρVg
Cancelling Vg from both sides (assuming V≠0):
ρ0=ρ
Substituting the density variation:
ρ0=αh
From this, we can find the depth h:
h=ρ0α
Step 4: Analyze the Motion of the Ball
When the ball is released, it will initially accelerate downward due to the weight being greater than the buoyant force. As it moves downward, the buoyant force increases (since the density of the liquid increases with depth).
Step 5: Determine the Maximum Depth
The ball will oscillate about the equilibrium position. The maximum depth it reaches can be determined by analyzing the forces at the extreme position. When the ball is at maximum depth, the forces will again balance, but at a greater depth.
The maximum depth occurs when:
ρ0=αhmax
At the maximum depth, the ball will reach a position where:
hmax=2ρ0α
Step 6: Calculate the Amplitude of SHM
The amplitude of the simple harmonic motion (SHM) can be found by taking the difference between the maximum depth and the mean position:
Amplitude=hmax−hmean=2ρ0α−ρ0α=ρ0α
Conclusion
Based on the analysis, we conclude:
- The ball will execute SHM with an amplitude of ρ0α.
- The maximum depth the ball will sink to is 2ρ0α.
Final Answer
- The ball will sink to a maximum depth of 2ρ0α (Option D is correct).
---
The density of the liquid varies with depth according to the equation:
ρ=αh
where ρ is the density of the liquid at depth h, and α is a constant.
When the ball of density ρ0 is released from the surface, two main forces act on it:
1. The gravitational force (weight) acting downward:
Fg=mg=ρ0Vg
where V is the volume of the ball.
2. The buoyant force (upthrust) acting upward, which is given by:
Fb=ρVg
Here, ρ is the density of the liquid at the depth where the ball is submerged.
The ball will reach an equilibrium position when the gravitational force equals the buoyant force:
Fg=Fb
Substituting the expressions for the forces:
ρ0Vg=ρVg
Cancelling Vg from both sides (assuming V≠0):
ρ0=ρ
Substituting the density variation:
ρ0=αh
From this, we can find the depth h:
h=ρ0α
When the ball is released, it will initially accelerate downward due to the weight being greater than the buoyant force. As it moves downward, the buoyant force increases (since the density of the liquid increases with depth).
The ball will oscillate about the equilibrium position. The maximum depth it reaches can be determined by analyzing the forces at the extreme position. When the ball is at maximum depth, the forces will again balance, but at a greater depth.
ρ0=αhmax
At the maximum depth, the ball will reach a position where:
hmax=2ρ0α
The amplitude of the simple harmonic motion (SHM) can be found by taking the difference between the maximum depth and the mean position:
Amplitude=hmax−hmean=2ρ0α−ρ0α=ρ0α
Based on the analysis, we conclude:
- The ball will execute SHM with an amplitude of ρ0α.
- The maximum depth the ball will sink to is 2ρ0α.
- The ball will sink to a maximum depth of 2ρ0α (Option D is correct).
Topper's Solved these Questions
- SIMPLE HARMONIC MOTIONBOOK - DC PANDEY ENGLISHCHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - Comprehension types
18 Videos
- SIMPLE HARMONIC MOTIONBOOK - DC PANDEY ENGLISHCHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - Matrix matching type questions
13 Videos
- SIMPLE HARMONIC MOTIONBOOK - DC PANDEY ENGLISHCHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - JEE Advanced
34 Videos
- SEMICONDUCTORS AND ELECTRONIC DEVICESBOOK - DC PANDEY ENGLISHCHAPTER - SEMICONDUCTORS AND ELECTRONIC DEVICESEXERCISE - More than One Option is Correct
3 Videos
- SOLVD PAPERS 2017 NEET, AIIMS & JIPMERBOOK - DC PANDEY ENGLISHCHAPTER - SOLVD PAPERS 2017 NEET, AIIMS & JIPMEREXERCISE - Solved paper 2018(JIPMER)
38 Videos
Similar Questions
A ball of density ρ is released from deep inside of a liquid of density 2ρ. It will move up
View SolutionA liquid of density ρ is filled in a vessel up to height H and a hole of cross section area A is made at a depth h below the free surface of liquid. The speed of liquid coming out of the hole is independent of
View SolutionA uniform solid sphere of radius R is in equilibrium inside a liquid whose density varies with depth from free surface as ρ=ρ0(1+hh0) where h is depth from free surface. Density of sphere σ will be :
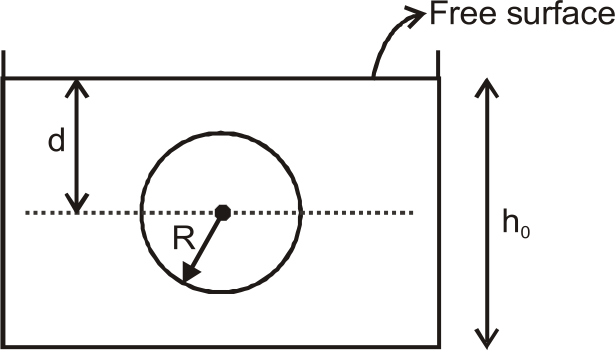
View SolutionA beaker containing a liquid of density ρ moves up with an acceleration a. The pressure due to the liquid at a depth h below the free surface of the liquid is.
View SolutionA liquid of density ρ is in a bucket that spins with angular velocity 'ω' as shown in figure. Prove that the free surface of the liquid has a parabolic shape. Find equation of this.
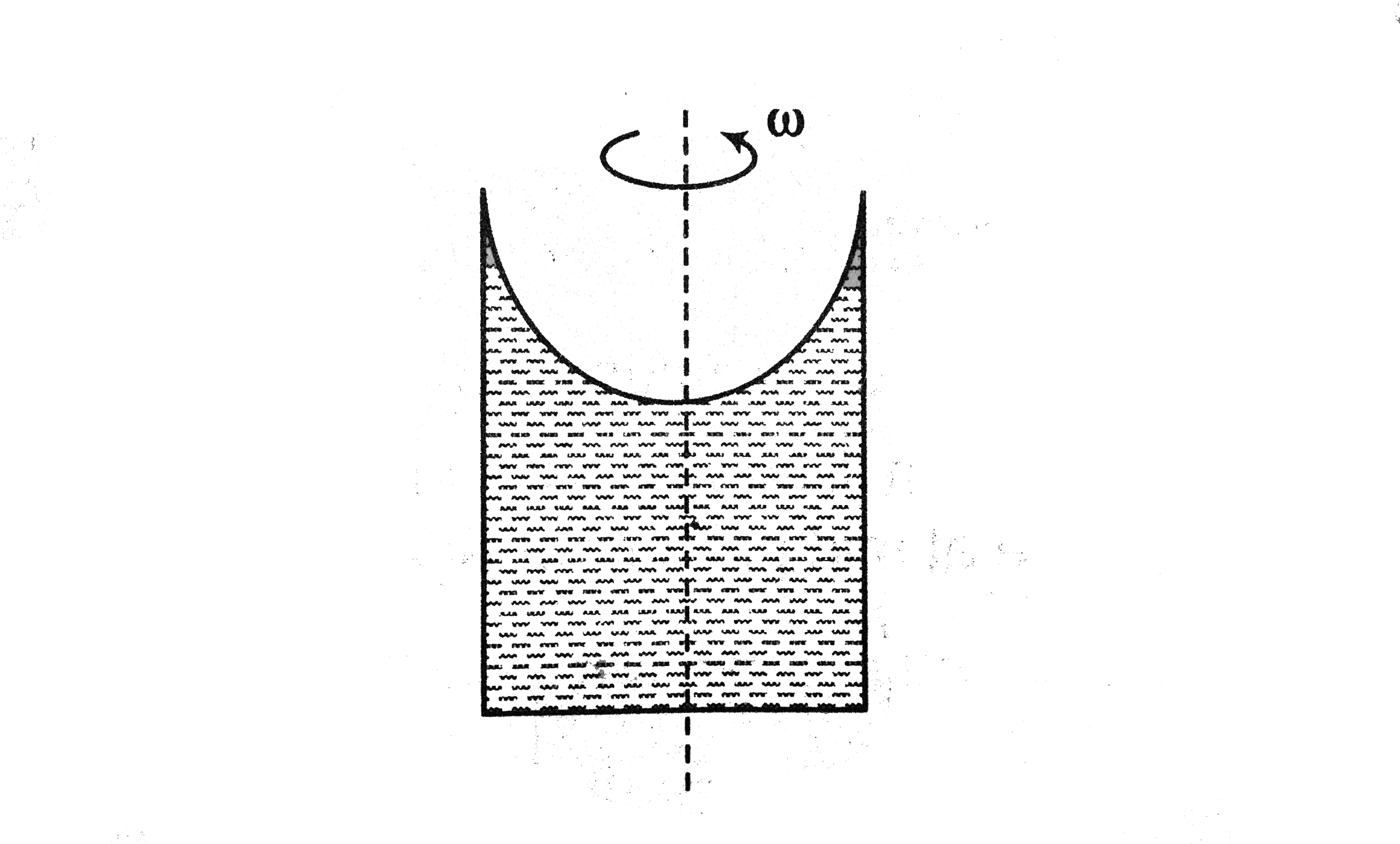 .
.
View SolutionA small ball of density rho_(0) is released from the surface of a liquid whose density varies with depth h as ρ=ρ02(α+βh). Mass of the ball is m. Select the most appropriate one option.
View SolutionA small ball of density ρ is placed from the bottom of a tank in which a non viscous liquid of density 2ρ is filled . Find the time taken by the ball to reach the surface of liquid and maximum height reached above the bottom of container . (Size of the ball and any type of drag force acting on the ball are negligible )
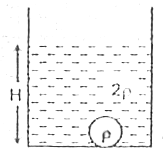
View SolutionA circular tube of uniform cross section is filled with two liquids densities ρ1 and ρ2 such that half of each liquid occupies a quarter to volume of the tube. If the line joining the free surfaces of the liquid makes an angle θ with horizontal find the value of θ.
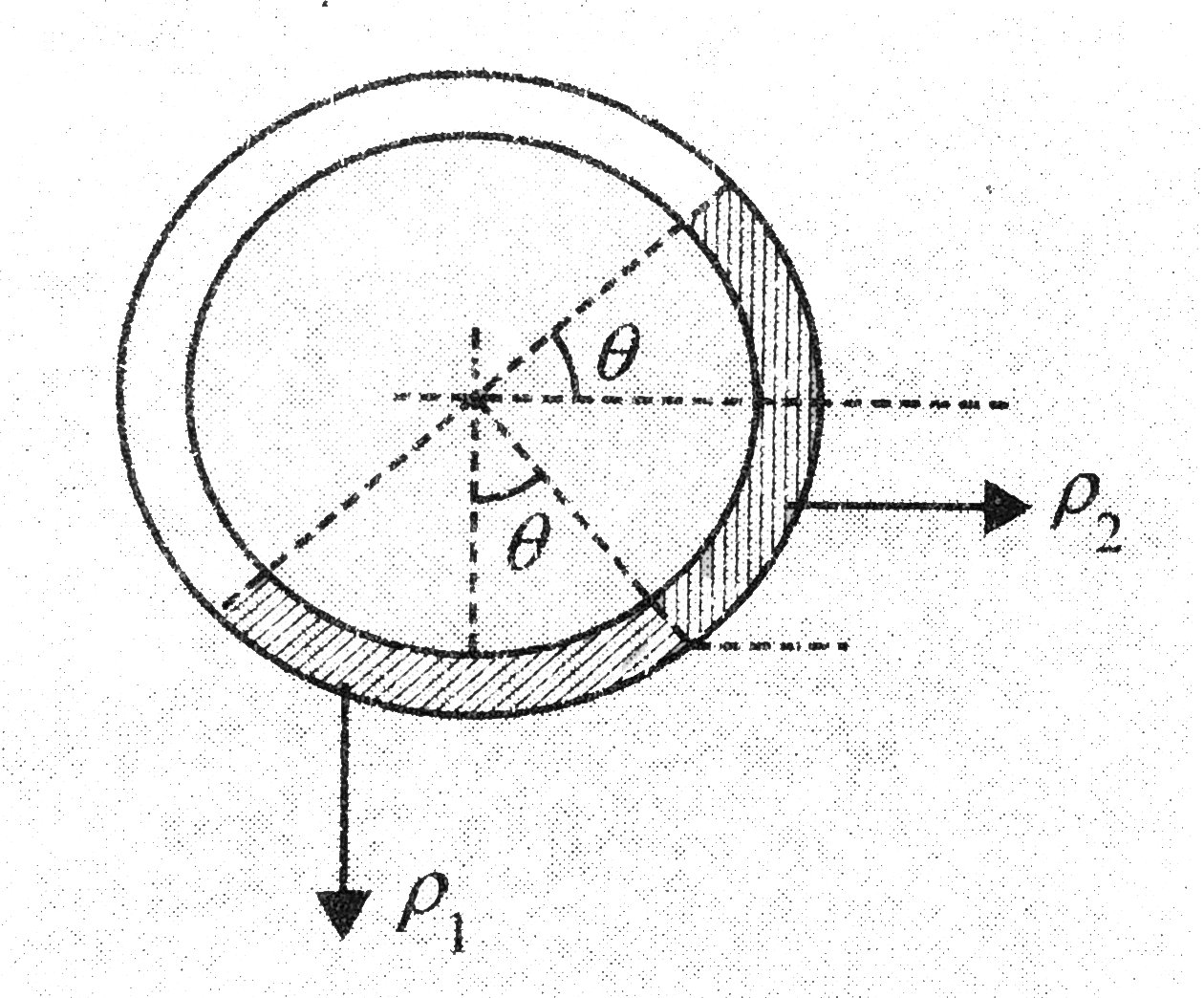
View SolutionIn the arrangement as shown, mB=3m, density of liquid is ρ and density of block B is 2ρ. The system is released from rest so that block B moves up when in liquid and moves down when out of liquid with the same acceleration. Find the mass of block A.
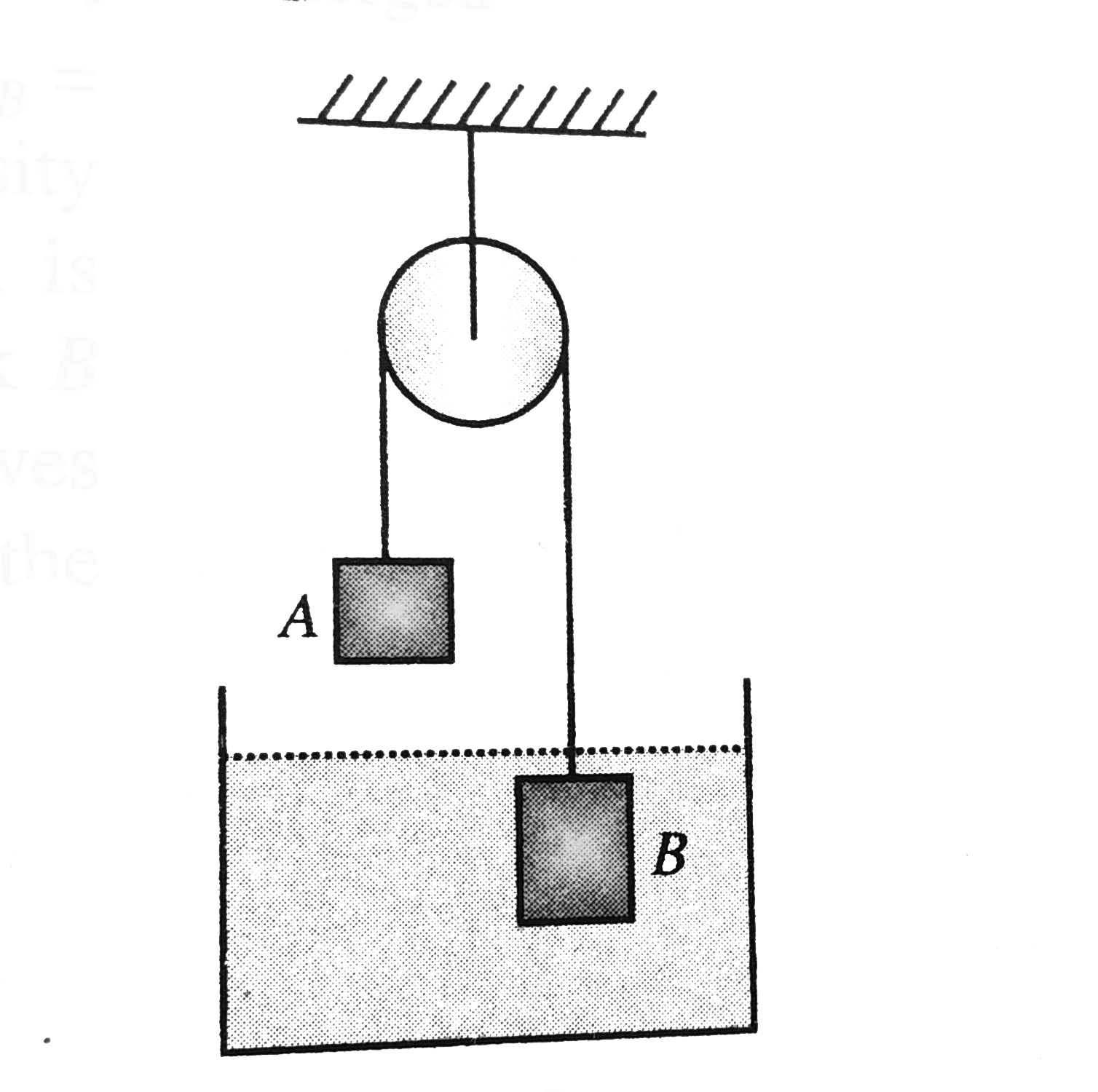
View SolutionA solid ball of density ρ1 and radius r falls vertically through a liquid of density ρ2. Assume that the viscous force acting on the ball is F=krv, where k is a constant and v its velocity. What is the terminal velocity of the ball ?
View Solution
