A liquid of density ρ is filled in a vessel up to height H and a hole of cross section area A is made at a depth h below the free surface of liquid. The speed of liquid coming out of the hole is independent of
h
a
ρ
g
The correct Answer is:C
To solve the problem, we need to determine the speed of the liquid coming out of the hole in the vessel and identify which quantity it is independent of.
1. Understand the Setup:
- We have a liquid of density ρ filled in a vessel to a height H.
- There is a hole with a cross-sectional area A at a depth h below the free surface of the liquid.
2. Apply Torricelli's Law:
- According to Torricelli's law, the speed v of the liquid flowing out of the hole can be derived from the principle of conservation of energy. The speed is given by:
v=√2gh
- Here, g is the acceleration due to gravity, and h is the vertical distance from the liquid surface to the hole.
3. Identify the Variables:
- The speed v depends on:
- g (acceleration due to gravity)
- h (depth of the hole)
- A (cross-sectional area of the hole)
- ρ (density of the liquid)
4. Analyze the Dependence:
- From the equation v=√2gh, we can see that the speed v is directly influenced by g and h.
- The cross-sectional area A of the hole does not appear in the equation for v when considering the speed derived from Torricelli's law.
5. Conclusion:
- The speed of the liquid coming out of the hole is independent of the density ρ of the liquid.
Final Answer:
The speed of the liquid coming out of the hole is independent of the density ρ.
- We have a liquid of density ρ filled in a vessel to a height H.
- There is a hole with a cross-sectional area A at a depth h below the free surface of the liquid.
- According to Torricelli's law, the speed v of the liquid flowing out of the hole can be derived from the principle of conservation of energy. The speed is given by:
v=√2gh
- Here, g is the acceleration due to gravity, and h is the vertical distance from the liquid surface to the hole.
- The speed v depends on:
- g (acceleration due to gravity)
- h (depth of the hole)
- A (cross-sectional area of the hole)
- ρ (density of the liquid)
- From the equation v=√2gh, we can see that the speed v is directly influenced by g and h.
- The cross-sectional area A of the hole does not appear in the equation for v when considering the speed derived from Torricelli's law.
- The speed of the liquid coming out of the hole is independent of the density ρ of the liquid.
The speed of the liquid coming out of the hole is independent of the density ρ.
Topper's Solved these Questions
- MECHANICAL PROPERTIES OF FLUIDSBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - MECHANICAL PROPERTIES OF FLUIDSEXERCISE - SECTION - C
15 Videos
- MECHANICAL PROPERTIES OF FLUIDSBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - MECHANICAL PROPERTIES OF FLUIDSEXERCISE - SECTION - D
2 Videos
- MECHANICAL PROPERTIES OF FLUIDSBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - MECHANICAL PROPERTIES OF FLUIDSEXERCISE - SECTION - A
50 Videos
- MAGNETISM AND MATTER BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - MAGNETISM AND MATTER EXERCISE - ASSIGNMENT (SECTION D)
26 Videos
- MECHANICAL PROPERTIES OF SOLIDSBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - MECHANICAL PROPERTIES OF SOLIDSEXERCISE - Assignment(Section-D)
14 Videos
Similar Questions
Knowledge Check
The pressure at depth h below the surface of a liquid of density ρ open to the atmosphere is
Agreater than the atmospheric pressure by ρghBless than the atmospheric pressure by ρghCequal to the atmospheric pressureDincreases exponentially with depthA cylinider is filled with non viscous liquid of density d to a height h0 and a hole is made at a height h1 from the bottom of the cylinder. The velocity issuing out of the hole is
A√2gh0B√2g(h0−h1)C√dgh1D√dgh0
A liquid is filled in a vessel and a hole is made at a depth h below the free surface of the liquid.
Statement-1 : Greater is the distance of the hole form the free surface of liquid, greater will be the velocity of efflux.
And
Statement-2 The speed of the liquid coming out of the orifice depends on the quantity of liquid in the vessel.
View SolutionA cylindrical vessel is filled with a liquid up to a height H. A small hole is made in the vessel at a distance y below the liquid surface as shown in figure. The liquid emerging from the hole strike the ground at distance x
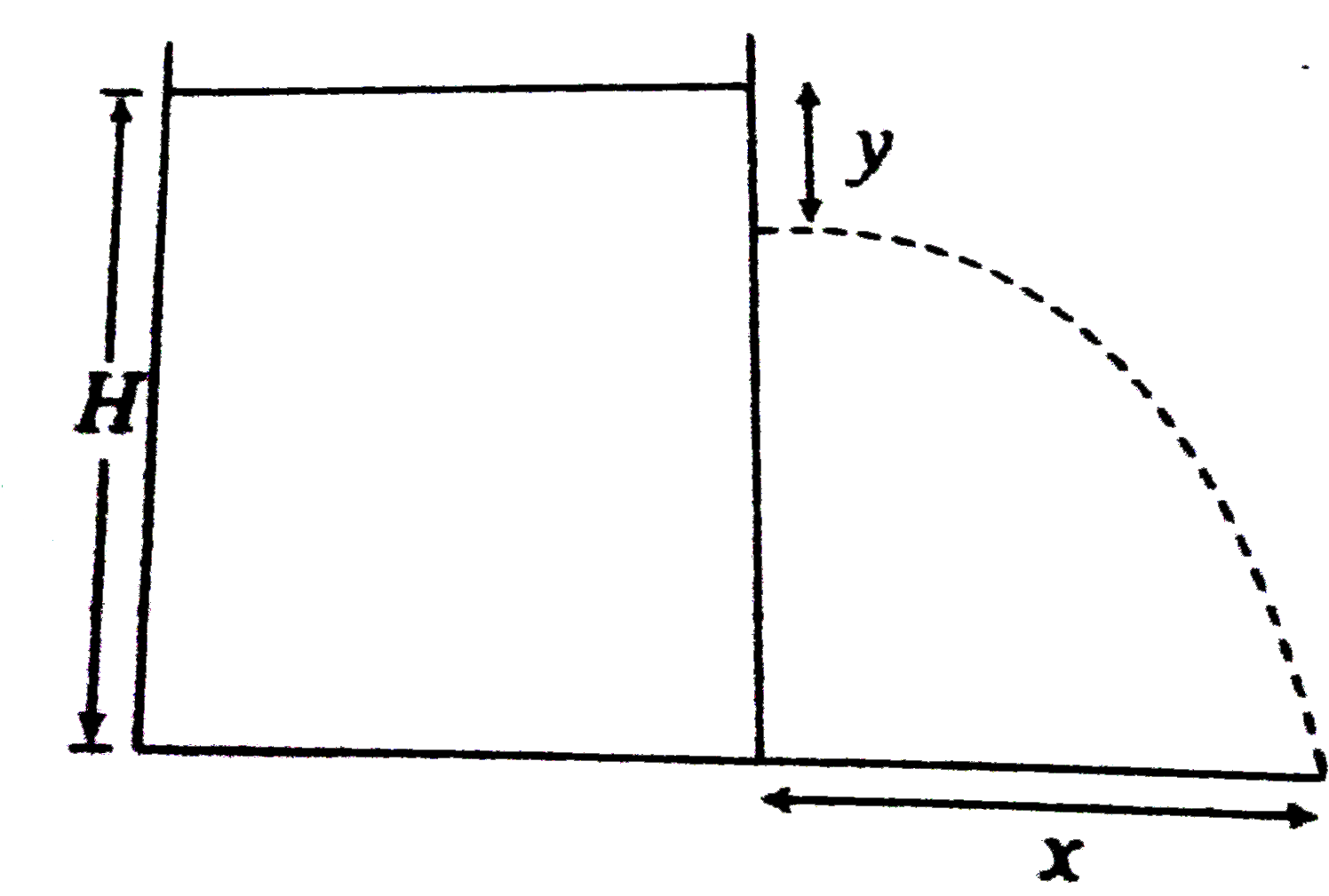
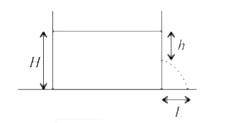
View SolutionA liquid is filled in a container to a height H. A small hole is opened in the side of the container a height h below the surface of the liquid. The distance L from the bottom of the container of the point where the stream of liquid leaving the container meets the ground is :
View SolutionA non viscous liquid of density ρ is filled in a tube with A as the area of cross section, as shown in the figure. If the liquid is slightly depressed in one of the arms, the liquid column oscillates with a frequency
View SolutionA cylindrical vessel contains a liquid of density ρ up to height h. The liquid is closed by a piston of mass m and area of cross section A. There is a small hole at the bottom of the vessel. The speed v with which the liquid comes out of the hole is

View SolutionA cylindrical vessel contains a liquid of density ρ up to height h. The liquid is closed by a piston of mass m and area of cross section A. There is a small hole at the bottom of the vessel. The speed v with which the liquid comes out of the hole is

View Solution
