Derive the expression for the radius of the orbit of the electron and its velocity using Bohr atom model. Solution in Tamil

Video Solution

Text Solution

Verified by Experts

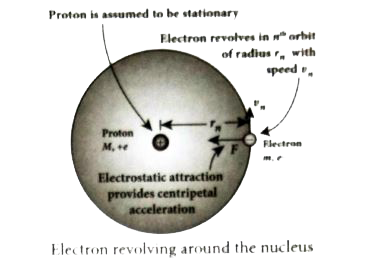
Radius of the orbit of the electron and velocity of the electron : Consider an atom which contains the nucleus at rest and an electron revolving around the nucleus in a circular orbit of radius $r_{n}$ as shown in figure. Nucleus is made up of protons and neutrons. Since proton is positively charged and neutron is electrically neutral, the charge of a nucleus is purely the total charge of protons.
Let Z be the atomic number of the atom, then +Ze is the charge of the nucleus. Let -e be the charge of the electron. From Coulomb.s law, the force of attraction between the nucleus and the electron is
${\to F}_{coloumb} = \frac{1}{4 π ε_{0}} \frac{(+ Z e) (e)}{r_{n}^{2}} ˆ r$
$= - \frac{1}{4 π ε_{0}} \frac{Z e^{2}}{r_{n}^{2}} ˆ r$
This force provides necessary centripetal force
${\to F}_{centripetal} = \frac{m v_{n}^{2}}{r_{n}} ˆ r$
where m be the mass of the electron that moves with a velocity $v_{n}$ in a circular orbit. Therefore ,
$∣ ∣ {\to F}_{coloumb} ∣ ∣ = ∣ ∣ {\to F}_{centripetal} ∣ ∣$
$\frac{1}{4 π ε_{0}} \frac{Z e^{2}}{r_{n}^{2}} = \frac{m v_{n}^{2}}{r_{n}}$
$r_{n} = \frac{4 π ε_{0} (m v_{n} r_{n}) 2}{Z m e^{2}}$ ...(1)
From Bohr.s assumption, the angular momentum quantization condition, $m v_{n} r_{n} = I_{n} = n_{h}$ .
$∴ r_{n} = \frac{4 π ε_{0} {(m v_{n} r_{n})}_{n}^{2}}{Z m e^{2}}$
$r_{n} = \frac{4 π ε_{0} {(n h)}^{2}}{Z m e^{2}} = \frac{4 π ε_{0} n^{2} h^{2}}{Z m e^{2}}$
$r_{n} = (\frac{ε_{0} h^{2}}{π m e^{2}}) \frac{n^{2}}{Z} (∴ h = \frac{h}{2 π})$ ...(2)
where n $\in N$ . Since $ε_{0}, h, e$ and $π$ are constants. Therefore , the radius of the orbit becomes
$r_{n} = a_{0} \frac{n^{2}}{Z}$
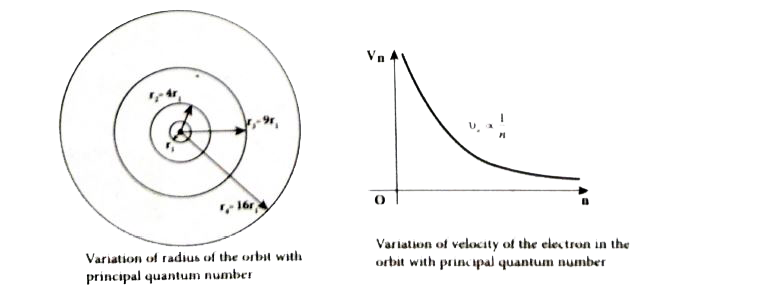
where $a_{0} = \frac{ε_{0} h^{2}}{π m e^{2}}$ =0.529 Å. This is known as Bohr radius which is the smallest radius of the orbit in an atom . Bohr radius is also used as unit of length called Bohr. 1Bohr =0.53 Å.For hydrogen atom (Z=1) , the radius of $n^{t h}$ orbit is
$r_{n} = a_{0} n^{2}$
For the first orbit (ground state ),
$r_{1} = a_{0}$ =0.529 Å
For the second orbit (first excited state),
$r_{2} = 4 a_{0}$ =2.116 Å
For the third orbit (second excited state) ,
$r_{3} = 9 a_{0}$ =4.761 Å and so on.
Thus the radius of the orbit from centre increases with n, this is $r_{n} \propto n^{2}$ as shown in figure . Further, Bohr.s angular momentum quantization condition leads to
$m v_{n} r_{n} = m v_{n} a_{0} n^{2} = n \frac{h}{2 π}$
$v_{n} = \frac{h}{2 π m a_{0}} \frac{Z}{n}$
$v_{n} \propto \frac{1}{n}$
Note that the velocity of electron decreases as the principal quantum number increases as shown in figure. This curve is the rectangular hyperbola. This implies that the velocity of electron in ground state is maximum when compared to excited states.

Updated on:21/07/2023

|

Class 12 PHYSICS SAMPLE PAPER-1 (SOLVED)

Topper's Solved these Questions

Similar Questions

FULL MARKS-SAMPLE PAPER-1 (SOLVED)-Questions

Home
Ask Doubt
Profile