Obtain the expression for electric field due to an uniformly charge spherical shell. Solution in Tamil
Electric field due to a uniformly charged spherical shell: Consider a uniformly charged spherical shell of radius R and total charge Q. The electric field at points outside and inside the sphere is found using Gauss law.
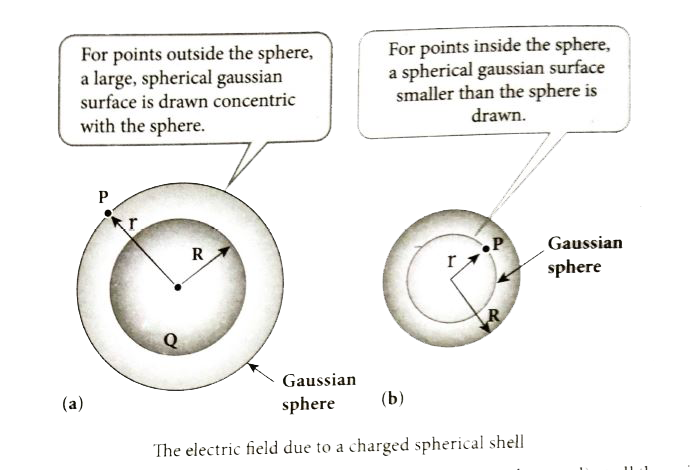
Case (a) At a point outside the shell (r> R): Let us choose a point P outside the shell at a distance r from the center as shown in figure (a). The charge is uniformly distributed on the surface of the sphere (spherical symmetry). Hence the electric field must point radially outward if Q> 0 and point radially inward if Q < 0. So we choose a spherical Gaussian surface of radius r and the total charge enclosed by this Gaussian surface is Q. Applying Gauss law,
∮→E.d→A=Qε0 ...(1)
The electric field →E and d→A point in the same direction (outward normal) at all the points on the Gaussian surface. The magnitude of →E is also the same at all points due to the spherical symmetry of the charge distribution
Hence E∮dAGaussian surface=Qε0...(2)
But ∮dAGaussian surface =total area of Gaussian surface =4πr2.
Substituting this value in equation (2).
E.4πr2=Qε0
E.4πr2=Qε0 (or) E=14πε0Qr2
In vector form →E=14πε0Qr2ˆr ...(3)
The electric field is radially outward if Q>0 and radially inward if Q<0. From equation (3) we infer that the electric field at a point outside the shell will be same as if the entire charge Q is concentrated at the center of the spherical shell. (A similar result is observed in gravitation, for gravitational force due to a spherical shell with mass M)
Case (b): At a point on the surface of the spherical shell (r= R): The electrical field a points on the spherical shell (r=R) is given by
→E=Q4πε0R2ˆr ...(4)
Case (c) At a point inside the spherical shell (r< R): Consider a point P inside the shell at a distance r from the center. A Gaussian sphere of radius r is constructed as shown in the figure (b). Applying Gauss law
∮→E.d→AGaussian surface=Qε0
E.4πr2=Qε0 ...(5)
Since Gaussian surface encloses no charge , So Q=0 . The equation (5) becomes
E=0 (r < R) ...(6)
The electric field due to the uniformly charged spherical shell is zero at all points inside the shell.
Topper's Solved these Questions
- SAMPLE PAPER-1 (SOLVED)BOOK - FULL MARKSCHAPTER - SAMPLE PAPER-1 (SOLVED)EXERCISE - Questions
28 Videos
- SAMPLE PAPER-07 (SOLVED )BOOK - FULL MARKSCHAPTER - SAMPLE PAPER-07 (SOLVED )EXERCISE - (PART - IV)(Answer all the questions.)
10 Videos
- SAMPLE PAPER-16 (UNSOLVED)BOOK - FULL MARKSCHAPTER - SAMPLE PAPER-16 (UNSOLVED)EXERCISE - PART-IV
10 Videos
Similar Questions
What is meant by Fraunhofer lines?
View SolutionCalculate the electric field due to a dipole on its equatorial plane. (OR) Electric field due to an electric dipole at a point on the equatorial plane
View SolutionDerive an expression for electrostatic potential energy of the dipole in a uniform electric field .
View SolutionObtain the expression for electric field due to an uniformly charge spherical shell.
View SolutionDerive an expression for electric intensity E due to a uniformly charged thin spherical shell at a point outside the shell.
View SolutionObtain the expression for electric field due to an uniformly charge spherical shell.
View SolutionObtain the expression for electric field due to an uniformly charge spherical shell.
View SolutionFind expression for electric field intensity due to a uniformly charged thin spherical shell at a point outside the shell.
View SolutionObtain the expression of electric field by thir spherical shell with uniform charge distribution at a point outside it.
View SolutionState Gauss's theorem with the help of diagram, derive an expression for the electric field intensity due to uniformly charged thin spherical shell at a point on the surface of the spherical shell.
View SolutionObtain an expression for electric field intensity due to uniformly charged spherical shell or hollow sphere.
View SolutionDerive an expression for electric field due to a uniformly charged spherical shell. i) at a point outside the shell
View SolutionDeduce the expression of field at P due to a uniformly charged thin spherical shell when the point,P is inside the shell.
View SolutionDerive the expression for electric field intensity due to a uniformly charged thin spherical shell at a point outside the shell
View SolutionDerive the expression for electric field intensity due to a uniformly charged thin spherical shell
at a point inside the shell.
View Solution