Describe Young's double slit experiment to produce interference pattern due to a monochromatic source of light. Deduce the expression for the fringe width.

Video Solution

Text Solution

Verified by Experts

Simplest experiment to show interference of light is Young's double slit experiment : $δ$ is a narrow slit (of width about 1 mn) illuminated by a monochromatic source of light. At a suitable distance ( $\approx 10 m m$ ) form $δ, two slits S_{1} and S_{2}$ are placed parallel to S. When a screen is placed at a large distance (about 2m) from the slit $S_{1} and S_{2}$ , alternate dark and bright bands appear on the screen. These are the interference bands or fringas. The bond disappear when either slit is covered.
Explanation : According to Huygen's pricipal, the monochromatic source of light illuminating the slit S tends out spherical wavefront.
The two waves of same amplitude and same freqency superimpose on each other.
Dark fringes appear on the screen when the crest of one wave falls on the trough of other and they neutralise the effect of each other.
Bright fringes appear on the screen when the crest of one wave coincides with the crest of other and they reinforce each other.
Expression for the fringe width :
Let d= distance between slits $S_{1} and S_{2}$
D= distance of screen from two slits
and x = distance between the central maxima O and observation point P.

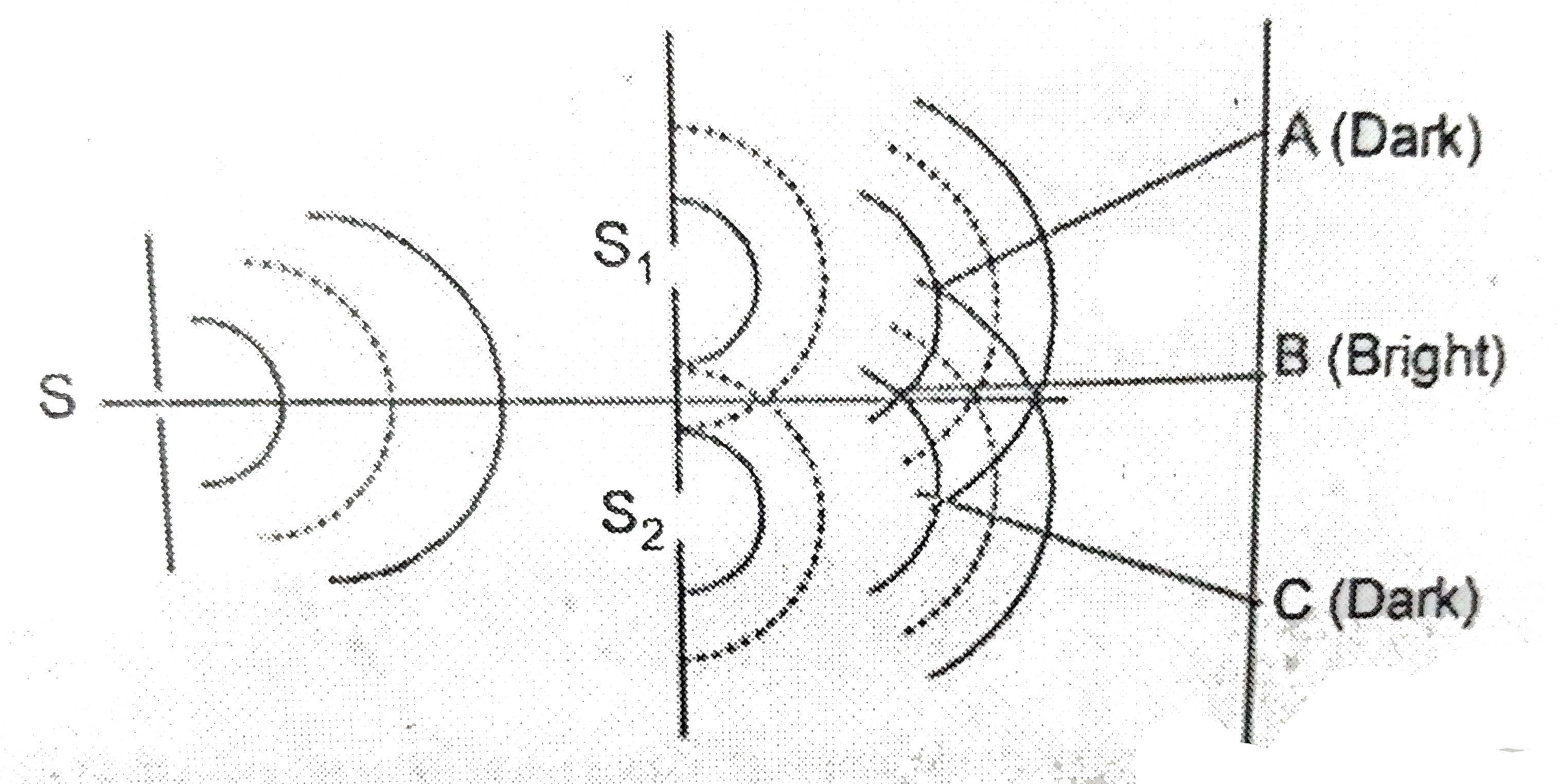
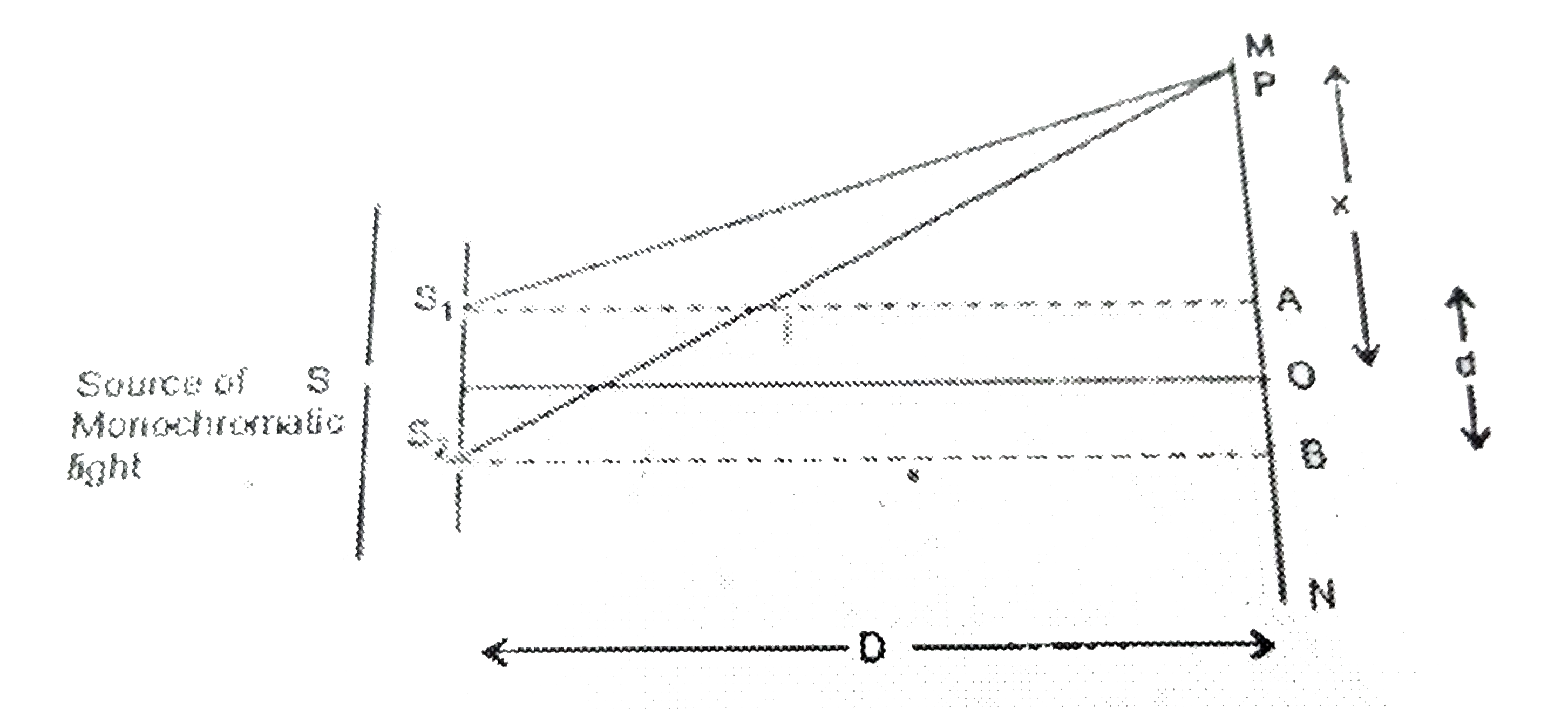
Light waves spread out from S and fall on both $S_{1} and S_{2}$ . The spherical waves emanating from $S_{1} and S_{2}$ will produce interference frings on the screen MN.
In right $Δ S_{1} A P$ , we have,
${(S_{1} P)}_{1}^{2} = {(S_{1} A)}_{1}^{2} + {(A P)}^{2}$
$S_{1} P = \sqrt{D^{2} + {(x - \frac{d}{2})}^{2}} = \sqrt{D^{2} ⎡ ⎢ ⎢ ⎢ ⎣ 1 + \frac{{(x - \frac{d}{2})}^{2}}{D^{2}} ⎤ ⎥ ⎥ ⎥ ⎦}$
$S_{1} P = D ⎡ ⎢ ⎢ ⎢ ⎣ 1 + \frac{{(x - \frac{d}{2})}^{2}}{D^{2}} ⎤ ⎥ ⎥ ⎥ ⎦ = D + \frac{{(x - \frac{d}{2})}^{2}}{2 D}$
Similarly $S_{2} P = D + \frac{{(x + \frac{d}{2})}^{2}}{2 D}$
Hence, path difference $= S_{2} P - S_{1} P$
$= D + \frac{{(x + \frac{d}{2})}^{2}}{2 D} - D - \frac{{(x - \frac{d}{2})}^{2}}{2 D} = \frac{1}{2 D} [x^{2} + \frac{d^{2}}{4} + x d - x^{2} - \frac{d^{2}}{4} + x d]$
$= \frac{1}{2 D} .2 x d = \frac{x d}{D}$ .
Now the intesity at point P is maximum or minimum according as the path difference is an integral multiple of wavelength or an odd intefral multiple of half wavelength.
(i) For Brigth fringe (constructive interference) : We will have constructive interference resulting in a bright fringe when path difference $= n λ$
$\frac{x d}{D} = n λ \Rightarrow x = \frac{n λ D}{d}$
$∴_{n} = \frac{n λ D}{d}$
where, $n = 0 \pm 1 \pm 2 ...$
Since, the separation between the centres of two consecutive bright fringes is called fringe width. It is denoted by $β$
$∴ Fringe width, β = x_{n + 1} - x_{n}$ .
$β = \frac{(m + 1) λ D}{d} \frac{n λ D}{d} = \frac{λ D}{d} (n + 1 - n)$
$∴ β = \frac{λ D}{d}$
(ii) For Dark fringe (destructive interference): We will have destructive interference resulting in a dark fringe when path difference
$= (2 n + 1) \frac{λ}{2}$
$\frac{x d}{D} = (2 n + 1) \frac{λ}{2} \Rightarrow x = \frac{(2 n + 1) λ D}{2 d}$
$∴ x_{n} = \frac{(2 n + 1) λ D}{2 d}$ ,
where, $n = 0, \pm 1, \pm 2, ...$
Fringe width $β = x_{n + 1} - x_{n}$
$= \frac{[2 (n + 1) + 1] λ D}{2 d} - \frac{(2 n + 1) λ D}{2 d} = (2 n + 2 + 1 - 2 n - 1) \frac{λ D}{2 d} = \frac{2 λ D}{2 d}$
$∴ β = \frac{λ D}{d}$
Hence, all bright and dark fringes are of equal width.
Observations :
(i) Fringe width is directly proportional to the wavalength of light i.e. $β α λ$ .
(ii) Fringe width is inversely proportional to the distance between screen and two sourves i.e. $β α \frac{1}{d}$ .
(iii) Fringe width is directly proportional to the distance between screen and two sources i.e. $β α D$

Updated on:21/07/2023

Yellow light of wavelength 6000 Ã… produces fringes of width 0.8 mm in Young's double slit experiment. If the source is replaced by another monochromatic source of wavelength 7500 Ã… and the separation between the slits is doubled then the fringe width becomes
A0.1 mm
B0.5 mm
C4.3 mm
D1 mm
In a Young's double slit experiment an electron beam is used to obtain interference pattern. If the speed of electron decreases then
Adistance between two consecutive fringes remains the same
Bdistance between two consecutive fringes decreases
Cdistance between two consecutive fringes increases
DNone of these.
A Young's double slit experiment uses a monochromatic source. The shape of the interference fringes formed on a screen is
Aparabola
Bstraight line
Ccircle
Dhyperbola