Distance between the centres of two stars is 10a. The masses of these stars are M and 16M and their radii a and 2a respectively. A body of mass m is fired straight from the surface of the larger star towards the surface of the smaller star. What should be its minimum initial speed to reach the surface of the smaller star? Obtain the expression in terms of G, M and a.
The correct Answer is:A, B, C
Let there are two stars 1 and 2 as shows below.
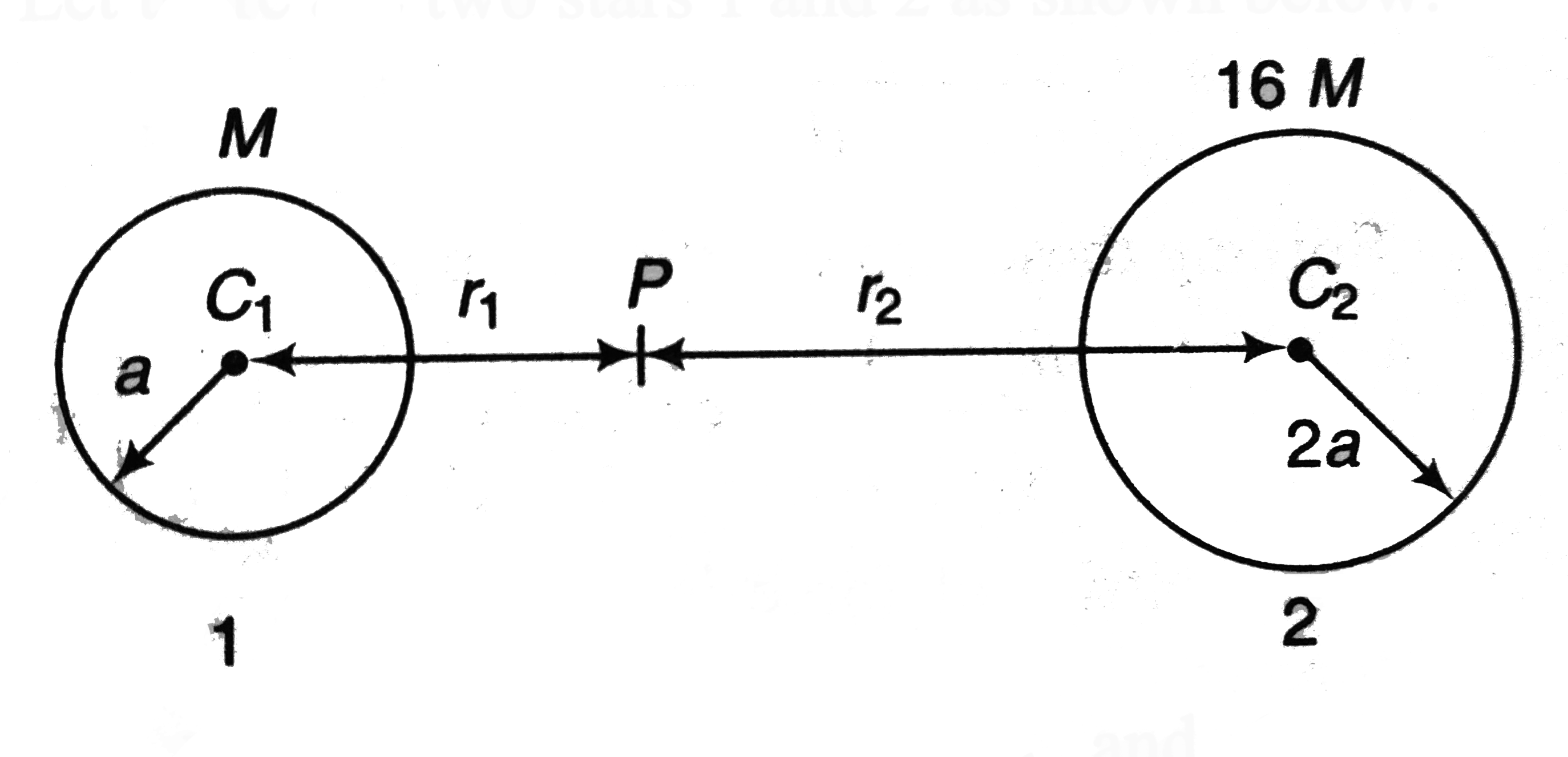
Let P is a point between C1 and C2, where gravitational field strength is zero. Or at P field stregth due to star 1 is equal and opposite to the field stength due to star 2. Hence,
GMr21=G(16M)r22 or r2r1=4
also r1+r2=10a
∴r2(44+1)(10a)=8a
and r1=2a
NOw, the body of mass m is projected from the surface of larger star to wards the smaller one. Between C2 and P it is attracted towards 2 and between C1 and p it will be attracted towards 1. point P becouse beyond that the particle is attracted towards the smallar star itself.
From conservation of mechanical energy12mν2min
= Potential energy of body at P
−Potential energy at the surface of the larger star.
∴12mν2min=[−GMmr1−16GMmr2]
−[−GMm10a−2a−16GMm2a]
=[−GMm2a−16GMm8a]−[−GMm8a−16GMma]
or 12mν2min=(848)GMma
∴νmin=3√52(√GMa)
Topper's Solved these Questions
- BOOK - DC PANDEYCHAPTER - GRAVITATIONEXERCISE - Check Point 10.120videos
GRAVITATION
- BOOK - DC PANDEYCHAPTER - GRAVITATIONEXERCISE - Check Point 10.220videos
GRAVITATION
- BOOK - DC PANDEYCHAPTER - GRAVITATIONEXERCISE - Level 2 More Than One Correct10videos
GRAVITATION
- BOOK - DC PANDEYCHAPTER - GENERAL PHYSICSEXERCISE - INTEGER_TYPE2videos
GENERAL PHYSICS
- BOOK - DC PANDEYCHAPTER - KINEMATICSEXERCISE - INTEGER_TYPE11videos
KINEMATICS
Similar Questions
Distance between the centres of two stars is 10a. The masses of these stars are M and 16M ans their radit a nd 2a respectively. A body of mass m is fired straight from the surface of the larger star tpwards the surface of the smaller star. What should be its minimum intial speed to reach the surface of the smaller star? Obtain the expression in terms of G, M and a.
View SolutionThe distance between the centres of two stars is 10α. The masses of these stars are M and 16M and their radii α and 2α. A body of mass m is fired straight from the surface of the larger star towards the smaller star. What should be its minimum initial speed to reach the surface of teh smaller star? Obtain the expression interms of G, M and α.
View Solution
Knowledge Check
- Question 1 - Select One
Distance between the centres of two stars is 10a. The masses of these stars are M and 16M and their radii a and 2a respectively. A body of mass m is fired straight from the surface of the larger star towards the smaller star. The minimum initial speed for the body to reach the surface of smaller star is
A23√GmaB32√5GmaC23√5GmaD32√Gma - Question 2 - Select One
Masses of stars and galaxies are usually expressed in terms of :
Aneutron massBearth 's massCsolar massDlunar mass - Question 3 - Select One
Light from distant star will be reaching on earth's surface in the form of
Aspherical wavefrontBplane wavefrontCcylindrical wavefrontDall of these
Two stars of masses M and 4M have radii x and 3x respectively. The distance between centres of two stars is 12x. A body of mass m is fired from the surface of bigger sphere towards smaller sphere. Find the minimum initial speed (in terms of G, x, M) required to reach the surface of smaller star.
View SolutionTwo planets have masses M and 16 M and their radii are a and 2a, respectively. The separation between the centres of the planets is 10a. A body of mass m is fired trom the surface of the larger planet towards the samller planet along the line joining their centres. For the body to be able to reach at the surface of smaller planet, the minimum firing speed needed is :
View SolutionA shooting star is a star.
View Solution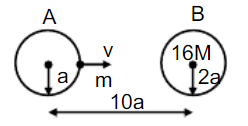
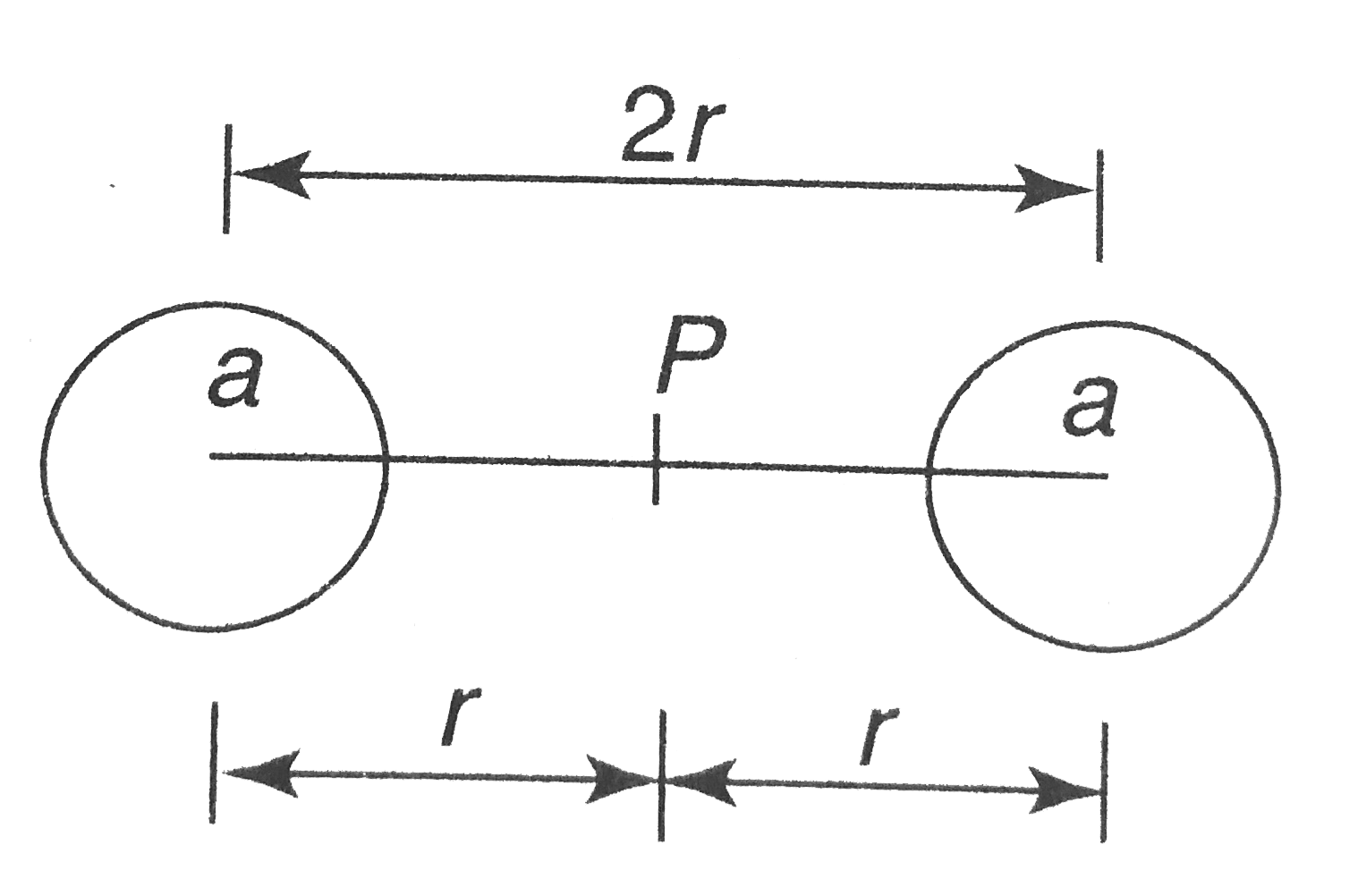
Two planets of mass M and 16M of radius a and 2a respectively, are at distance 10a. Find minimum speed of a particle of mass m at surface of smaller planet so that it can reached from smaller planet to larger planet.View SolutionA particle is projected from the surface of one star towards other star of same radius a and mass with such a minimum velocity K√GMa, so that it is attracted towards other star. Find the value of K if two stars are 2r distance apart:
 View Solution
View Solution

