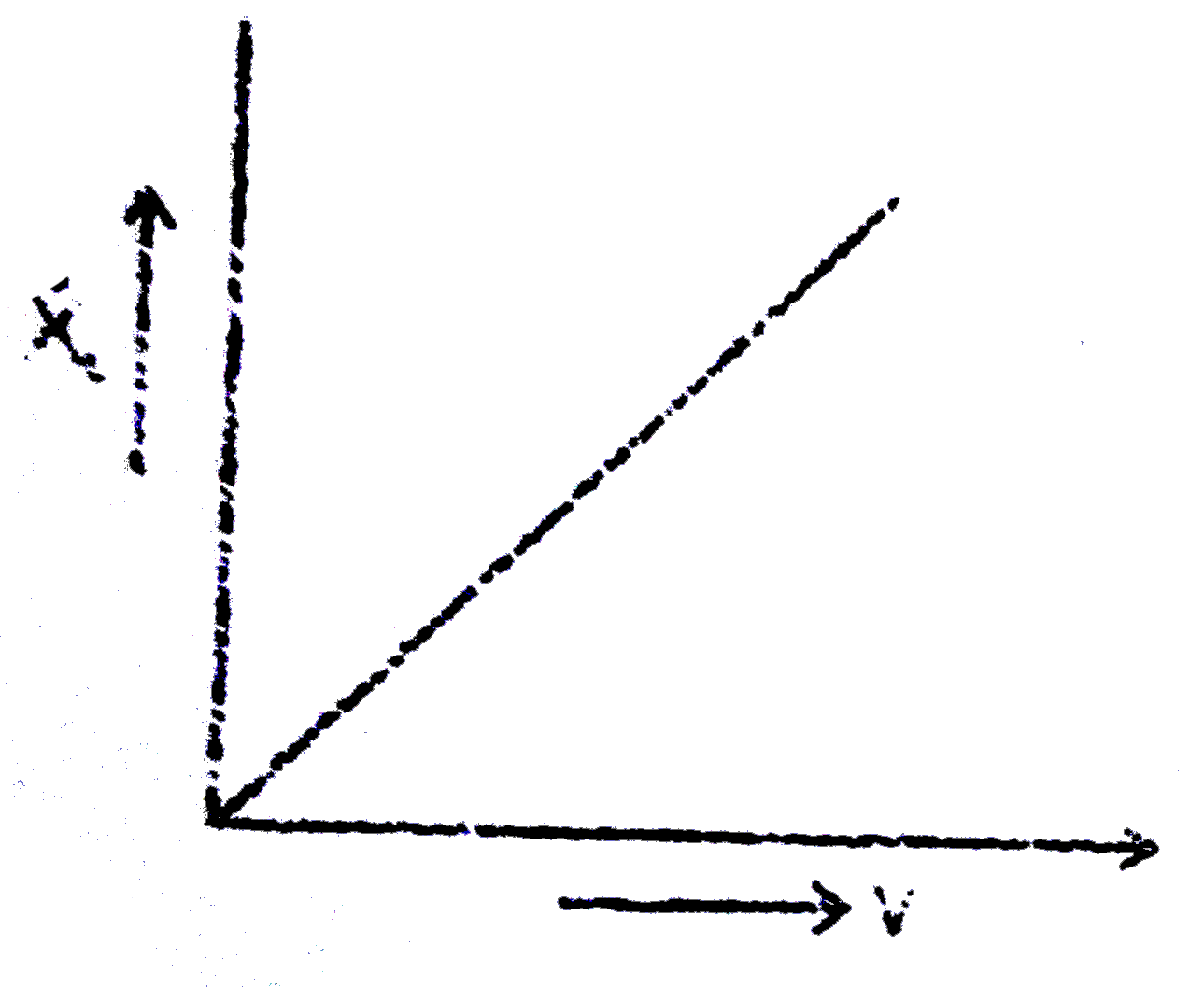
Explain the term 'inductive reactance'.Show graphically the variation of inductive reactance with frequency of the applied alternating voltage.
An a.c. voltage E=E0sinωt is applied across a pure inductor L.Show mathematically that the current flowing through it lags behind the applied voltage by a phase angle of π/2.
Inductive reactance :The effective resistance offered by inductance L is called inductive reactance.It is denoted by XL.it SI unit is ohm.The graph between frequency (v) and XL is shown in fig.
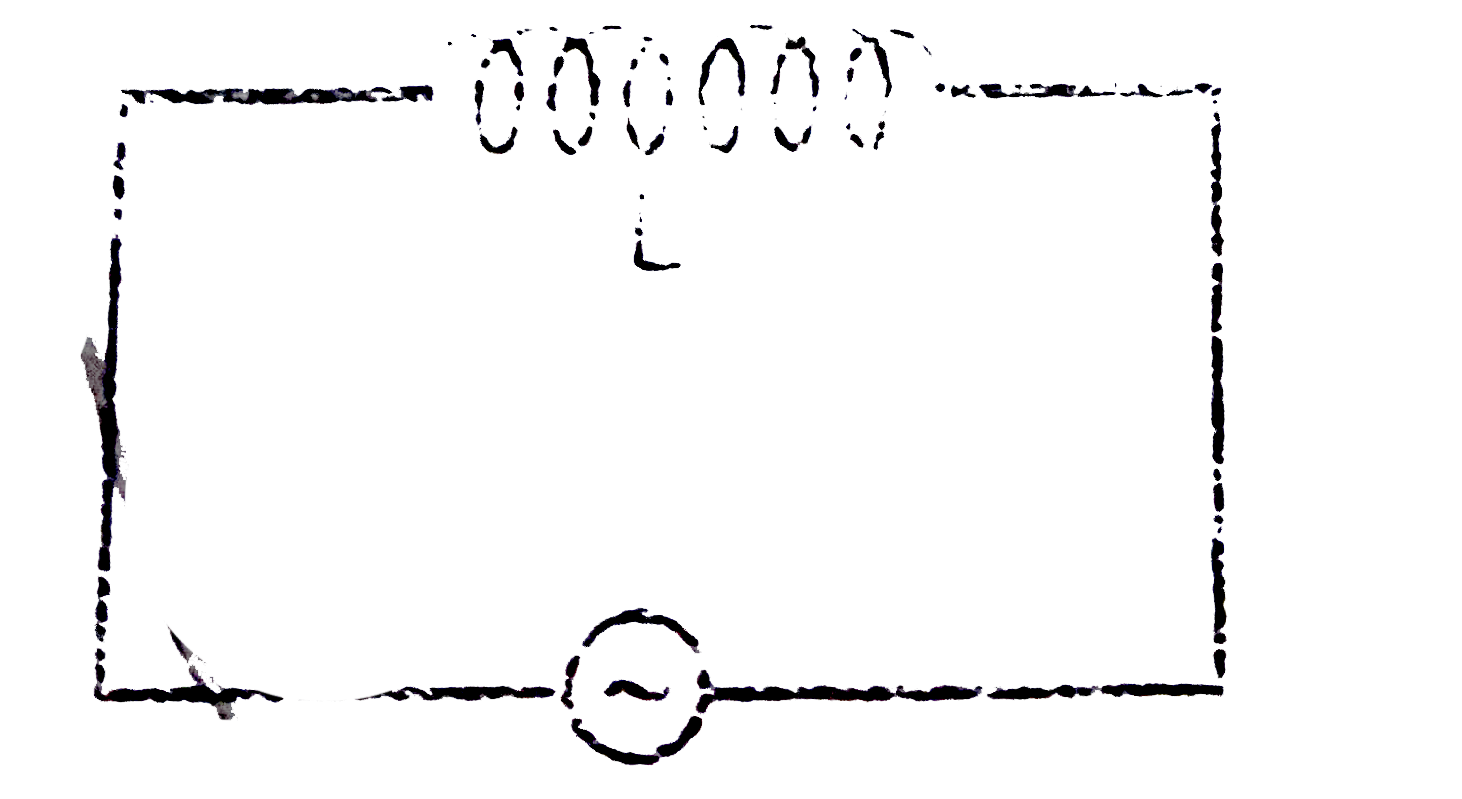
A.C. Source containing an inductor: Let a source of alternating e.m.f E=E0sinωt be connected to an inductor of inductance L only.
If i be the current through the circuit, then
instanteous induced e.m.f produced V=Ldidt
di=VLdt
di=VmLsinωtdt,[∵V=Vmsinωt]
Integrating both sides, we get
∫di=VmL∫sinωtdt
i=VmL(−cosωtω)=−VmωLcosωt
i=−VmωLsin(π2−ωt)
=−VmωLsin(ωt−π2)
i=imsin(ωt−π2)...(i)
where i=VmωL is the amplitude of the current.The quanity ωL is called the inductive reactance and is denoted by XL i.e., XLωL
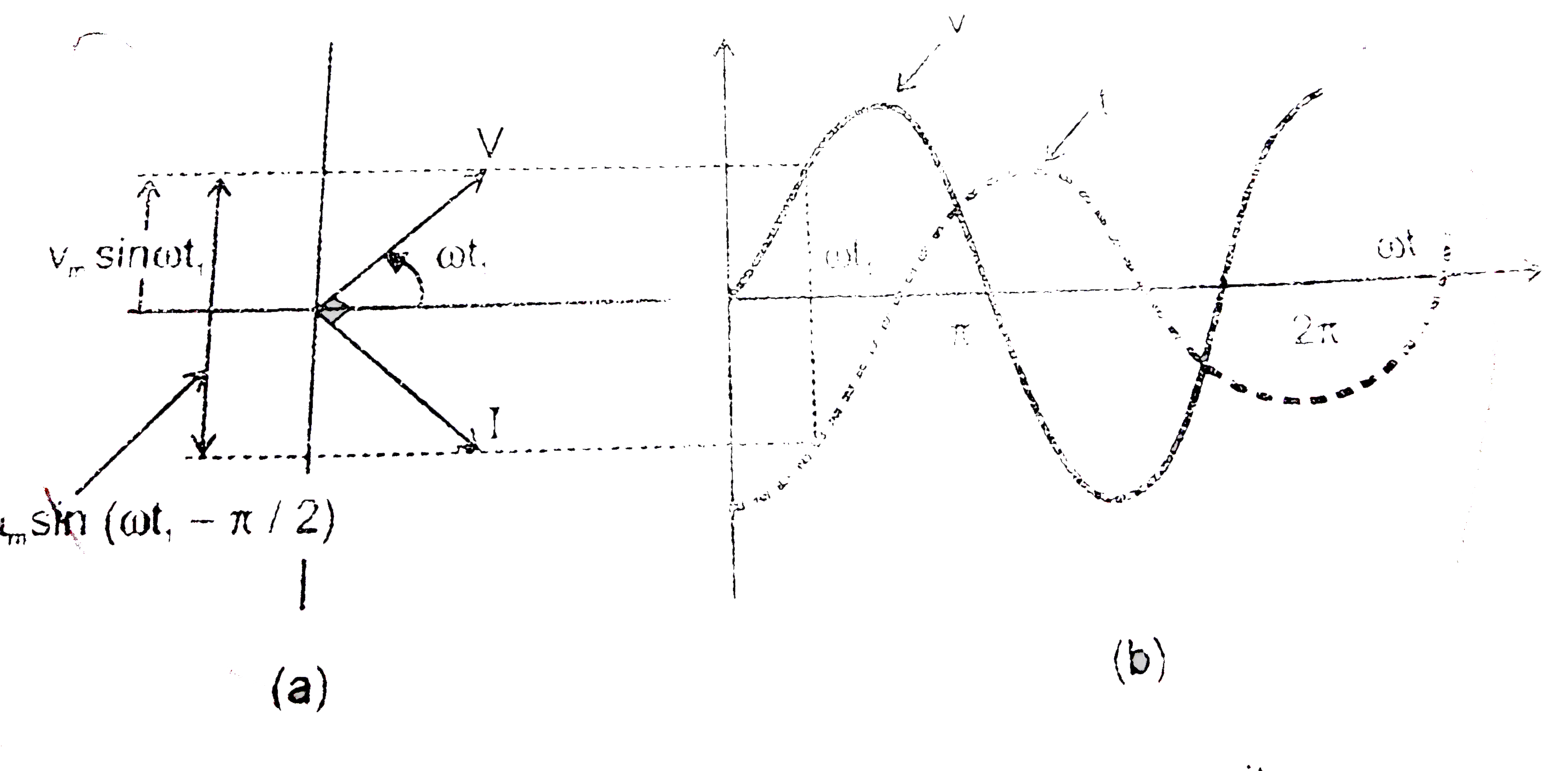
The equation (i) shows that the current logs the voltage by π2
(or e.m.f lends the current by a phase angle π2)
The phasor diagram for the circuit between →V and →i at any istant t is shown in Fig. (a)and the graph of V and i versus ωt is shown in Fig. (b)
Topper's Solved these Questions
- ALTERNATING CURRENTBOOK - RESONANCECHAPTER - ALTERNATING CURRENTEXERCISE - A.L.P
10 Videos
- ALTERNATING CURRENTBOOK - RESONANCECHAPTER - ALTERNATING CURRENTEXERCISE - SOLVED MISCELLANEOUS
14 Videos
- ALTERNATING CURRENTBOOK - RESONANCECHAPTER - ALTERNATING CURRENTEXERCISE - Exercise -3 Part-2
3 Videos
- ATOMIC PHYSICSBOOK - RESONANCECHAPTER - ATOMIC PHYSICSEXERCISE - Advanved level problems
17 Videos
Similar Questions
Explain the term 'capacitive reactance'.Show graphically the variation of capacitive reactance with frequency of the applied alternating voltage.
An a.c. voltage E=E0sinωt is applied a across a pure capacitor C.Show mathematically that the current flowing through it leads the applied voltage by a phase angle of π/2
View SolutionAn voltage, E=E0sinωt is applied acorss an inductor L. Obtain an expression for the current.
View Solution
Knowledge Check
An alternating voltage E=E0sinωt , is applied across a coil of inductor L. The current flowing through the circuit at any instant is
AE0ωLsin(ωt+π/2)BE0ωLsin(ωt−π/2)CE0ωLsin(ωt−π/2)DE0ωLsin(ωt+π/2)A small signal voltage V(t)=V0sinωt is applied across an ideal capacitor C:
ACurrent l(t) leads voltage V(t) by 180∘BCurrent l(t) lags voltage V(t) by 90∘COver a full cycle the capacitor C does not consume any energy from the voltage sourceDCurrent l(t) is in phase with voltage V(t)A small signal voltage V(t)=V0sinωt is applied across an ideal capacitor C:
ACurrent I(t), lags voltage V(t) by 90∘BOver a full cycle the capacitor C does not consume any energy from the voltage source.CCurrent I(t) is in phase with voltage V(t)DCurrent I(t) leads voltage V(t) by 180∘
Sketch a graph showing the variation of reactance of an inductor with frequency of the applied voltage
View SolutionAn a.c. voltage V=Vm sin ot is applied across an inductor L. Obtain an expression for current I.
View SolutionIf an alternating voltage V=V_0 sin omegat is applied across an inductance I, the current through the inductance will be
View SolutionIf an alternating voltage V=V_0 sin omegat is applied across an inductance I, the current through the inductance will be
View SolutionA sinusoidal voltage V(t) = 100 sin (500t) is applied across a pure inductance of L = 0.02 H. the current through the coil is
View Solution
