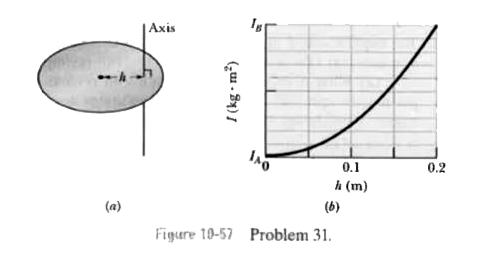
Figure 10-57 a shows a disk that can rotate about an axis at a radial distance h from the center of the disk. Figure 10-57b gives the rotational inertia I of the disk about the axis as a function of that distance h, from the center out to the edge of the disk. The scale on the I axis is set by $I_{A} = 0.050 k g \cdot m^{2} and I_{B} = 0.150 k g \cdot m^{2}$ . What is the mass of the disk?

Video Solution

Text Solution

Verified by Experts

2.5 kg

|

Updated on:21/07/2023

Class 12 PHYSICS RIGID BODY DYNAMICS

Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One
A uniform disc of mass 2kg is rotated about an axis perpendicular to the plane of the disc . If radius of gyration is 50cm, then the M.I. of disc about same axis is
A $0.25 k g m^{2}$
B $0.5 k g m^{2}$
C $2 k g m^{2}$
D $1 k g m^{2}$
Question 2 - Select One
A uniform disk of mass $300 k g$ is rotating freely about a vertical axis through its centre with constant angular velocity $ω .$ A boy of mass $30 k g$ starts from the centre and moves along a radius to the edge of the disk. The angular velocity of the disk now is
A $\frac{ω_{0}}{6}$
B $\frac{ω_{0}}{5}$
C $\frac{4 ω_{0}}{5}$
D $\frac{5 ω_{0}}{6}$
Question 3 - Select One
The moment of inertia of cylinder of radius a, mass M and height h about an axis parallel to the axis of the cylinder and distance b from its centre is :
A $\frac{1}{2} M (a^{2} + 2 b^{2})$
B $\frac{1}{2} M (2 a^{2} + b^{2})$
C $\frac{1}{2} M (a^{2} + b^{2})$
D $\frac{1}{2} M (\frac{a^{2}}{3} + \frac{b^{2}}{12})$

RESNICK AND HALLIDAY-RIGID BODY DYNAMICS - I-PROBLEMS

Home
Ask Doubt
Profile