Figure shown two blocks in contact sliding down an inclined surfase of inclination 30∘. The friction coefficient between the block of mass 4.0kg and the incline is μ2=0.30. Find the acceleration of 2.0kg block. (g=10m/s2)
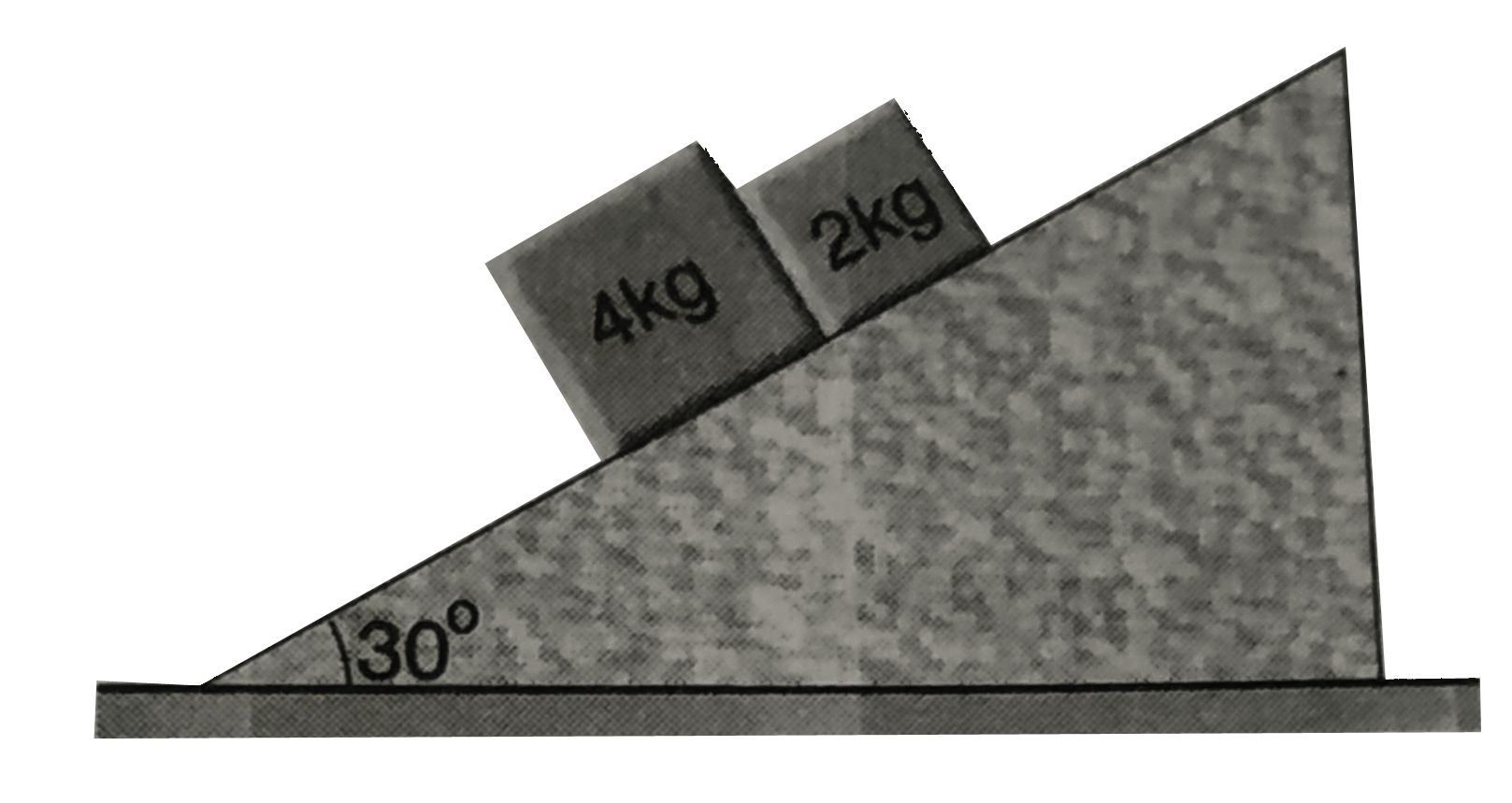
Since μ1<μ2 ,acceleration of 4kg block, if allowed to move seperately. But as the 2.0kg block is behind the 4.0kg block both of them will move with same acceleration say a. Taking both the block as a single system,
Force down the plane on the system =(4+2)gsin30∘
=(6)(10)(12)=30N
Force up the plane on the system
=μ1(2)(g)cos30∘+μ2(4)(g)cos30∘
=(2μ1+4μ2)gcos30∘
=(2×0.2+4×0.3)(10)(0.86)
=13.76N
:. Net force down the plane is F=30−13.76=16.24N
:. Acceleration of both the block down the plane will be a.
a=F4+216.246=2.7m/s2
Topper's Solved these Questions
- BOOK - DC PANDEYCHAPTER - LAWS OF MOTIONEXERCISE - Exercise 8.110videos
LAWS OF MOTION
- BOOK - DC PANDEYCHAPTER - LAWS OF MOTIONEXERCISE - Exercise 8.29videos
LAWS OF MOTION
- BOOK - DC PANDEYCHAPTER - LAWS OF MOTIONEXERCISE - Medical entrances gallery39videos
LAWS OF MOTION
- BOOK - DC PANDEYCHAPTER - KINEMATICS 1EXERCISE - INTEGER_TYPE15videos
KINEMATICS 1
- BOOK - DC PANDEYCHAPTER - LAWS OF THERMODYNAMICSEXERCISE - Level 2 Subjective18videos
LAWS OF THERMODYNAMICS
Similar Questions
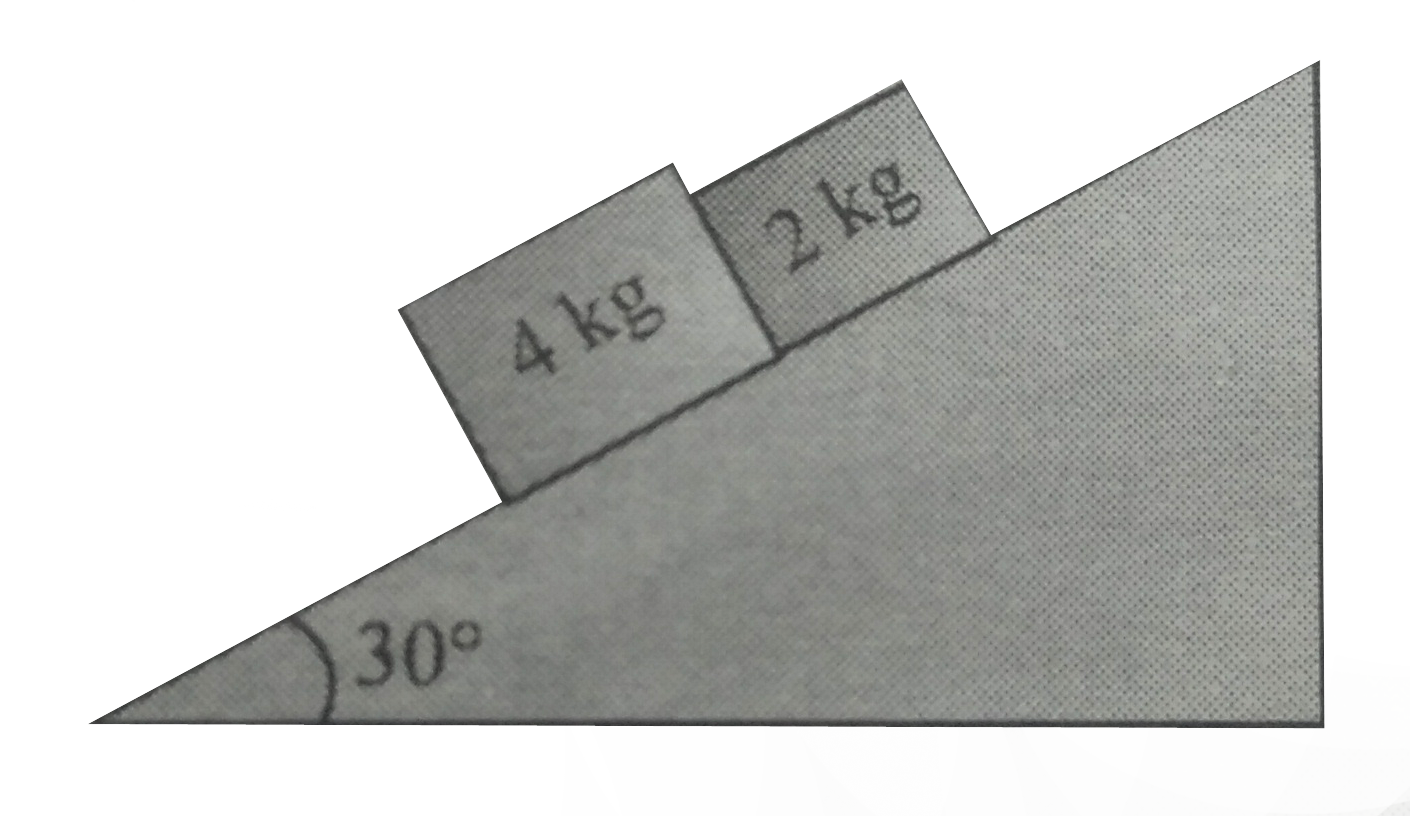
Figure shown two blocks in contact sliding down an inclined surface of inclination 30∘. The block of mass 2kgand the incline is μ1=0.20 and the incline is μ2=0.30 .Find the acceleration of 2.0kg block (in ms−2)g=10ms−2
 View Solution
View SolutionFigure shows tow blocks in contact sliding down an inclined surface of inclination 300. The friction coefficient between the block o fmass 2.0 kg and the incline is μ1 and that between the block of mas 4.0 kg and the incline is μ2. Calculate teh acceleration of the 2.0 kg bloc if a. μ1=0.20andμ2=0.30b.μ1=0.30andμ2=0.20.Take g=10ms2.
 View Solution
View Solution
Knowledge Check
- Question 1 - Select One
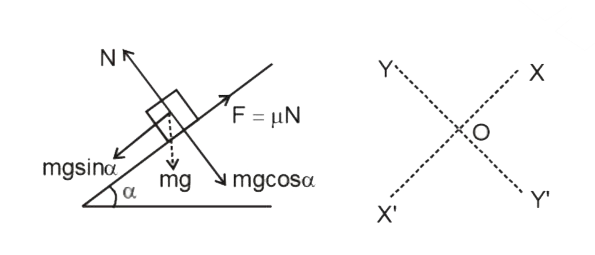
A block of mass m slides down an inclined plane of inclination θ with uniform speed The coefficient of friction between the block and the plane is μ. The contact force between the block and the plane is .
Amgsinθ√1+μ2B√(mgsinθ)2+(μmgcosθ)2CmgsinθDmg - Question 2 - Select One
A block of mass m slides down an inclined plane of inclination θ with uniform speed. The coefficient of friction between the block and the plane is μ. The contact force between the block and the plane is
AmgBmgsinθ√1+μ2CmgsinθD√(mgsinθ)2+(μmgcosθ)2
Figure shows two blocks in contact sliding down an inclined surface of inclination 30º. The friction coefficient between the block of mass 2.0 kg and the incline is μ1, and that between the block of mass 4.0 kg and the incline is μ2. Calculate the acceleration of the 2.0 kg block if μ1 = 0.30 and μ2 = 0.20, Take g = 10 m/s2
 View Solution
View SolutionIn Fig. 6-52, two blocks, in contact, slide down an inclined plane AC of inclination 30∘ . The coefficient of kinetic friction between the 2.0 kg block and the incline is u1=0.20 and that between the 4.0 kg block and the incline is μ2=0.30. Find the magnitude of the acceleration.
 View Solution
View SolutionA 20kg box is gently placed on a rough inclined plane of inclination 30∘ with horizontal. The coefficient of sliding friction between the box and the plane is 0.5.Find the acceleration of the box down the incline.
 View Solution
View SolutionA block of mass 10 kg is sliding on a surface inclined at 30∘ with horizontal . If coefficient of friction between the block and the surface is 0.5 , find acceleration produced in the block . Take g=9.8m/2 .
View SolutionA block of mass 10 kg is kept on a fixed rough (μ=0.8) inclined plane of angle of inclination 30∘. The frictional force acting on the block is
 View Solution
View SolutionA block slides down on inclined plane (angle of inclination 60∘ ) with an accelration g/2 Find the coefficient friction
 View Solution
View Solution

