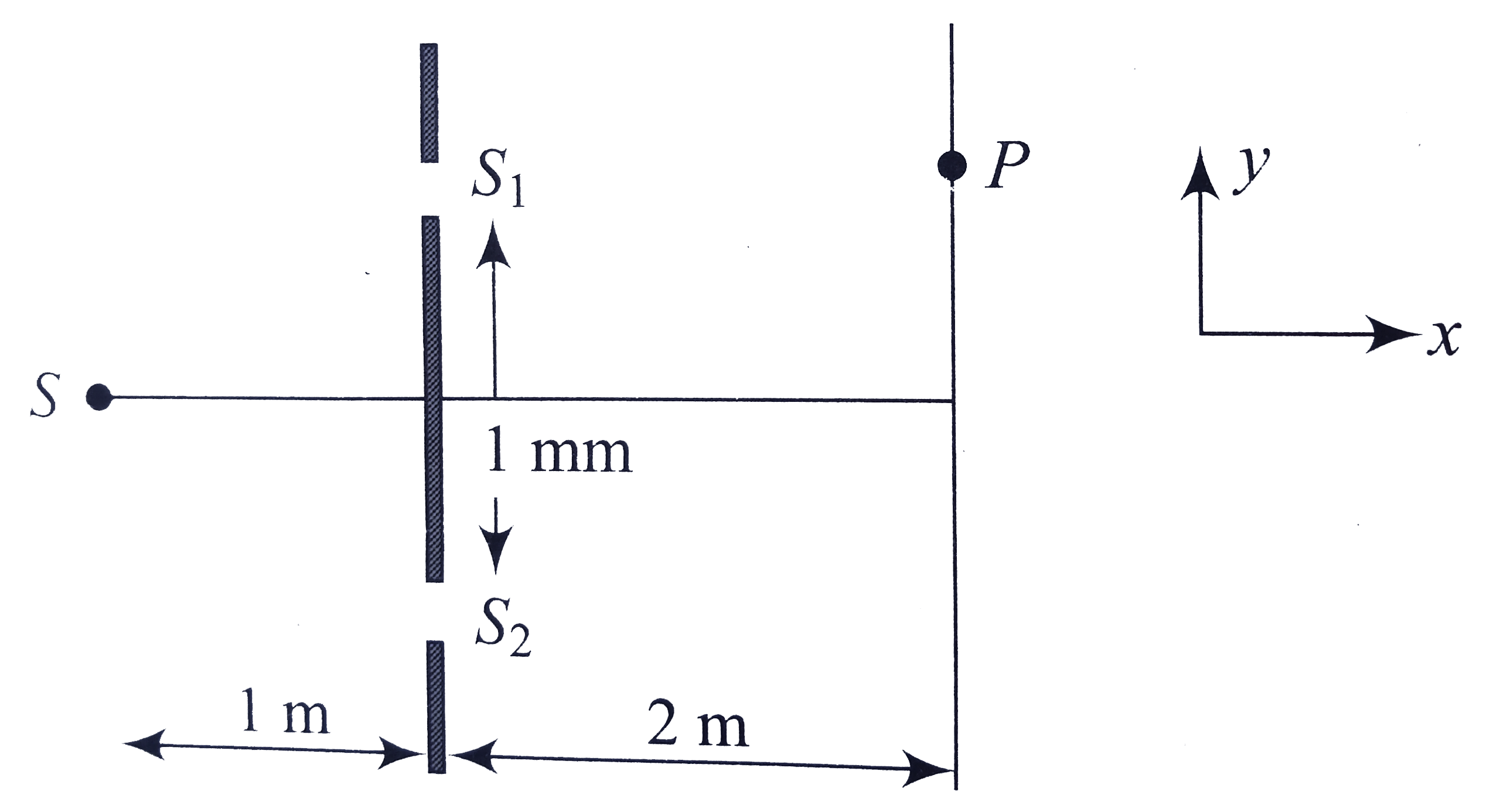
Figure shows a Young's double slit experiment set-up. The source S of wavelength 4000Å oscillates along y-axis according to the equation y−sinπt, where y is in millimeters and t is in seconds. The distance two slits S1andS2 is 0.5 mm.

The position of central maxima as a function of time is 4sinπt
The position of central maxima as a function of time is −4sinπt
The instant at which maximum intensity occurs at P for the first time is 1πsin−1(5980)
The instant at which minimum intensity occurs at P for the first time is 1πsin−1(2780)
The correct Answer is:B, C, D
Topper's Solved these Questions
- WAVE OPTICSBOOK - DC PANDEYCHAPTER - WAVE OPTICSEXERCISE - For JEE Advanced C. Comprehension Type Questions. Passage (I)
2 Videos
- WAVE OPTICSBOOK - DC PANDEYCHAPTER - WAVE OPTICSEXERCISE - For JEE Advanced C. Comprehension Type Questions. Passage (II)
2 Videos
- WAVE OPTICSBOOK - DC PANDEYCHAPTER - WAVE OPTICSEXERCISE - For JEE Advanced Only one option is correct
23 Videos
- SOLVED PAPERS 2018BOOK - DC PANDEYCHAPTER - SOLVED PAPERS 2018EXERCISE - JIPMER
22 Videos
Similar Questions
In a modified YDSE the sources S of wavelength 5000 A oscillates about axis of setup according to the equation y=0.5sin(π6)t where y is in millimeter and t in second. At what time ti will the intensity at P, a point exactly in front of slit S1 be maximum for the first time?
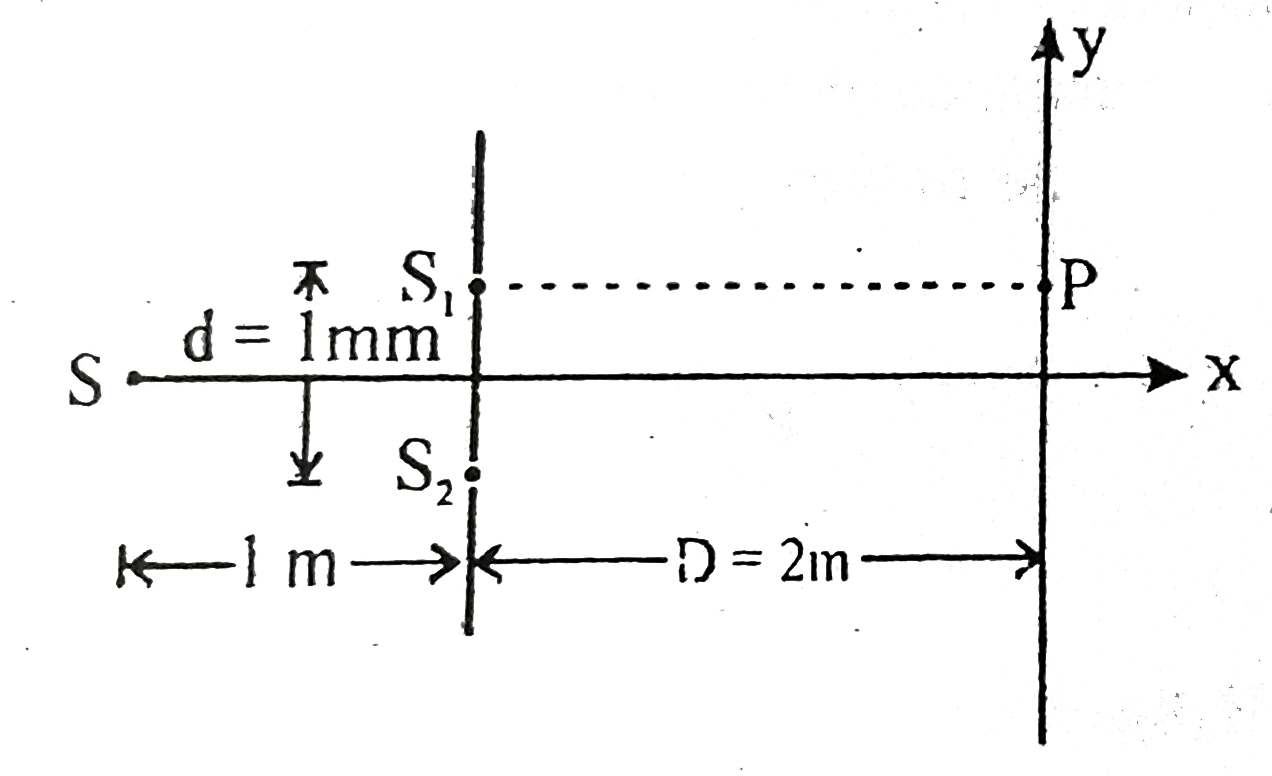
View SolutionIn a Young's double-slit experiment set up, source S of wavelength 6000Å illuminates two slits S1 and S2 which act two coherent sources. The sources S oscillates about its shown position according to the eqation y=1+cosπt, where y is in millimeter and t in second.
At t=0, fringe width is β1, and at t=2 s, width of figure is β2. Then
View Solution
Knowledge Check
In young’s double slit experiment with a source of light of wavelength 6320Å, the first maxima will occur when
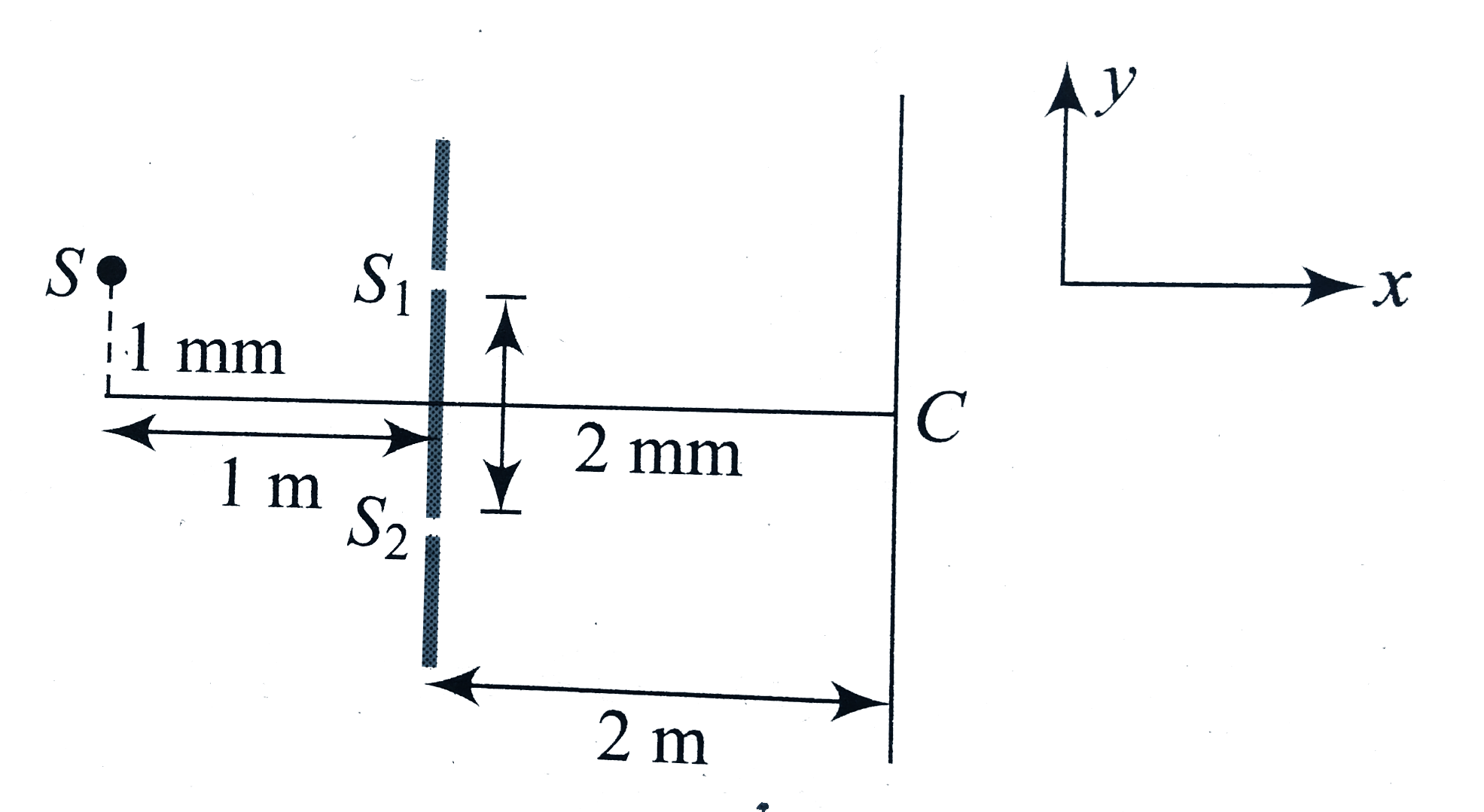
APath difference is 9480ÅBPhase difference is 2π radianCPath difference is6320ÅDPhase difference is π radianIn a Young's double-slit experiment set up, source S of wavelength 6000Å illuminates two slits S1 and S2 which act two coherent sources. The sources S oscillates about its shown position according to the eqation y=1+cosπt, where y is in millimeter and t in second.

At t=0, fringe width is β1, and at t=2 s, width of figure is β2. ThenAβ1>β2Bβ2>β1Cβ1=β2Ddata is insufficientIn a Young's double-slit experiment set up, source S of wavelength 6000Å illuminates two slits S1 and S2 which act two coherent sources. The sources S oscillates about its shown position according to the eqation y=1+cosπt, where y is in millimeter and t in second.
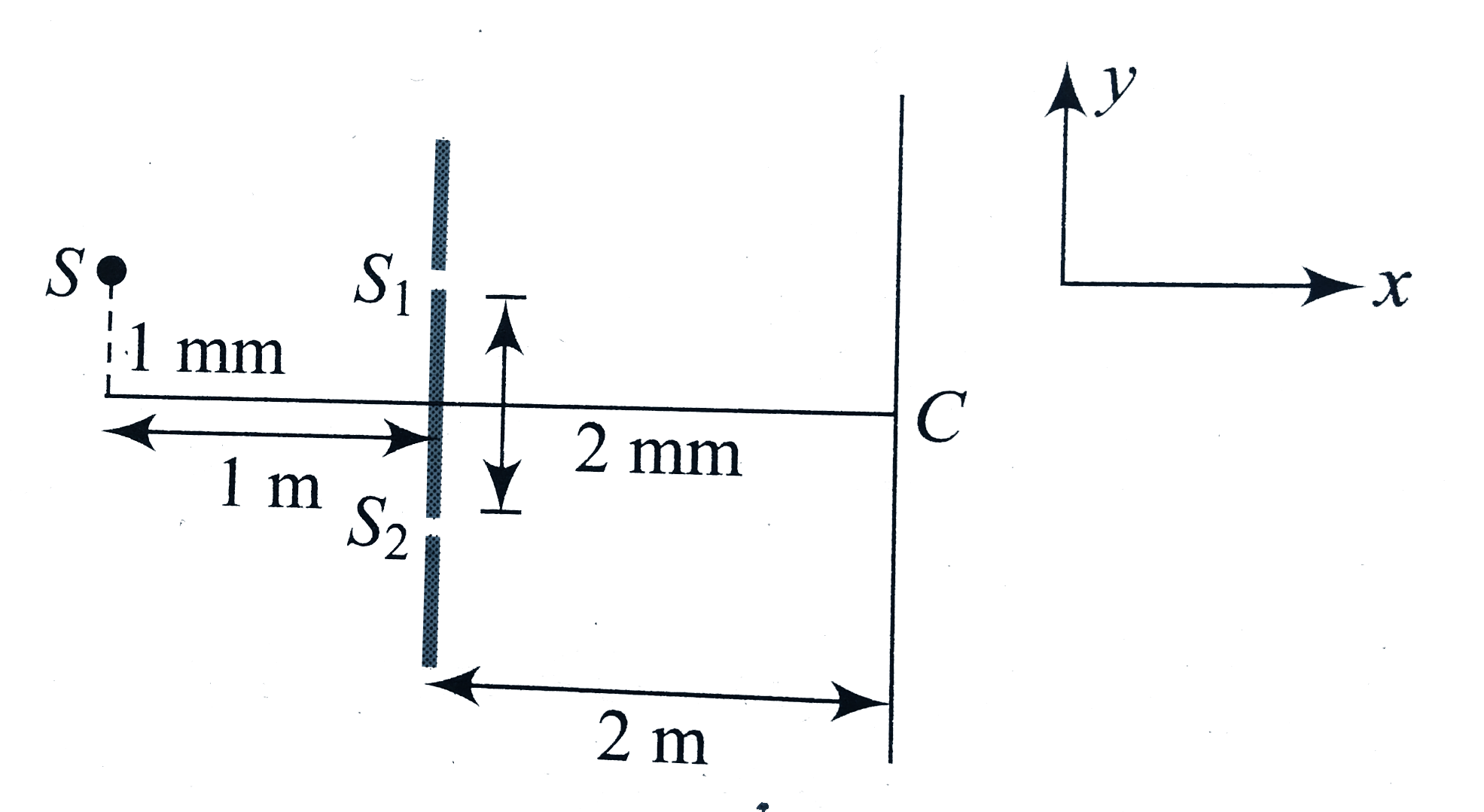
At t=1 s, a slab of thickness 2×10−3mm an refractive index 1.5 is placed just in front of S1. The central maximum is formed atA1 mm above CB1 mm below CC2 mm above CD2 mm below C
In a Young's double-slit experiment set up, source S of wavelength 6000Å illuminates two slits S1 and S2 which act two coherent sources. The sources S oscillates about its shown position according to the eqation y=1+cosπt, where y is in millimeter and t in second.
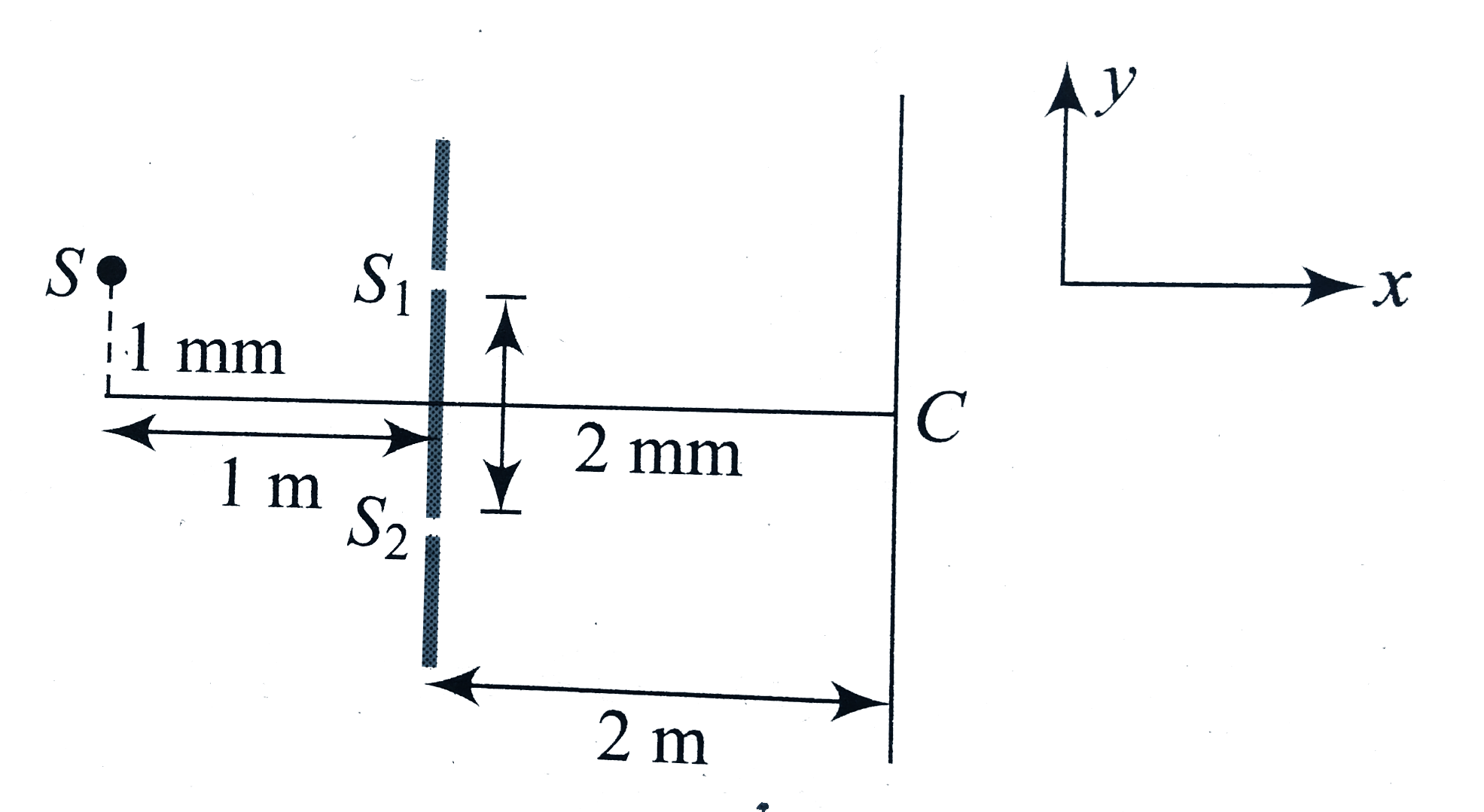
At t=2 s, the position of central maxima is
View SolutionIn young's double-slit experiment set up, sources S of wavelength 50 nm illumiantes two slits S1 and S2 which act as two coherent sources. The sources S oscillates about its own position according to the equation y=0.5sinπt, where y is in nm and t in seconds. The minimum value of time t for which the intensity at point P on the screen exaclty in front of the upper slit becomes minimum is
View SolutionIn a Young's double-slit experiment set up, source S of wavelength 6000Å illuminates two slits S1 and S2 which act two coherent sources. The sources S oscillates about its shown position according to the eqation y=1+cosπt, where y is in millimeter and t in second.

At t=2 s, the position of central maxima is
View SolutionIn young's double-slit experiment set up, sources S of wavelength 50 nm illumiantes two slits S1 and S2 which act as two coherent sources. The sources S oscillates about its own position according to the equation y=0.5sinπt, where y is in nm and t in seconds. The minimum value of time t for which the intensity at point P on the screen exaclty in front of the upper slit becomes minimum is

View SolutionIn Young’s double slit experiment, the distance between the two slits is made half, then the fringe width will become
View Solution
