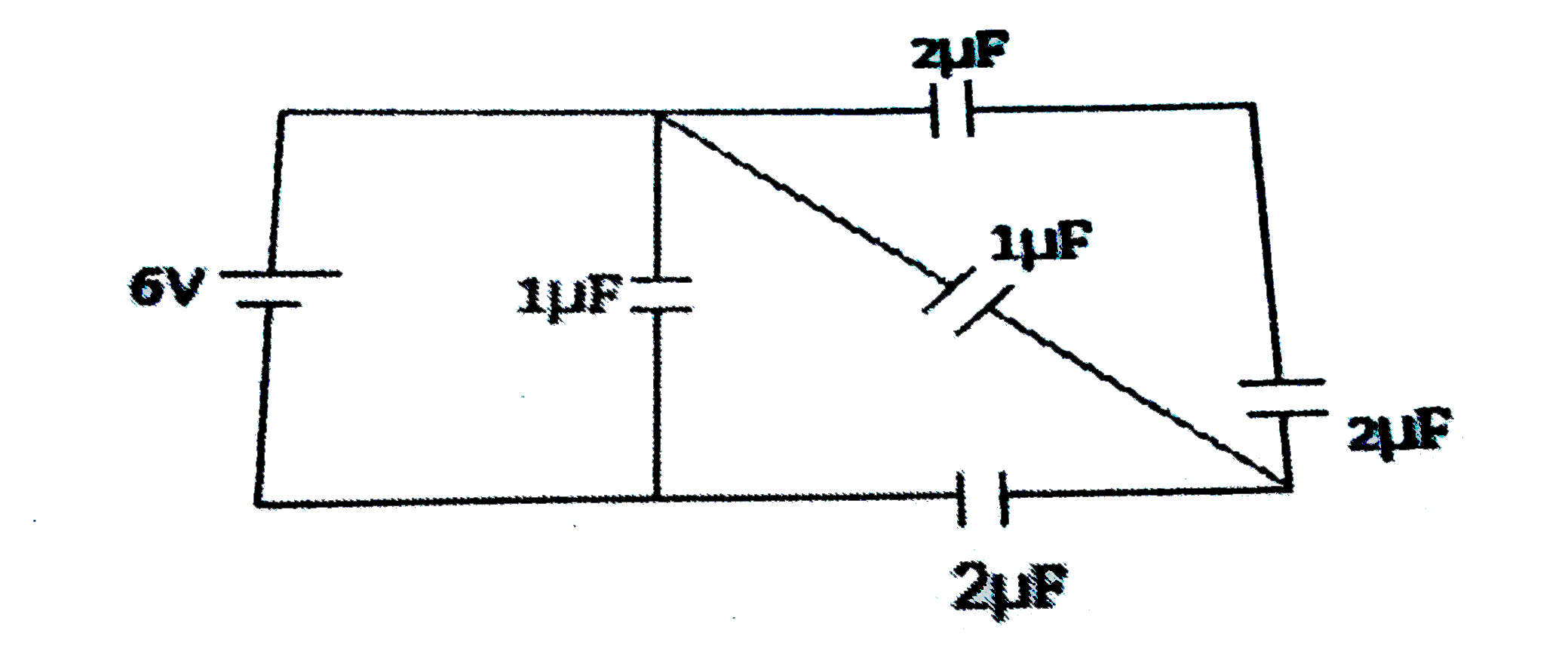
Find the total energy stored in the capacitors in the given network of Fig.

Text Solution

Verified by Experts

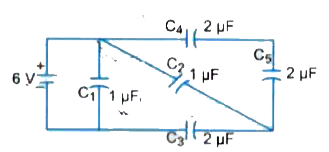
In fig , capacitors $C_{4}$ and $C_{5}$ of 2 $μ F$ each are in series , their equivalent capacitance
$C . = \frac{C_{4} C_{5}}{C_{4} + C_{5}} = \frac{2 \times 2}{2 + 2} = 1 μ F$
Now C. and $C_{2}$ are in parallel , hence their equivalent capacitance
$C . . = C_{2} + C . = 1 + 1 = 2 μ F$
Further C.. and $C_{3}$ are in series , their equivalent capacitance
$C_{0} = \frac{C . . C_{3}}{C . . + C_{3}} = \frac{2 \times 2}{2 + 2} = 1 μ F$
Now C. and $C_{2}$ are in parallel , hence their equivalent capacitance $C . . = C_{2} + C . = 1 + 1 = 2 μ F$
Further C.. and $C_{3}$ are in series , their equivalent capacitance
$C_{0} = \frac{C . . C_{3}}{C . . + C_{3}} = \frac{2 \times 2}{2 + 2} = 1 μ F$
Finally , $C_{0}$ is in parallel with $C_{1}$ , hence net resultant capacitance $C = C_{0} + C_{1} = 1 + 1 = 2 μ F$
$∴$ Total energy stored in the capacitors in the given network
$U = \frac{1}{2} C V^{2} = \frac{1}{2} \times 2 \times 10^{- 6} \times {(6)}^{2} = 3.6 \times 10^{- 5} J$

|

Updated on:21/07/2023

Class 12 PHYSICS ELECTRIC CHARGES AND FIELDS

Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One
Find out total energy stored in the capacitors.
A $500 μ J$
B $300 μ J$
C $600 μ J$
D $1300 μ J$
Question 2 - Select One
Find the total energy stored in capacitors in the network shown in Fig.

A3.6 × 10 − 5 J
B0.6 × 10 − 5 J
C5.6 × 10 − 5 J
Dnone of these

U-LIKE SERIES-ELECTROSTATIC POTENTIAL AND CAPACITANCE -LONG ANSWER QUESTIONS-I

Home
Ask Doubt
Profile