For a prism kept in air, it is found that for an angle of incidence , the angle of refraction A, angle of deviation and anble of emergence e become equal. Then, the refractive index of the prism is
1.73
1.15
1.5
1.33
The correct Answer is:a.
To find the refractive index of the prism given that the angle of incidence (I) is 60 degrees and the angle of refraction (A), angle of deviation (Δ), and angle of emergence (e) are all equal, we can follow these steps:
Step 1: Understand the relationships
We know from the problem that:
- I=60∘
- A=Δ=e
Step 2: Use the prism formula
In a prism, the relationship between the angles can be expressed as:
I+e=A+Δ
Since A=Δ=e, we can substitute e in the equation:
I+e=A+e
This simplifies to:
I=A
Thus, we have:
A=60∘
Step 3: Identify the case of minimum deviation
Since I=e and A=Δ, this indicates that we are at the condition of minimum deviation. In this case, the angle of deviation Δ can be expressed as:
Δmin=I
Thus:
Δmin=60∘
Step 4: Use the formula for refractive index
The refractive index n of a prism can be calculated using the formula:
n=sin(A+Δmin2)sin(A2)
Substituting the known values:
- A=60∘
- Δmin=60∘
We have:
n=sin(60∘+60∘2)sin(60∘2)
This simplifies to:
n=sin(60∘)sin(30∘)
Step 5: Calculate the sine values
Using known values:
- sin(60∘)=√32
- sin(30∘)=12
Substituting these values into the equation:
n=√3212=√3
Step 6: Final answer
Thus, the refractive index of the prism is:
n=√3≈1.732
We know from the problem that:
- I=60∘
- A=Δ=e
In a prism, the relationship between the angles can be expressed as:
I+e=A+Δ
Since A=Δ=e, we can substitute e in the equation:
I+e=A+e
This simplifies to:
I=A
Thus, we have:
A=60∘
Since I=e and A=Δ, this indicates that we are at the condition of minimum deviation. In this case, the angle of deviation Δ can be expressed as:
Δmin=I
Thus:
Δmin=60∘
The refractive index n of a prism can be calculated using the formula:
n=sin(A+Δmin2)sin(A2)
Substituting the known values:
- A=60∘
- Δmin=60∘
n=sin(60∘+60∘2)sin(60∘2)
This simplifies to:
n=sin(60∘)sin(30∘)
Using known values:
- sin(60∘)=√32
- sin(30∘)=12
n=√3212=√3
Thus, the refractive index of the prism is:
n=√3≈1.732
Topper's Solved these Questions
- GEOMETRICAL OPTICSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - GEOMETRICAL OPTICSEXERCISE - Assertion-Reasoninig
2 Videos
- GEOMETRICAL OPTICSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - GEOMETRICAL OPTICSEXERCISE - Linked Comprehension
51 Videos
- GEOMETRICAL OPTICSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - GEOMETRICAL OPTICSEXERCISE - Subjective
26 Videos
- ELECTRON,PHONTS,PHOTOELECTRIC EFFECT & X-RAYSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - ELECTRON,PHONTS,PHOTOELECTRIC EFFECT & X-RAYSEXERCISE - dpp 3.3
15 Videos
- HEATING EFFECT OF CURRENTBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - HEATING EFFECT OF CURRENTEXERCISE - Thermal Power in Resistance Connected in Circuit
27 Videos
Similar Questions
For a prism kept in air, it is found that for an angle of incidence 60∘ , the angle of refraction 'A', angle of deviation δ, and angle of emergence 'e' become equal. The minimum angle of incidence of a ray that will be transmitted through the prism is
View SolutionFor a ray of light refracted through a prism of angle 60∘, the angle of incidence is equal to the angle of emergence, each equal to 45∘. Find the refractive index of the material of the prism.
View Solution
Knowledge Check
A ray of light is incident at 60∘ on one face of a prism of angle 30∘ and the emergent ray makes 30∘ with the incident ray. The refractive index of the prism is
A1.732B1.414C1.5D1.33
If the angle of minimum deviation is of 60∘ for an equilateral prism , then the refractive index of the material of the prism is
View SolutionFor a prism of refractive index 1.732, the angle of minimum deviation is equal to the angle of the prism. The angle of the prism is
View SolutionIf the angle of prism is 60∘ and the angle of minimum deviation is 40∘ , the angle of refraction will be
View SolutionA ray of light is incident at 60∘ on one face of a prism of angle 30∘ and the emergent ray makes 30∘ with the incident ray. The refractive index of the prism is
View SolutionIn an isosceles prism of angle 45∘, it is found that when the angle of incidence is same as the prism angle, the emergen ray grazes the emergent surface.
Find the refractive index of the material of the prism. For what angle of incidenc, the angle of deviation will be minimum?
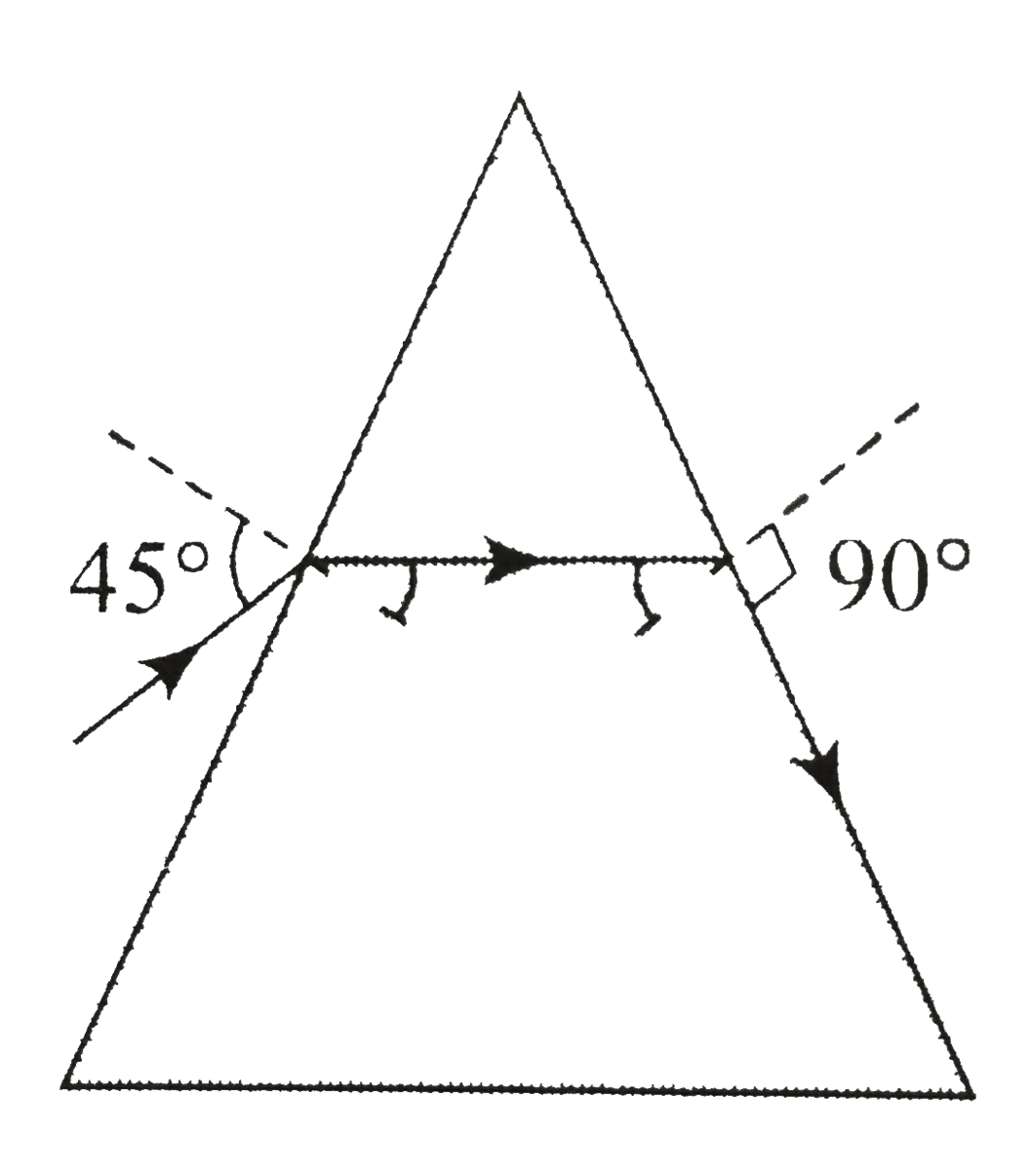
View SolutionThe angle of minimum deviation measured with a prism is 30∘ and the angle of prism is 60∘. The refractive index of prism material is
View SolutionFor a small angled prism, angle of minimum deviation (δ) varies with the refractive index of the prism as shown in the graph
View Solution
