For an LCR circuit driven at frequency ω, the di equation reads Ldidt+Ri+qC=Vi=Vmsinωt
(a) Multiply the equation by i and simplify where possible.
(b) Interpret each term physically.
(c) Cast the equation in the form of a conservation of energy statement.
(d) Integrate the equation over one cycle to find that the phase difference between v and i must be acute.Solution in Gujrati
Let circuit L-C-R as shown in figure.

From Kirchhoff.s law of closed circuit,
VL+VC+VR=Vmsinωt
Ldidt+qC+iR=Vmsinωt…(1)
Multiplying I on both side,
Lididt+qCi+i2R=VmIsinωt...(2)
where Lididt=ddt(12Li2)
indicates the rate of change of energy stored in an inductor.
i2R=P Joule heat loss
qCi=ddt(q22C) indicates the rate of change of energy stored in the capacitor and Vi = rate at which driving force pours in energy.
Hence, equation (2) is in the form of conservation of energy statement.
Integrating both sides of equation (2) with respect to time over one full cycle (0 - T) we may write
∫T0ddt(12Li2+q22C)dt+∫T0Ri2dt=12∫T0V
where, Vmsinωt=V
∴[0+12i2Rt]T0=12∫T0Vidt
∴[0+12i2RT]=12∫T0Vidt
∴ 0+(Positive)=∫T0Vidt[∵i2RT>0]
∴∫T0Vidt>0 if phase difference between V and i is a constant and acute angle.
Topper's Solved these Questions
- ALTERNATING CURRENTS BOOK - KUMAR PRAKASHANCHAPTER - ALTERNATING CURRENTS EXERCISE - SECTION-D MCQs (DARPAN BASED ON TEXTBOOK)
188 Videos
- ALTERNATING CURRENTS BOOK - KUMAR PRAKASHANCHAPTER - ALTERNATING CURRENTS EXERCISE - SECTION-D MCQs (COMPETITIVE EXAMS)
64 Videos
- ALTERNATING CURRENTS BOOK - KUMAR PRAKASHANCHAPTER - ALTERNATING CURRENTS EXERCISE - SECTION-C NCERT EXEMPLAR ( Short Answer)
6 Videos
- ATOMSBOOK - KUMAR PRAKASHANCHAPTER - ATOMSEXERCISE - Section-D -MCQs asked in GUJCET / Board Exam
34 Videos
Similar Questions
In an A.C. circuit the inductive reactance is defined as ......
View SolutionPower factor of AC series circuit having resistance R and inductor L having angular frequency ω is ……..
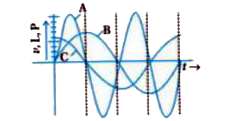
View SolutionA device 'X' is connected to an ac source. The variation of voltage, current and power in one complete cycle is shown in figure.
(a) Which curve shows power consumption over a full cycle ?
(b) What is the average power consumption over a cycle ?
(c) Identify the device 'X'.
View SolutionThe ratio of longest wavelength and the shortest wavelength observed in the 5 spectral series of emission spectrum of hydrogen is .......
View SolutionThe equation of electromotive forces for an electric circuit containing resistance and self inductance is E=R i+Ldidt, where E is the electromotive force given to the circuit, R, the resistance and L, the coefficient of induction. Find the current i at time t when (i) E=0 (ii) E=a ,non-zero constant.
View SolutionFor an LCR circuit driven at frequency ω, the equation reads Ldidt+Ri+qC=υi=υmsinωt
(i) Multiply the equation by I and simplify where possible. (ii) Interpret each term physically.
(iii) Cast the equation in the form of a conservation of energy statement.
(iv) Intergrate the equation over one cycle to find that the phase difference between υ and i must be acute.
View Solutionएक प्रत्यावर्ती धारा - स्त्रोत की वोल्टेज समीकरण V=110sin(ωt−π/6) वोल्ट तथा परिपथ में धारा की समीकरण i=5sin(ωt−π/6) एम्पियर है । V व i बीच कलान्तर तथा परिपथ की प्रतिबाधा ज्ञात कीजिए । परिपथ में औसत शक्ति - क्षय क्या होगा ?
View Solutionएक प्रत्यावर्ती धारा - परिपथ में वोल्टेज समीकरण V=110sinωt वोल्ट तथा परिपथ में धारा समीकरण i=5sin(ωt−π/6) एम्पियर है । परिपथ की प्रतिबाधा तथा शक्ति - गुणाक की गणना कीजिए ।
View Solutionनिम्नलिखित के विमीय सूत्र निकालें।
(a) आवेश Q (b) विद्युत विभव V (c) धारिता C तथा (d) प्रतिरोध R
इनसे सम्बंधित कुछ समीकरण इस प्रकार हैं
Q=It,U=Vit,Q=CV,V=RI,
जहाँ I विद्युत -धारा, t समय तथा U ऊर्जा हैं।
View SolutionThe equation of electromotive force for an electric circuit containing resistance and self inductance is E=Ri+Ldidt, where E is the electromotive force is given to the circuit, R the resistance and L, the coefficient of induction. Find the current i at time t when E = 0.
View SolutionThe equation of an alternating voltage is E=220sin(ωt+π/6) and the equation of the current in the circuit is I=10sin(ωt−π/6). Then the impedance of the circuit is
View SolutionIn a series LCR circuit, an alternating emf (V) and current (I) are given by the equation V=V0sinωt,I=I0sin(ωt+π3) The average power dissipated in the circuit over a cycle of AC is
View Solutionf आवृति के AC परिपथ में किसी यंत्र के सिरों के मध्य का विभान्तर V तथा धारा i को दर्शाया गया है V=5cosωt वोल्ट तथा i=2 sin ωt ऐम्पियर (जहाँ (ω=2πf) तो यंत्र का अपव्यय होता है
View SolutionIn a series LCR circuit , and alternating emf (v) and current (i) are given by the equation v=v0sinωt,i=i0sin(ωt+π3). The average power dissipated in the circuit over a cycle of AC is
View SolutionFor an LCR circuit driven at frequency omega , the equation reads L (di)/(dt) + Ri + (q)/(C ) = upsilon_(i) = upsilon_(m) sin omega t (i) Multiply the equation by I and simplify where possible. (ii) Interpret each term physically. (iii) Cast the equation in the form of a conservation of energy statement. (iv) Intergrate the equation over one cycle to find that the phase difference between upsilon and i must be acute.
View Solution
