Frequency of oscillation of a body is 5 Hz when a force F1 is applied and 12 Hz when another force F2 is applied. If both forces F1 and F2 are applied together, then frequency of oscillation of the body will be
13 Hz
169 Hz
62 Hz
52 Hz
The correct Answer is:C
To solve the problem, we need to find the frequency of oscillation when both forces F1 and F2 are applied together. The frequencies given for the individual forces are 5 Hz and 12 Hz, respectively.
1. Understanding the relationship between frequency and force constant:
The frequency of oscillation f of a mass m attached to a spring (or any oscillatory system) is given by the formula:
f=12π√km
where k is the spring constant (or force constant).
2. Setting up equations for the given frequencies:
For the first force F1 (frequency = 5 Hz):
5=12π√k1m⟹k1=(5⋅2π)2m=100π2m
For the second force F2 (frequency = 12 Hz):
12=12π√k2m⟹k2=(12⋅2π)2m=576π2m
3. Finding the combined spring constant:
When both forces are applied together, the effective spring constant k is the sum of the individual spring constants:
k=k1+k2=100π2m+576π2m=676π2m
4. Calculating the new frequency:
Now, we can find the new frequency f when both forces are applied:
f=12π√km=12π√676π2mm=12π√676π2=12π⋅26π=262=13 Hz
Final Answer:
The frequency of oscillation of the body when both forces F1 and F2 are applied together is 13 Hz.
---
The frequency of oscillation f of a mass m attached to a spring (or any oscillatory system) is given by the formula:
f=12π√km
where k is the spring constant (or force constant).
For the first force F1 (frequency = 5 Hz):
5=12π√k1m⟹k1=(5⋅2π)2m=100π2m
12=12π√k2m⟹k2=(12⋅2π)2m=576π2m
When both forces are applied together, the effective spring constant k is the sum of the individual spring constants:
k=k1+k2=100π2m+576π2m=676π2m
Now, we can find the new frequency f when both forces are applied:
f=12π√km=12π√676π2mm=12π√676π2=12π⋅26π=262=13 Hz
The frequency of oscillation of the body when both forces F1 and F2 are applied together is 13 Hz.
Topper's Solved these Questions
Similar Questions
Knowledge Check
When a constant force is applied to a body, it moves with unifrom
AaccelerationBvelocityCspeedDmomentumWhen a constant force is applied to a body, it moves with unifrom
AspeedBvelocityCaccelerationDmomentumA force F1 of 500 N is required to push a car of mass 1000 kg slowly at constant speed on a leveled road. If a force F2 of 1000N is applied, the acceleration of the car will be :
AzeroB1.5ms−2C1ms−2D0.5ms−2
What force F must be applied so that m1 and m2 are at rest on m3 in
View SolutionWhen a force is applied to a body, state its effect.
View SolutionA force F is applied horizontally on mass m1 as shown in figure. Find the contact force between m1 and m2
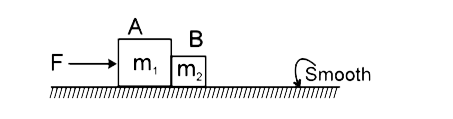
View SolutionA force produces an acceleration of 5 m/s2 in a body and same force an acceleration of 15m/s2 in another body. The acceleration produced by the same force when applied to the combination of two bodies will be -
View SolutionA wire has an elongation of 0.04m when a force F is applied on it . Now its length & diameter are double and same force F is applied on it. Find new elongation in the wire
View Solution
