From a rigid support, a rope of mass M is hung vertically. To the free end of this rope, a wooden block of mass m is attached. At the lower end of rope, a transverse pulse of wavelength λ is produced. What will be wavelength of pulse when it reaches the top of the rope ?
To solve the problem, we need to determine the wavelength of a transverse pulse as it travels up a vertically hung rope with a mass attached to its free end. We will follow these steps:
Step 1: Understand the system
- We have a rope of mass M hanging vertically from a rigid support.
- A wooden block of mass m is attached to the free end of the rope.
- A transverse pulse is produced at the lower end of the rope with an initial wavelength λ.
Step 2: Determine the tension in the rope
- At the lower end of the rope, the tension T1 is equal to the weight of the block, which is T1=mg.
- As we move up the rope, the tension increases due to the weight of the rope itself. The tension at the top of the rope, T2, can be expressed as:
T2=(m+M)g
where M is the mass of the rope and m is the mass of the block.
Step 3: Calculate the speed of the wave
- The speed of a wave on a rope is given by the formula:
v=√Tμ
where T is the tension in the rope and μ is the mass per unit length of the rope.
- For the lower end of the rope, using T1:
v1=√T1μ=√mgμ
Step 4: Relate speed to wavelength
- The wavelength λ is related to the speed v and frequency f of the wave:
λ=vf
- Hence, we can express the wavelength at the lower end as:
λ=1μ√mgμ
Step 5: Calculate the new wavelength at the top
- At the top of the rope, the tension is T2:
v2=√T2μ=√(m+M)gμ
- The new wavelength λ′ at the top can be expressed as:
λ′=v2f
- Since the frequency remains constant, we can relate the two wavelengths:
λ′λ=v2v1
Step 6: Substitute and simplify
- Substituting the expressions for v1 and v2:
λ′λ=√(m+M)gμ√mgμ
- Simplifying gives:
λ′λ=√m+Mm
- Rearranging gives us the final expression for the new wavelength:
λ′=λ√m+Mm
Final Answer
The wavelength of the pulse when it reaches the top of the rope is:
λ′=λ√m+Mm
- We have a rope of mass M hanging vertically from a rigid support.
- A wooden block of mass m is attached to the free end of the rope.
- A transverse pulse is produced at the lower end of the rope with an initial wavelength λ.
- At the lower end of the rope, the tension T1 is equal to the weight of the block, which is T1=mg.
- As we move up the rope, the tension increases due to the weight of the rope itself. The tension at the top of the rope, T2, can be expressed as:
T2=(m+M)g
where M is the mass of the rope and m is the mass of the block.
- The speed of a wave on a rope is given by the formula:
v=√Tμ
where T is the tension in the rope and μ is the mass per unit length of the rope.
- For the lower end of the rope, using T1:
v1=√T1μ=√mgμ
- The wavelength λ is related to the speed v and frequency f of the wave:
λ=vf
- Hence, we can express the wavelength at the lower end as:
λ=1μ√mgμ
- At the top of the rope, the tension is T2:
v2=√T2μ=√(m+M)gμ
- The new wavelength λ′ at the top can be expressed as:
λ′=v2f
- Since the frequency remains constant, we can relate the two wavelengths:
λ′λ=v2v1
- Substituting the expressions for v1 and v2:
λ′λ=√(m+M)gμ√mgμ
- Simplifying gives:
λ′λ=√m+Mm
- Rearranging gives us the final expression for the new wavelength:
λ′=λ√m+Mm
The wavelength of the pulse when it reaches the top of the rope is:
λ′=λ√m+Mm
Topper's Solved these Questions
- BOOK - MODERN PUBLICATIONCHAPTER - WAVESEXERCISE - REVISION EXERCISES107videos
WAVES
- BOOK - MODERN PUBLICATIONCHAPTER - WAVESEXERCISE - MULTIPLE CHOICE QUESTIONS88videos
WAVES
- BOOK - MODERN PUBLICATIONCHAPTER - WAVESEXERCISE - NCERT EXEMPLAR PROBLEMS31videos
WAVES
- BOOK - MODERN PUBLICATIONCHAPTER - UNITS AND MEASUREMENTEXERCISE - CHAPTER PRACTICE TEST15videos
UNITS AND MEASUREMENT
- BOOK - MODERN PUBLICATIONCHAPTER - WORK, ENERGY AND POWER EXERCISE - Chapter Practice Test16videos
WORK, ENERGY AND POWER
Similar Questions
A uniform rope of elngth l and mass m hangs vertically from a rigid support. A block of mass m is attached to the free end of the rope. A transverse pulse of wavelength λ is produced at the lower end of the rope. The wavelength of the pulse, when it reaches the top of the rope. is aλ. Find a2
 View Solution
View SolutionA uniform rope of length 12m and mass 6kg hangs vertically from a rigid support . A block of mass 2kg is attached to the free end of the rope . A transverse pulse of wavelengths 0.06m is produced at the lower end of the rope . What is the wavelength of the pulse when it reaches the top of the rope?
View Solution
Knowledge Check
- Question 1 - Select One
A uniform rope of legnth L and mass m1 hangs vertically from a rigid support. A block of mass m2 is attached to the free end of the rope. A transverse pulse of wavelength λ1 is produced at the lower end of the rope. The wavelength of the pulse when it reaches the top of the rope is λ2. The ratio λ2λ1 is
A√m1+m2m1B√m1m2C√m1+m2m2D√m2m1 - Question 2 - Select One
A uniform rope of legnth L and mass m1 hangs vertically from a rigid support. A block of mass m2 is attached to the free end of the rope. A transverse pulse of wavelength λ1 is produced at the lower end of the rope. The wavelength of the pulse when it reaches the top of the rope is λ2. The ratio λ2λ1 is
A√m1m2B√m1+m2m2C√m2m1D√m1+m2m1 - Question 3 - Select One
A uniform rope of length L and mass m1 hangs vertically from a rigid support. A block of mass m2 is attached to the free end of the rope. A transverse pulse of wavelength λ1 is produced at the lower end of the rope. The wavelength of the pulse when it reaches the top of the rope is λ2. The ratio λ2λ1 is
A√m1m2B√m1+m2m2C√m2m1D√m1+m2m1
A uniform rope of length 12 mm and mass 6 kg hangs vertically from a rigid support. A block of mass 2 kg is attached to the free end of the rope. A transverse pulse of wavelength 0.06 m is produced at the lower end of the rope. What is the wavelength of the pulse when it reaches the top of the rope?
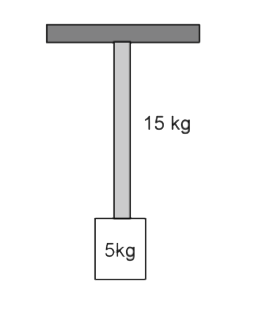
View SolutionA uniform rope of length 10 m and mass 15 kg hangs vertically from a rigid support. A block of mass 5 kg is attached to the free end of the rope. A transverse pulse of wavelength 0.08 m is produced at the lower end of the 3 rope. The wavelength of the pulse when it reaches the top of the rope will be-
 View Solution
View SolutionA uniform rope of length 20m and mass 8kg hangs vertically froma rigid support. A block of mass 2kg is attached to the free end of the rope. A transverse pulse of wavelength 0.06m is produced at the lower end of the rope. What is the wavelength of the pulse when it reaches the top os the rope?
View SolutionA uniform rope of length 10 cm and mass 4 kg hangs vertically from a rigid support. A block of mass 2 kg is attached to the free end of the rope. A transverse pulse of wavelength 0.06 m is produced at the lower end of the rope, what is the wavelength of the pulse when it reaches the top of the rope?
View SolutionA uniform rope of length 12m and mass 6kg hangs vertically from a rigid support. A block of mass 2kg is attached to the free end of the rope. A transverse pulse of wavelength 0.06m is produced at the lower end of the rope. What is the wavelength of the pulse whwn it reaches the top of the rope?
View Solution

