(i) The equation of wave in a string fixed at both end is y=2sinπtcosπx. Find the phase difference between oscillations of two points located at x=0.4m and x=0.6m.
(ii) A string having length L is under tension with both the ends free to move. Standing wave is set in the string and the shape of the string at time t=0 is as shown in the figure. Both ends are at extreme. The string is back in the same shape after regular intervals of time equal to T and the maximum displacement of the free ends at any instant is A. Write the equation of the standing wave.

The correct Answer is:(i) π
(ii) y=AcosπxLcos2πTt
Step by step video, text & image solution for (i) The equation of wave in a string fixed at both end is y = 2 sin pi t cos pi x. Find the phase difference between oscillations of two points located at x = 0.4 m and x = 0.6 m. (ii) A string having length L is under tension with both the ends free to move. Standing wave is set in the string and the shape of the string at time t = 0 is as shown in the figure. Both ends are at extreme. The string is back in the same shape after regular intervals of time equal to T and the maximum displacement of the free ends at any instant is A. Write the equation of the standing wave. by Physics experts to help you in doubts & scoring excellent marks in Class 11 exams.
Updated on:21/07/2023Topper's Solved these Questions
Similar Questions
A long taut string is plucked at its centre. The pulse travelling on it can be described as y(x,t)=e−(x+2t)2+e−(x−2t)2. Draw the shape of the string at time t=0, a short time after t=0 and a long time after t=0.
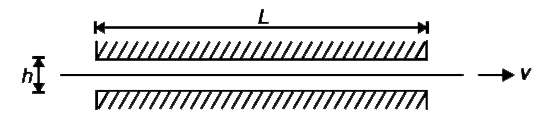
View SolutionDuring a painting process, a thin, flat tape of width b (dimension perpendicular to the plane of the figure) is pulled through a paint filled channel of length L. The density and viscosity of the paint liquid is ρ and η respectively. The tape is pulled at a constant speed v and width of the channel is h. Find the minimum force needed to pull the tape.
View Solution(i) The cause of increases in kinetic energy when a man starts running without his feet slipping on ground is asked to two students. Their answers are– Harshit: Cause of increase in kinetic energy is work done by friction force. Without friction the man cannot run. Akanksha: Cause of increase in kinetic energy is work done by internal (muscle) forces of the body. Who is right? (ii) An inextensible rope is hanging from a tree. A monkey, having mass m, climbs to a height h grabbing the rope tightly. The monkey starts from rest and ends up hanging motionlessly on the rope at height h. (a) How much work is done by gravity on the monkey? (b) How much work is done by the rope on the monkey? (c) Using work – energy theorem, explain the increase in mechanical energy of the monkey.
View SolutionThe equation of a standing wave, produced on a string fixed at both ends, is y=(0.4cm)sin[(0.314cm−1)x]cos[(600πs−1)t] What could be the smallest length of the string ?
View SolutionStanding Waves In A Strings Fixed At Both Ends
View SolutionA string of mass 'm' and length l, fixed at both ends is vibrating in its fundamental mode. The maximum amplitude is 'a' and the tension in the string is 'T'. If the energy of vibrations of the string is π2a2TηL. Find η
View SolutionThe wave-function for a certain standing wave on a string fixed at both ends is y(x,t)=0.5sin(0.025πx)cos500t where x and y are in centimeters and t is seconds. The shortest possible length of the string is :
View SolutionA string of mass 'm' and length l, fixed at both ends is vibrating in its fundamental mode. The maximum amplitude is 'a' and the tension in the string is 'T'. If the energy of vibrations of the string is π2a2TηL. Find η
View Solutionदोनों किनारों पर बँधी एक डोरी पर अप्रगामी तरंग स्थित है । इसका समीकरण है ,
y=(0.4cm)sin[(π10cm−1)x]cos[(600πs−1)t],
इस डोरी की न्यूनतम संभव लंबाई कितनी है ?
View SolutionThe displacement of a string fixed at both the ends is given by y= 0.8 sin ((pi x)/(3)) cos (60 pi t) where y and x in metres and t is in second. What is the wavelength and frequency of the two interfering waves ?
View SolutionStanding Wave Vibrations of string fixed at both ends
View SolutionEquation of standing waves in a string under tension T=10N is given by Y=2sin(10 pi x)sin(100 pi t) cm where x is in m and t is in second). Linear mass density of string is
View SolutionEquation of standing waves in a string under tension T=10N is given by , Y=2sin(10 pi x)sin(100 pi t)cm x is in m and t is in second).Linear mass density of string is
View SolutionA string of length l along x-axis is fixed at both ends and is vibrating in second harmonic. If at t=0 , y=2.5mm for incident wave, the equation of standing wave is ( T is tension and mu is linear density)
View SolutionThe equation of a standing wave, produced on a string fixed at both ends, is y=(0.4cm)sin[(0.314cm−1)x]cos[(600πs−1)t] What could be the smallest length of the string ?
View Solution
