If a planet of given density were made larger, its force of attraction for an object on its surface would increase because of the greater distance from the object to the centre of the planet. Which effect predominates?
To solve the question, we need to analyze the effects of increasing the size of a planet with a given density on the gravitational force experienced by an object on its surface. We will consider the gravitational force formula and how mass and distance from the center of the planet play a role.
1. Understanding Gravitational Force:
The gravitational force F between two masses is given by Newton's law of gravitation:
F=G⋅m1⋅m2r2
where G is the gravitational constant, m1 is the mass of the planet, m2 is the mass of the object, and r is the distance from the center of the planet to the object.
2. Calculating Mass of the Planet:
The mass m1 of the planet can be expressed in terms of its volume and density:
m1=Volume×Density
For a spherical planet, the volume V is given by:
V=43πR3
where R is the radius of the planet. Therefore, the mass of the planet becomes:
m1=43πR3⋅ρ
where ρ is the density of the planet.
3. Substituting Mass in Gravitational Force Equation:
Substituting the expression for mass into the gravitational force equation gives:
F=G⋅(43πR3⋅ρ)⋅m2R2
Simplifying this, we get:
F=43πGρm2R
4. Analyzing the Effect of Increasing Radius:
From the equation F=43πGρm2R, we see that the gravitational force F is directly proportional to the radius R of the planet. Therefore, if the radius of the planet increases while keeping the density constant, the gravitational force F will increase.
5. Conclusion:
Although increasing the radius increases the distance from the center of the planet, the increase in mass (due to volume increase) has a more significant effect on the gravitational force. Thus, the effect of increasing the radius (and hence the mass) predominates over the effect of increasing distance.
Final Answer:
The effect of increasing the radius of the planet predominates, leading to an increase in the gravitational force experienced by an object on the surface of the planet.
---
The gravitational force F between two masses is given by Newton's law of gravitation:
F=G⋅m1⋅m2r2
where G is the gravitational constant, m1 is the mass of the planet, m2 is the mass of the object, and r is the distance from the center of the planet to the object.
The mass m1 of the planet can be expressed in terms of its volume and density:
m1=Volume×Density
For a spherical planet, the volume V is given by:
V=43πR3
where R is the radius of the planet. Therefore, the mass of the planet becomes:
m1=43πR3⋅ρ
where ρ is the density of the planet.
Substituting the expression for mass into the gravitational force equation gives:
F=G⋅(43πR3⋅ρ)⋅m2R2
Simplifying this, we get:
F=43πGρm2R
From the equation F=43πGρm2R, we see that the gravitational force F is directly proportional to the radius R of the planet. Therefore, if the radius of the planet increases while keeping the density constant, the gravitational force F will increase.
Although increasing the radius increases the distance from the center of the planet, the increase in mass (due to volume increase) has a more significant effect on the gravitational force. Thus, the effect of increasing the radius (and hence the mass) predominates over the effect of increasing distance.
The effect of increasing the radius of the planet predominates, leading to an increase in the gravitational force experienced by an object on the surface of the planet.
Topper's Solved these Questions
- GRAVITATIONBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - GRAVITATIONEXERCISE - Exercise 6.3
16 Videos
- GRAVITATIONBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - GRAVITATIONEXERCISE - Exercise 6.4
16 Videos
- GRAVITATIONBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - GRAVITATIONEXERCISE - Exercise 6.1
13 Videos
- FLUID MECHANICSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - FLUID MECHANICSEXERCISE - INTEGER_TYPE
1 Videos
- KINEMATICS-1BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - KINEMATICS-1EXERCISE - Integer
9 Videos
Similar Questions
If the angular velocity of a planet about its own axis is halved, the distance of geostationary satellite of this planet from the centre of the centre of the planet will become
View SolutionIf a force is applied to an object, away from its centre of mass, it tends to rotate the object.
View SolutionStatement I: In free space a uniform spherical planet of mass M has a smooth narrow tunnel along its diameter. This planet and another superdense small particle of mass M start approaching towards each other from rest under action of their gravitational forces. When the particle passes through the centre of the planet, sum of kinetic energies of both the bodies is maximum.
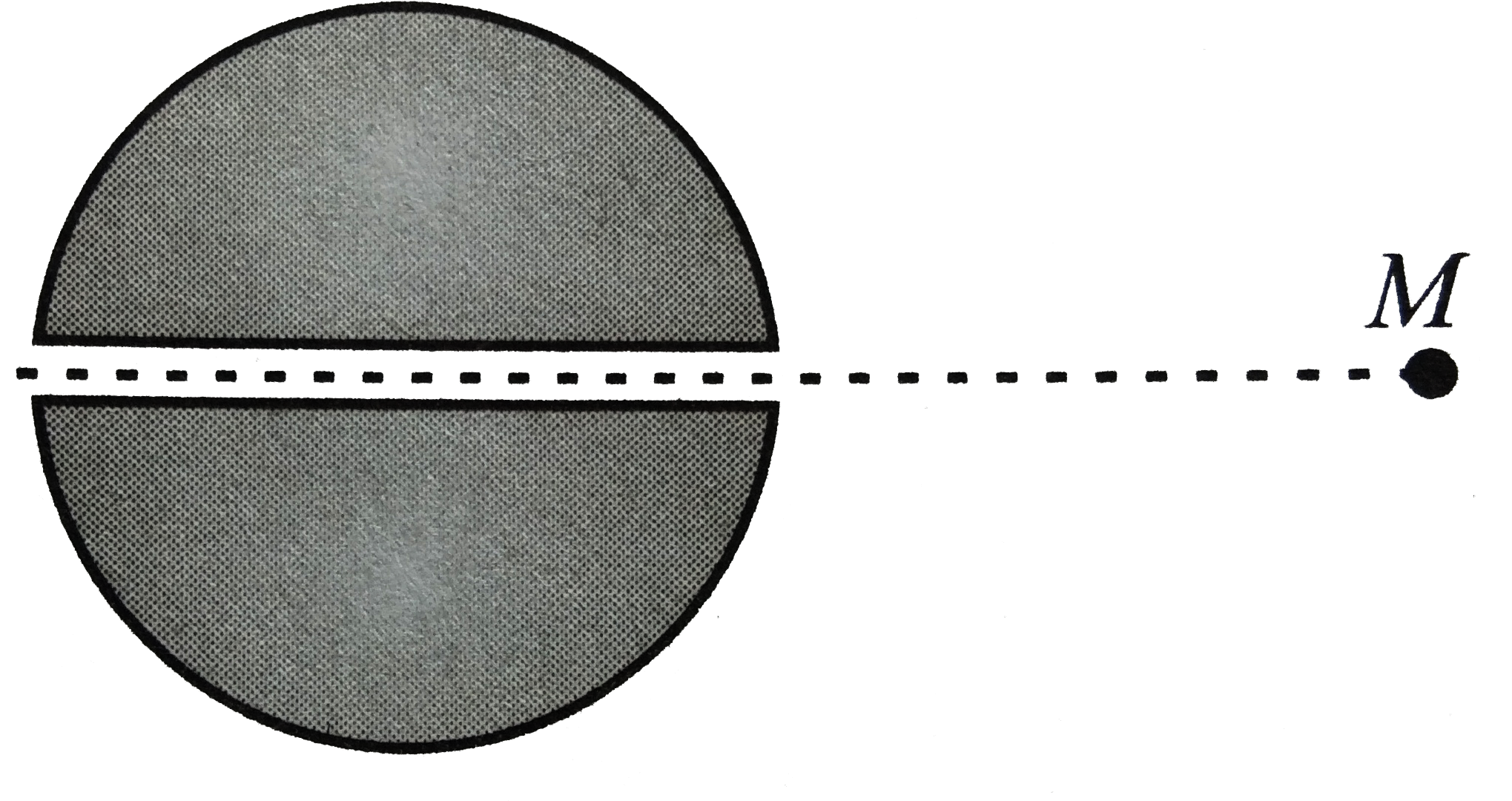
Statement II: When the resultant of all forces acting on a particle or a particle like object (initially at rest) is constant in direction, the kinetic energy of the particle keeps on increasing.
View Solution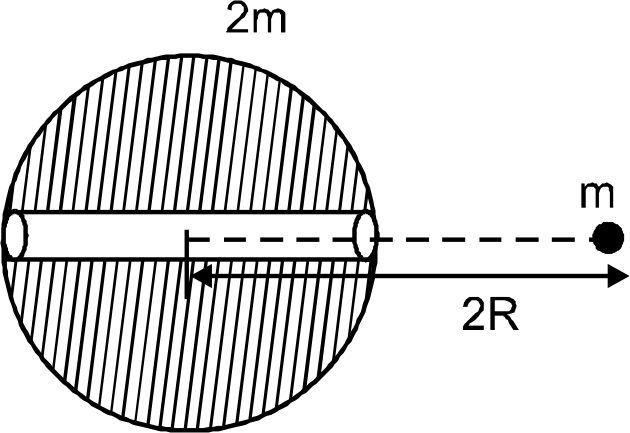
A solid spherical planet of mass 2m and radius 'R' has a very small tunnel along its diameter. A small cosmic particle of mass m is at a distance 2R from the centre of the planet as shown. Both are initially at rest, and due to gravitational attraction, both start moving toward each other. After some time, the cosmic particle passes through the centre of the planet. (Assume the planet and the cosmic particle are isolated from other planets)
View SolutionTwo object are initially at rest on a frictionless surface. Object 1 has a greater mass than object 2. The same constant force start to act on each object. The force is removed from each object after it accelerates over a distance d. after the force is removed from both objects, which statement is correct (p:momentum, K: kinetic energy)?
View SolutionAn object of mass m is raised from the surface of the earth to a height equal to the radius of the earth, that is, taken from a distance R to 2R from the centre of the earth. What is the gain in its potential energy ?
View SolutionA table with smooth horiztonal surface is truning at an angular speed ω abouyt its axis. A groove is made on the surface along a radius and a particle is gently placed inside the groove at a distance a from the centre. Find the speed of the speed of the particle as its distance from teh centre becomes L.
View SolutionA table with smooth horizontal surface is turning at an angular speed ω about its axis. A groove is made on the surface along a radius and a particle is gently placed inside the groove at a distance a from the centre. Find the speed of the speed of the particle as its distance from teh centre becomes L.
View SolutionA table with smooth horizontal surface is turning at an angular speed ω about its axis. A groove is made on the surface along a radius and a particle is gently placed inside the groove at a distance a from the centre. Find the speed of the speed of the particle as its distance from the centre becomes L.
View Solutionin which fo the following cases the centre of mass of a rod is certainly not at its centre?
(A) The density continuously increases from left ot right
(B) The density continuously decreases from left to right
(C) The density decreases from left to right up to the centre and then increases
(D) The density increases from left to right up to the centre and then decreases
Select correct alternative.
View Solution
