In a chain of identical atoms the vibration frequency ω depends on wave number kas ω=ωmaxsin(ka/2), where ωmax is the maximum vibration frequency ω, a is the distance between neighbouring atoms. Making use of this dispersion relation, find the dependence of the number of longitudinal vibrations per unit. frequency interval on ω i.e., dN/ω, if the length of the chain is l. Having obtained dN/ω, find the total number N of possible longitudinal vibrations of the chain.
If the chain has N atoms, we can assume atom number 0 and N+1 held ficed. Then the displacement of the nth atom has the form
un=A(sinmπL,na)sinωt
Here k=mπL. Allowed frequencies then have the form
ω=ωmaxsinka2
In our from only +vek values are allowed.
The number of models in a wave number range dk is
dN=Ldkπ=LπdKdω
But dω=a2ωmaxcoska2dk
Hence dωqadk=a2√ω2max−ω2
So dN=2Lπadω√ω2max−ω2
(b) The total number modes is
N=∫ωmax02Lπadω√ω2_(max)−ω2=2Lπa.π2=La
i.e., the number of atoms in the chain.
Topper's Solved these Questions
- ATOMIC AND NUCLEAR PHYSICSBOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - ATOMIC AND NUCLEAR PHYSICSEXERCISE - Radioactivity
35 Videos
- ATOMIC AND NUCLEAR PHYSICSBOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - ATOMIC AND NUCLEAR PHYSICSEXERCISE - Nuclear Reaction
42 Videos
- ATOMIC AND NUCLEAR PHYSICSBOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - ATOMIC AND NUCLEAR PHYSICSEXERCISE - Properties Of Atom
69 Videos
- DIRECT CURRENTBOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - DIRECT CURRENTEXERCISE - All Questions
15 Videos
Similar Questions
There is a square membrane of area S. Find the number of natural vibrations perpendicular ot its plane in the frequency interval from ωtoω+dω if the propogation velocity of vibrations is equal to v.
View SolutionFind the number of natural transverse vibration of a string length l in the frequency interval from ω to ω+dω if the propagation velocity of vibrations is equal to v. All vibrations are supposed to occur in one plane.
View Solution
Knowledge Check
Which one of the following is the correct relation between frequency/ and angular frequency ω?
Af=πωBω=2πfCf=2ω/πDf=2πωThe graph between wave number (→v) and angular frequency (ω) is
A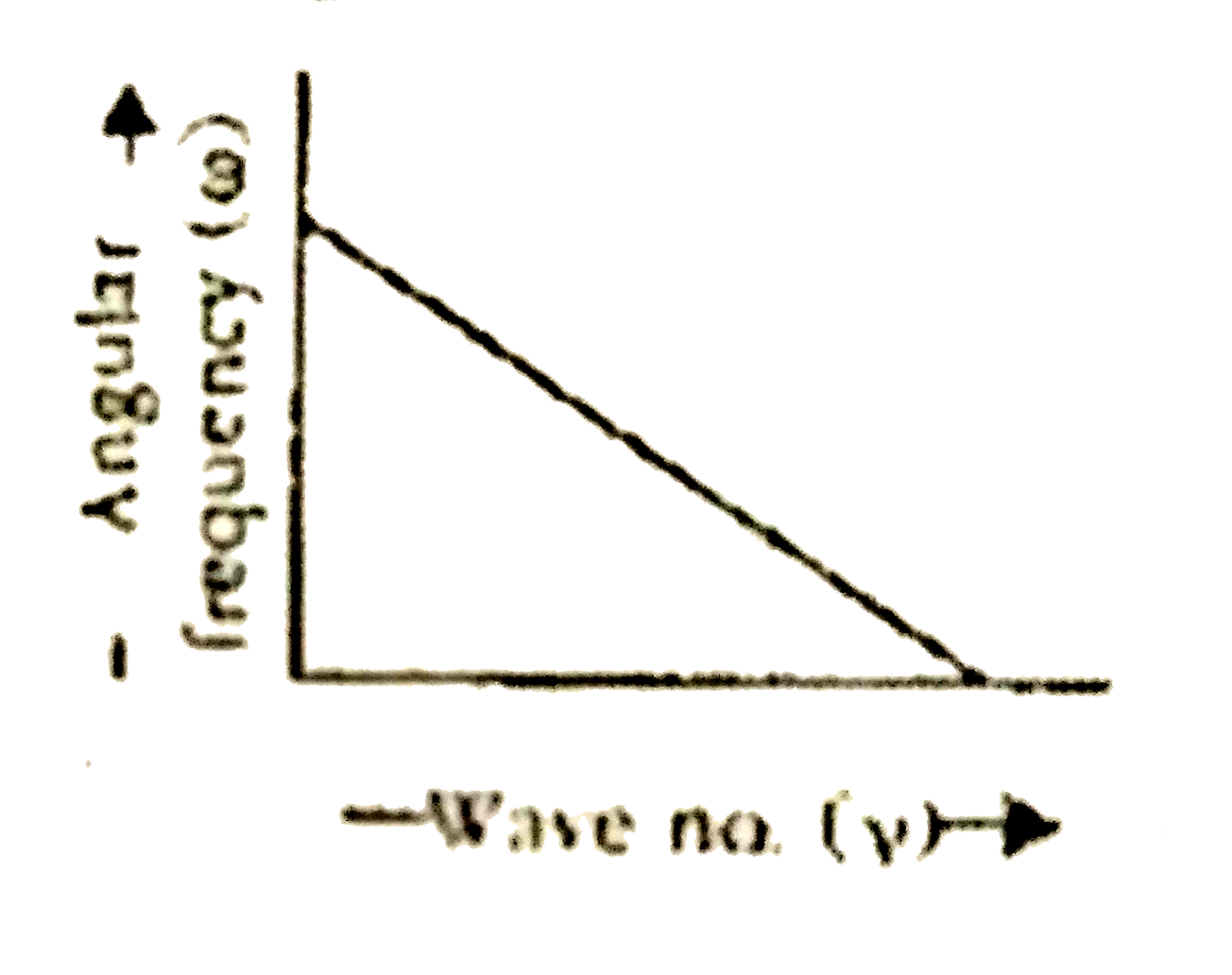 B
B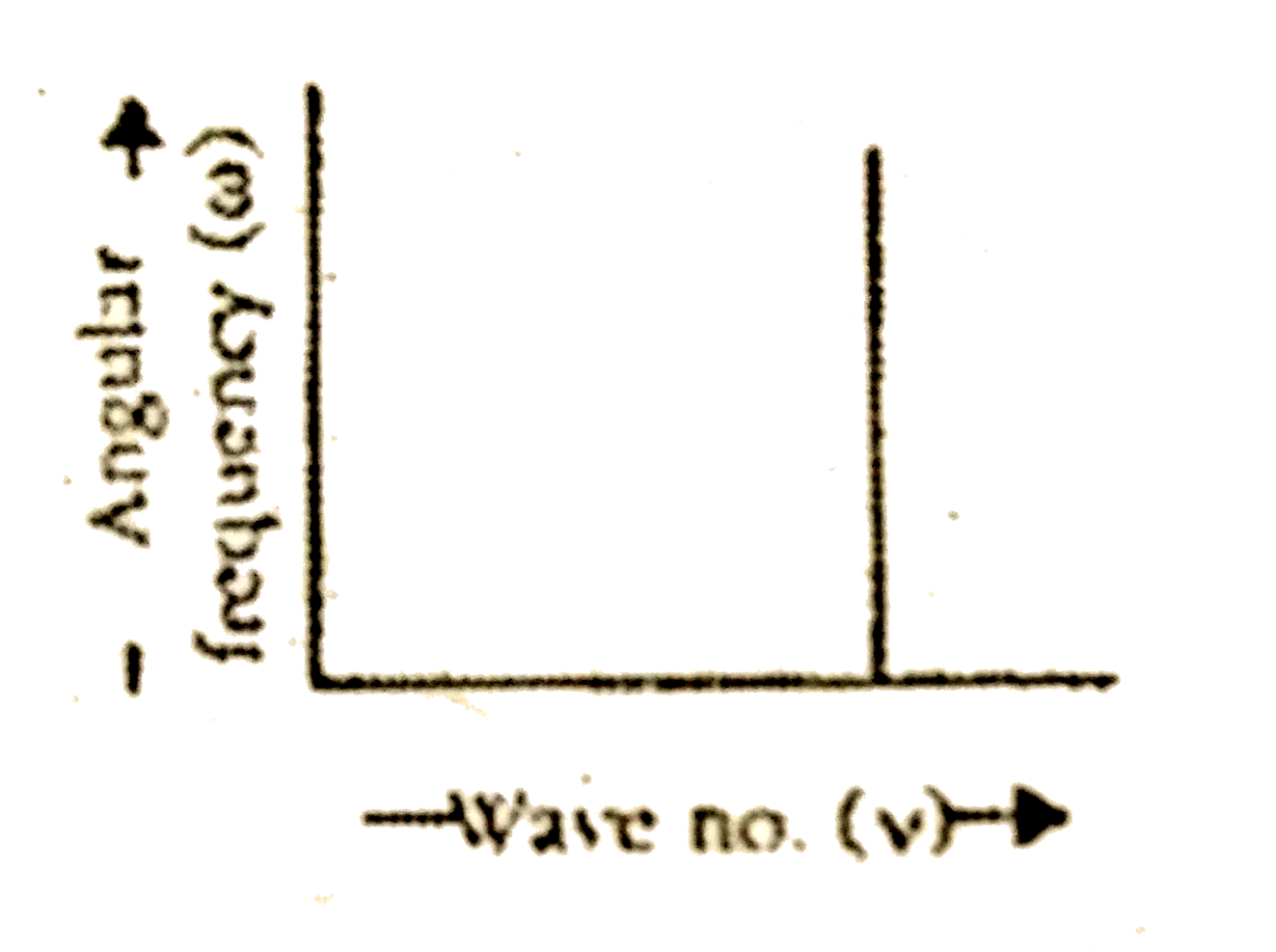 C
C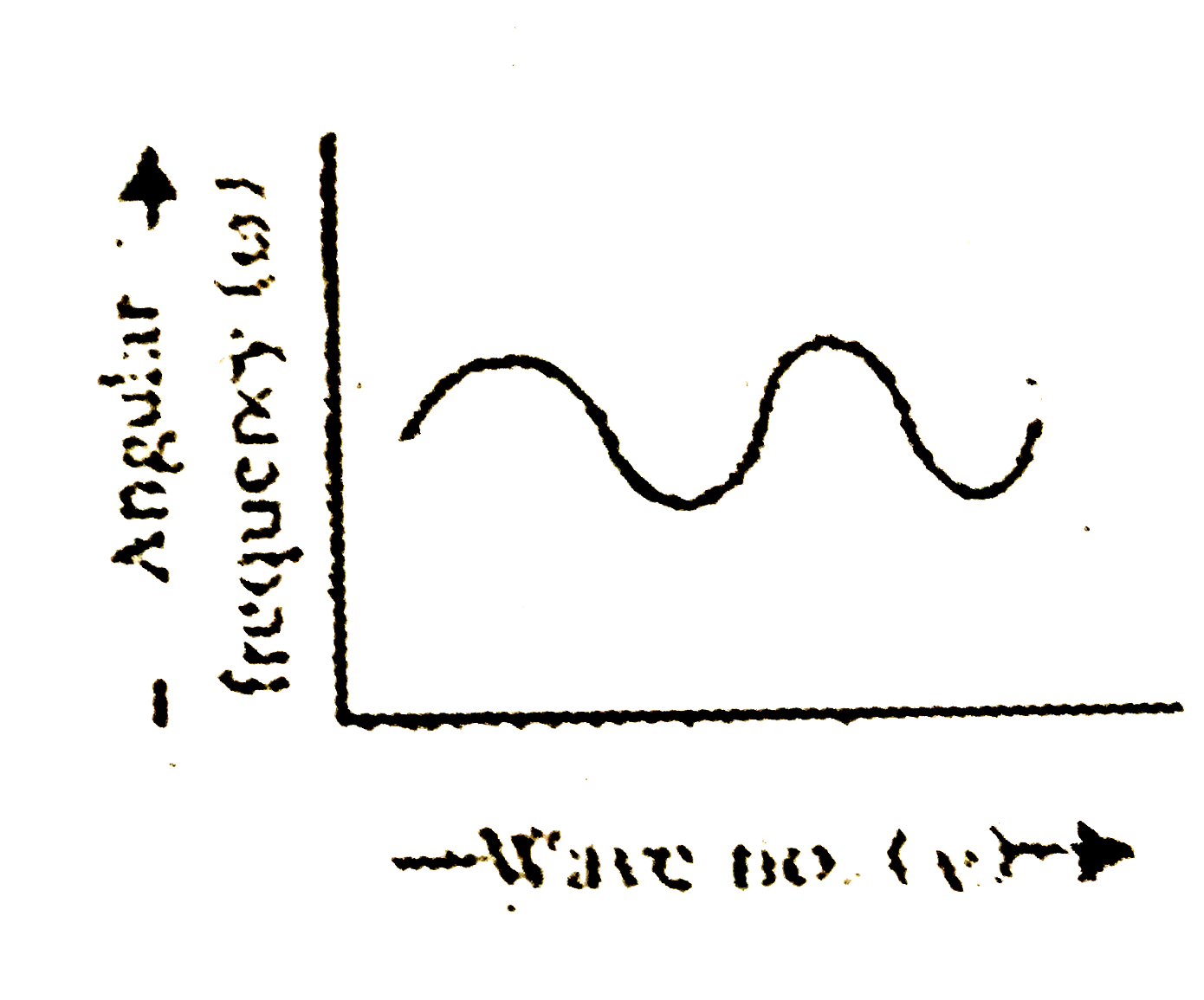 D
D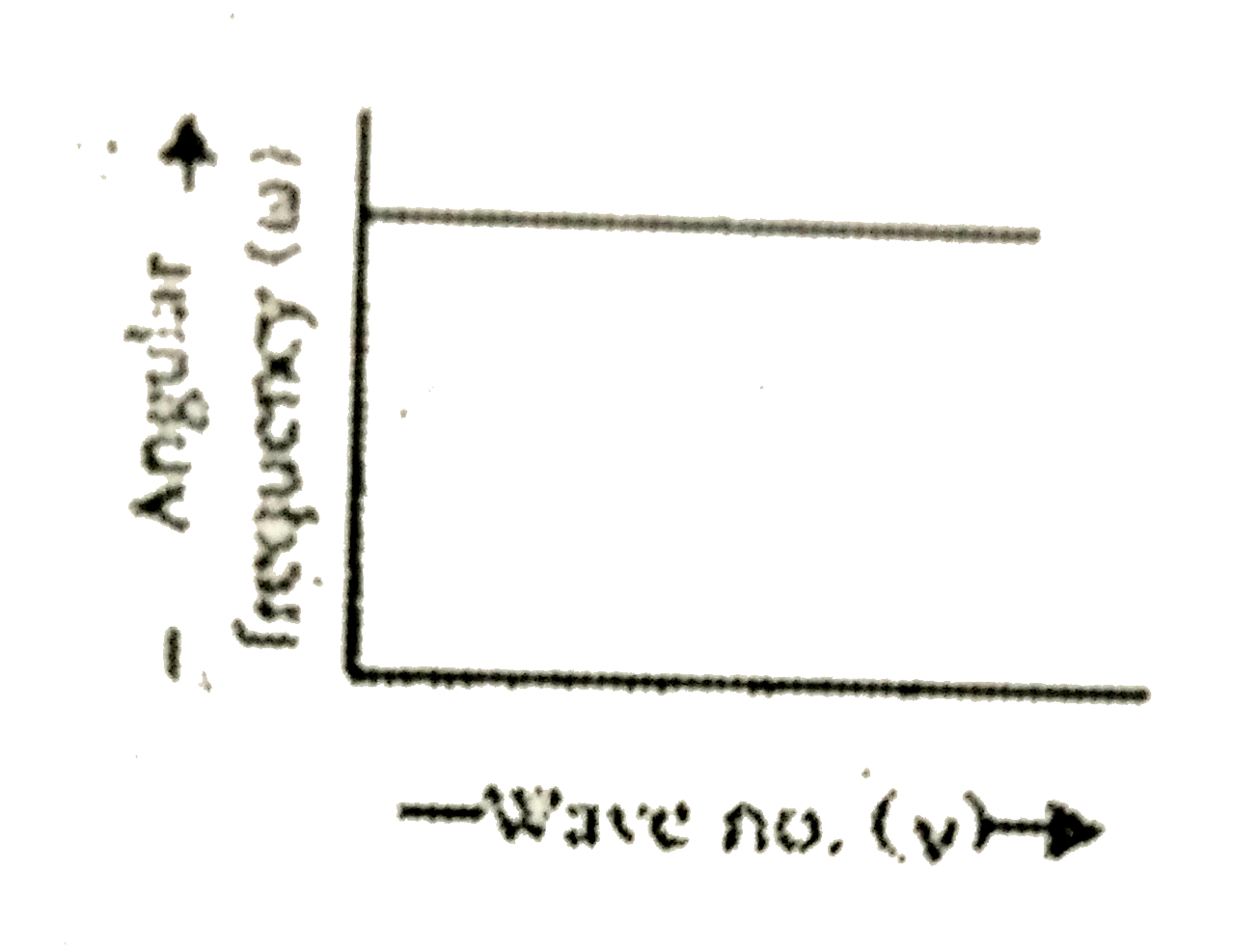
Find the number of natural transverse vibration of a rigtht-angled parallelipoped of volume V in the frquency interval from ω→ω+dω if the propogation velocity of vibrations is equal to v
View SolutionThe ratio of ω/k for a travelling wave (where ω is the angular frequency and k is the angular wave number) is .........
View SolutionIn forcd oscillation of a particle, the amplitude is maximum for a frequency ω1 of the force, while the energy is maximum for a frequency ω2 of the force. What is the relation between ω1 and ω2 ?
View SolutionMaking use of Planck's formula, derive the expressions determining the number of photons per 1cm3 of a cavity at a temperature T in the spectral intervals (ω,ω+dω) and (λ,λ+dλ).
View SolutionThe graph between wave number (→v) and angular frequency (ω) is
View SolutionIn forced oscillation of a particle the amplitude is maximum for a frequency ω1 of the force while the energy is maximum for a frequency ω2 of the force, then .
View Solution
