In the arrangement shown in figure, the string has a mass of 4.5 g. How much time will it take for a transverse disturbance produced at the floor to reach the pulley ? Take g=10ms−2
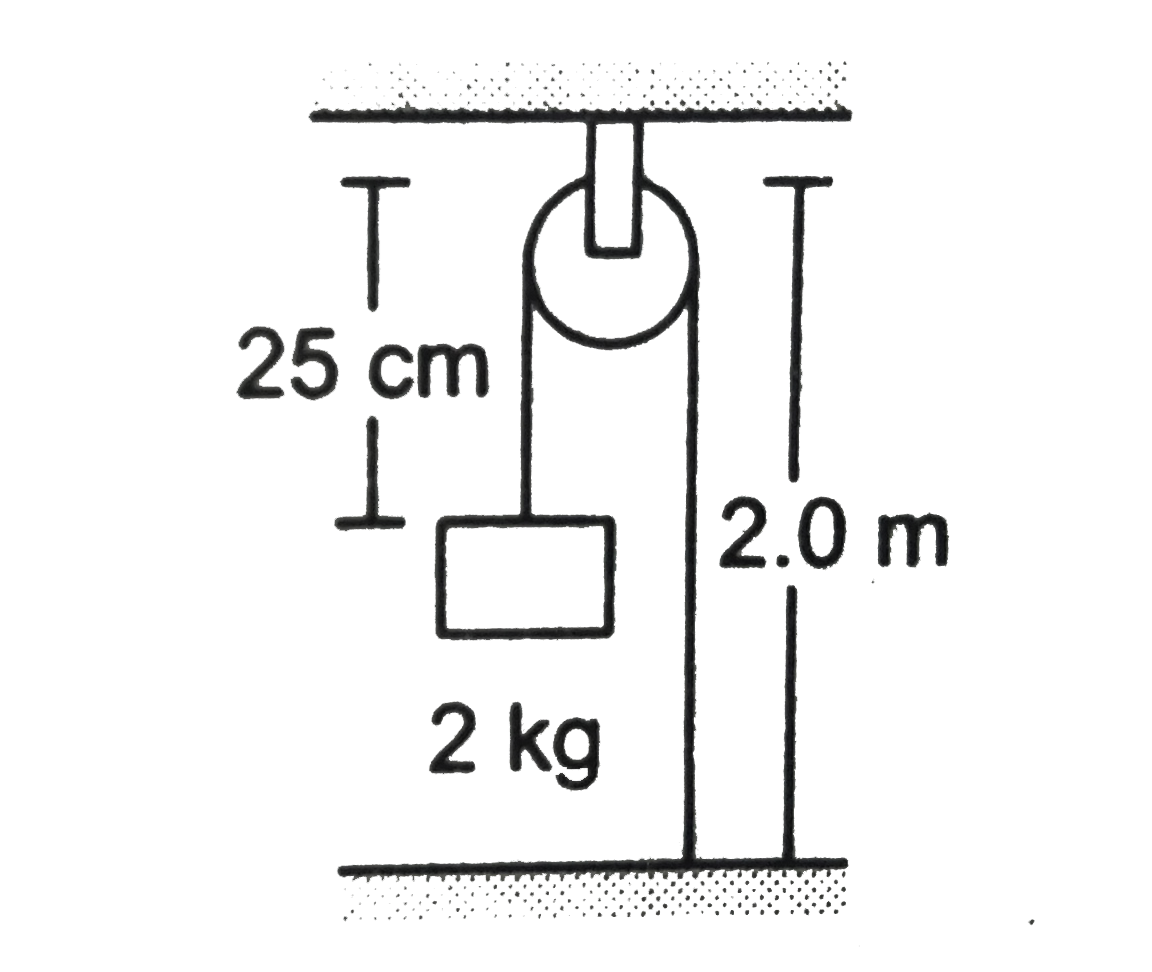 .
.
The correct Answer is:B
Total length of string =2+0.25=2.25m
Mass per unit length
m=4.5×10−32.25
=2×10−3kgm
T=2g=20N
wavespeedv=√(Tm)
sqr((202×10−3))
=√104
=102ms=100ms
Time taken to reach the pulley,
t=(sv)
=2100=0.02sec.
Topper's Solved these Questions
Similar Questions
In the arrengement shown in figure, the string has a mass of 4.5g. How much time will it take for a transverse disturbance produced at the floor to reach the pulley? Take g=10ms−2.
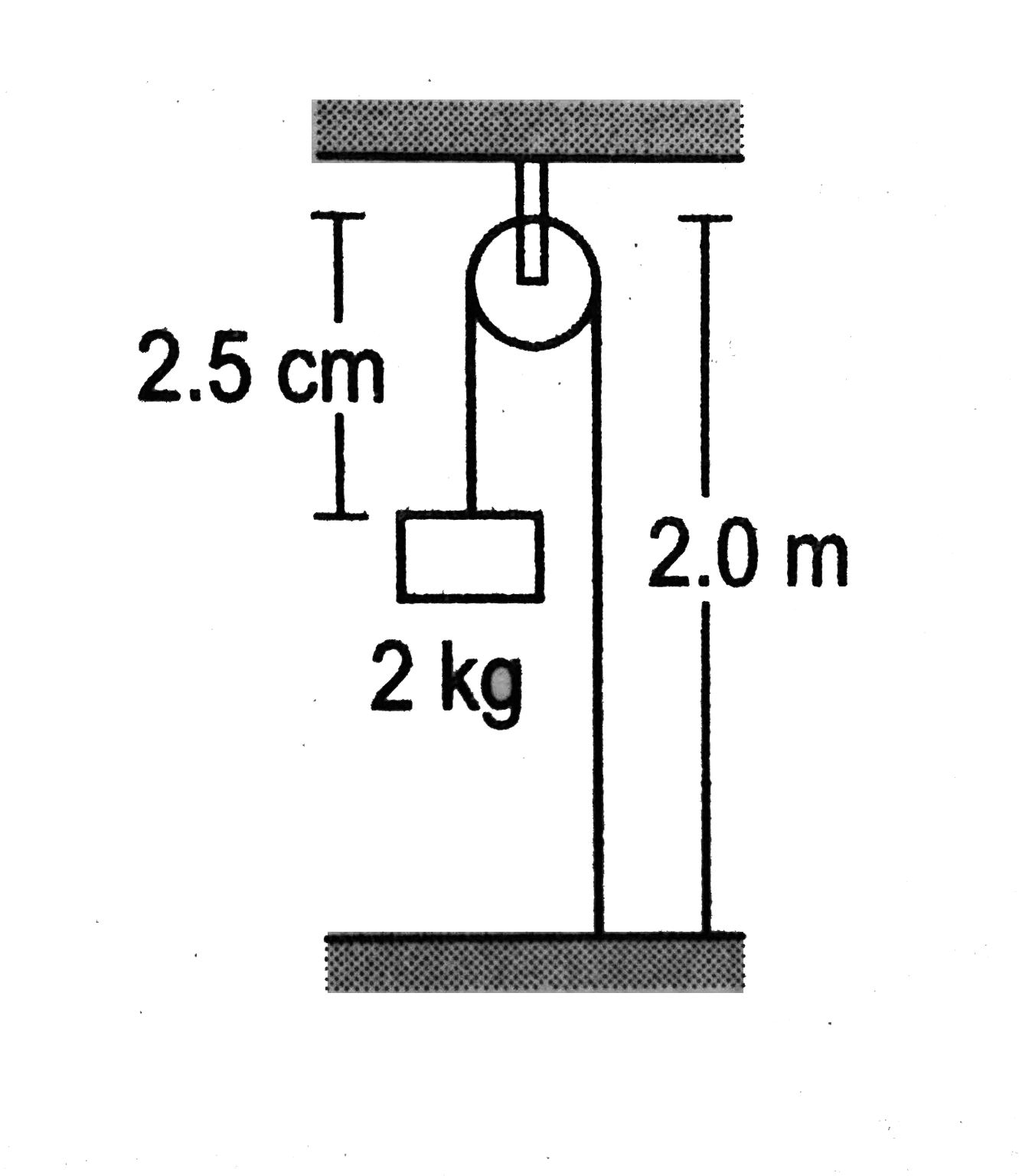
View SolutionIn the arrangement shown in figure, the string has mass of 5kg. How much time will it take for a transeverse distubance produced at the floor to reach the pulley A? Take g=10m/s2.

View Solution
Knowledge Check
In figure, the string has a mass of 14 kg. How much time will it take for a transverse disturbance produced at fixed support to reach the pulley?
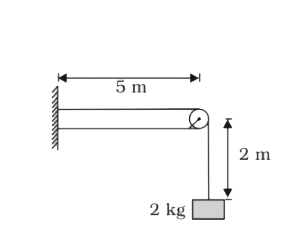 A0.91secB1.20secC1.59 secD2.35
A0.91secB1.20secC1.59 secD2.35A first order reaction has specific rate of 10−2s−1. How much time will it take for 20 g of the reactant to reduce to 5 g?
A238.6 sB138.6sC346.5 sD693.0sThe first order reaction has a specific rate 10−3s−1 How much time will it take for 10 g of the reactant to reduce to 5 g ?
A360 sB180 sC90 sD270 s
In the arrangement shown in figure, the string has mass of 5kg. How much time will it take for a transeverse distubance produced at the floor to reach the pulley A? Take g=10m/s2.

View SolutionA metal ball of mass 5 kg falls from a height of 490 m. How much time will it take to reach the ground ?
View SolutionIn the arrangement shown in figure, what should be the mass of block A so that the system remains at rest. Also find force exerted by string on the pulley Q.
(g=10m/s2)
View SolutionA first order reaction has specific rate constant of 10−3s−1. How much time will it take for 10g to reduce to half the quantity (i.e. 5g) ?
View SolutionA first order reaction has a specific reaction rate of 10−2s−1. How much time will it take for 20 g of the reactant to reduce to 5 g ?
View Solution
