A steel wire of mass 4.0 g and length 80 cm is fixed at the two ends. The tension in the wire is 50 N. Find the frequency and wavelength of the fourth harmonic of the fundamental.
The correct Answer is:frequency = 250 and λ=40cm
m=(480)gcm
=0.005kgm
T=50N
L=80cm=0.8m
v=√(Tm)
=√(500.005)=100ms
Fundamental frequency
=f=12L√(Tm)
={12×0.8×√(500.005)}
=100(2×0.8)
=1001.6=62.Hz
First =62.5Hz
f4=Frequency of forth harmonic
=4f0=f3=62.5×4
=250Hz
v=f4λ4
→(vf)=100250
=0.4m=40cm
Topper's Solved these Questions
Similar Questions
A 2.00 m-long rope, having a mass of 80 g, is fixed at one end and is tied to a light string at the other end. The tension in the string is 256 N. (a) Find the frequencies of the fundamental and the first two overtones. (b) Find the wavelength in the fundamental and the first two overtones.
View SolutionA 1m long rope, having a mass of 40g, is fixed at one end and is tied to a light string at the other end. The tension in the string in 400N. Find the wavelength in second overtone (in cm).
View Solution
Knowledge Check
A sonometer wire of length 114 cm is fixed at both the ends. Where should the two bridges be placed so as to divide the wire into three segments whose fundamental frequencies are in the ratio 1:3:4?
AAt 36 cm and 84 cm from one endBAt 24 cm and 72 cm from one endCAt 48 cm and 96 cm from one endDAt 72 cm and 96 cm from one endIf a string fixed at both ends, vibrates in its fourth harmonic, the wavelength is 15 cm. What is the length of the string ?
A20 c mB30cmC25 cmD40 cmIn a stretched wire under tension and fixed at both ends, the area of cross section of the wire is halved and the tension is doubled. The frequency of the wire will be
AtwiceBhalfCthree tiemsD3 times
A sonometer wire has a total length of 1m between the fixed ends. Where should the two bridges be placed below the wire so that the three segments of the wire have their fundamental frequencies in the ratio 1 : 2 : 3 ?
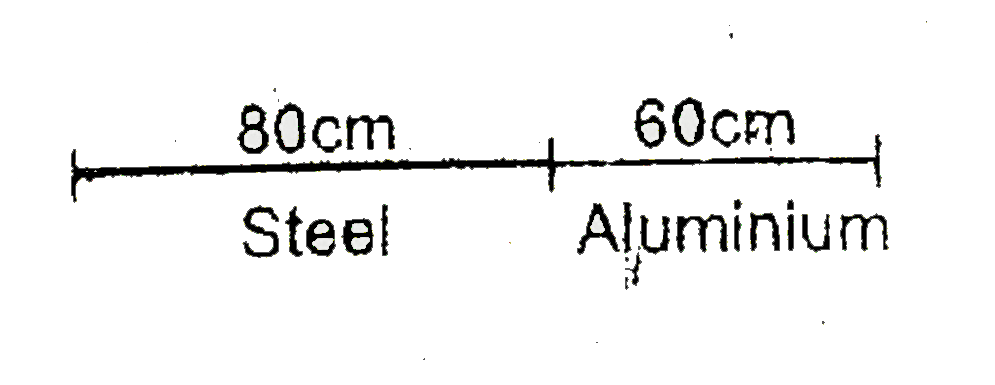
View SolutionFigure shows an aluminium wire of length 60 cm joined to a steel wire of length 80 cm and stretched between two fixed supports. The tension produced is 40 N. The cross-sectional area of the steel wire is .1.0mm2 and that of the aluminium wire is 3.0 mm 2. What could be the minimum frequency of a tuning fork which can produce standing waves in the system with the joint as a node ? The density of aluminium is 2.6gcm−3and that of steel is 7.8gcm−3
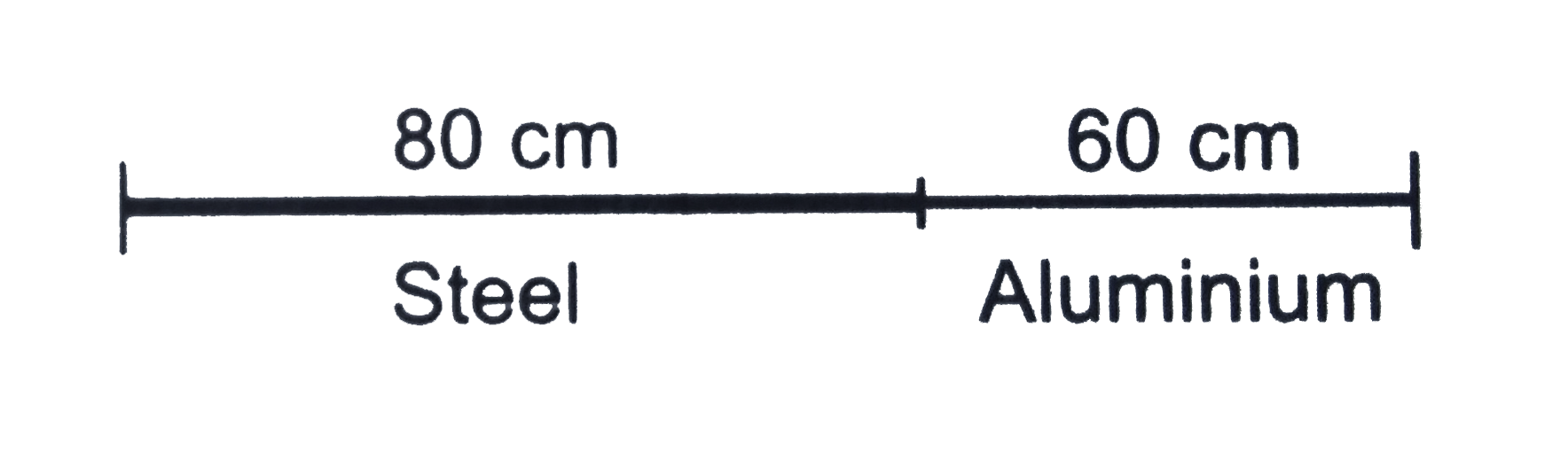
View SolutionFigure shows an aluminium wire of length 60cm joined to a steel wire of length 80cm and stretched between two fixed supports. The tension produced is 40N. The cross-sectional area of the steel wire is 1.0mm2 and that of the aluminimum wire is 3.0mm2 The minimum frequency of a tuning fork which can produce standing waves in the system with the joint as a node is 10P (in Hz) the find P. Given density of aluminimum is 2.6g/cm3 and that of steel is 7.8g/cm3.
View SolutionAn iron rod of mass 50 g and length 50 cm is suspended by master wires as shown in the figure. Calculate the magnitude and direction of magnetic field to be set in the region such that the tension is the wires is zero. The current in the rod is 4 A.

View SolutionA sonometer wire has a length of 114 cm between its two fixed ends. Where should the two briges be places so as todivide the wire into three segments, whos fundamental frequencies are in the ratio 1:3:4?
View Solution
