Let I= current through a conductor, R= its resistance and V= potential difference across its ends. According to Ohm's law, product of two of these quantities equals the third. Obtain Ohm's law from dimensional analysis. Dimensonal formulae for R andV are ML2I−2T−3andML2T−3I−1 respectively.
DimensioN/Al formule of R =[ML2II^2T−3],DimensioNAslforμlaeofV=[ML^2I^-2T^-3]. DimensioN/Al formulae of [ML2I−1T−3]=[ML2T−2][I],[ML2I−IT−3]=[ML2T−3I−2][I],→V=IR
Topper's Solved these Questions
Similar Questions
Let I = current through a conductor, R =its resistance and V = potential difference across its ends. According to Ohm's law, product of two of these quantites equals the third. Obtain Ohm's law from dimensional analysis. Dimensional formula for R and V are [ML2T−3A−2]and[ML2T−2A−1] respectively.
View SolutionIf a current of 3 A flows through a wire of resistance 8 ohms, calculate the potential difference applied across its ends.
View Solution
Knowledge Check
When a current I is passed through a wire of constant resistance, it produces a potential difference V across its ends. The graph drawn between log I and log V will be
A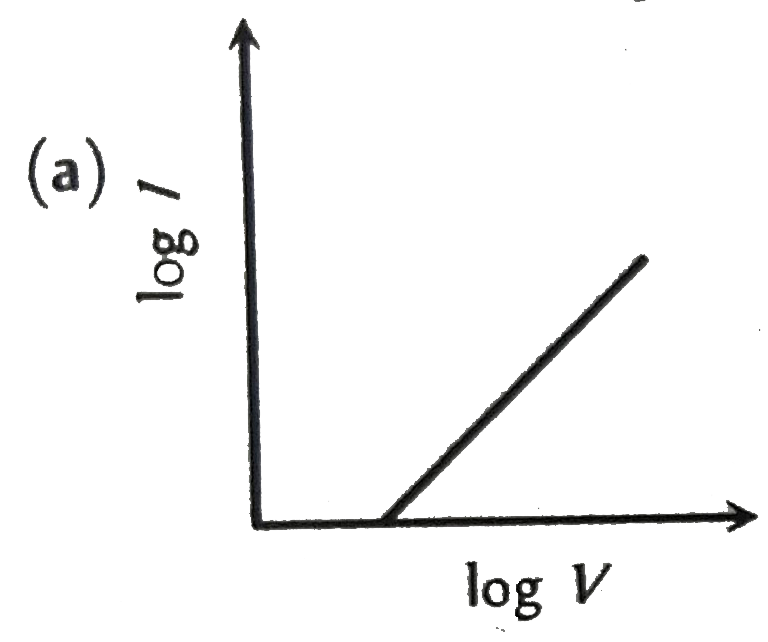 B
B C
C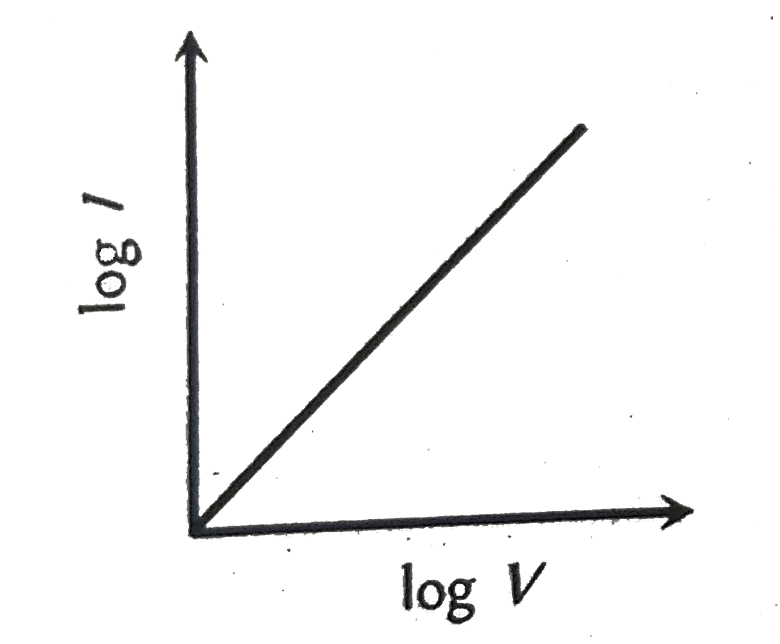 D
D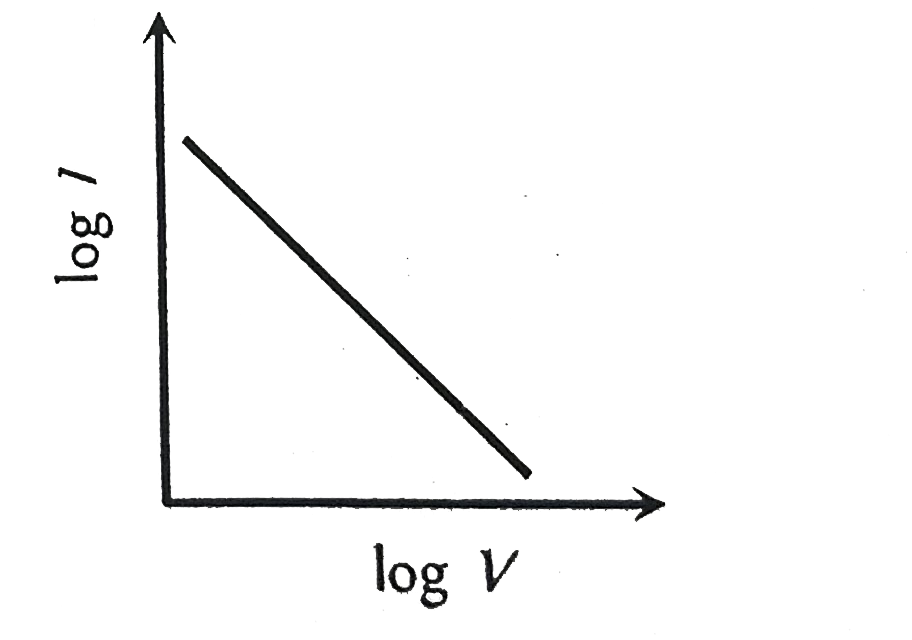
Imagine a conductor through which a current I is flowing and let V be the potential difference between the ends of the conductor. Then, Ohm’s law states that
AV∞lBV = RICV = 2 RIDBoth (1) and (2)A current of 2.0 ampere passes through a cell of e.m.f. 1.5 volts having internal resistance of 0.15 ohm. The potential difference measured, in volts, across both the ends of the cell will be
A1.35B1.50C1.00D1.20
The current flowing through a conductor and the potential difference across its two ends are as per reading of the ammeter and the voltmeter shown in (Fig. 3.50). Find the resistance of the conductor.
 .
.
View SolutionThe heat produced in a wire carrying an electric current depends on the current, the resistance and the time. Assuming that the dependuance is of the product of powers type, guress an eqn. between these quantites uning dimesional analysis. The dimensional formula of resistance is ML2A−2T−3 and heat is a form of energy.
View SolutionThe rate of Joule heat is given by P=V×I where V is the potential difference across the ends of a conductor and I is the current flowing through it. Does this relation hold for a condutor that does not obey Ohm's law?
View SolutionThe following tables gives the current i (in amperes) through two devices for several values of potential difference V (in volts). From these data, determine which device does not obey Ohm's law.

View SolutionIn figure i1=10e−2tA,i2=4A,vC=3e−2tV
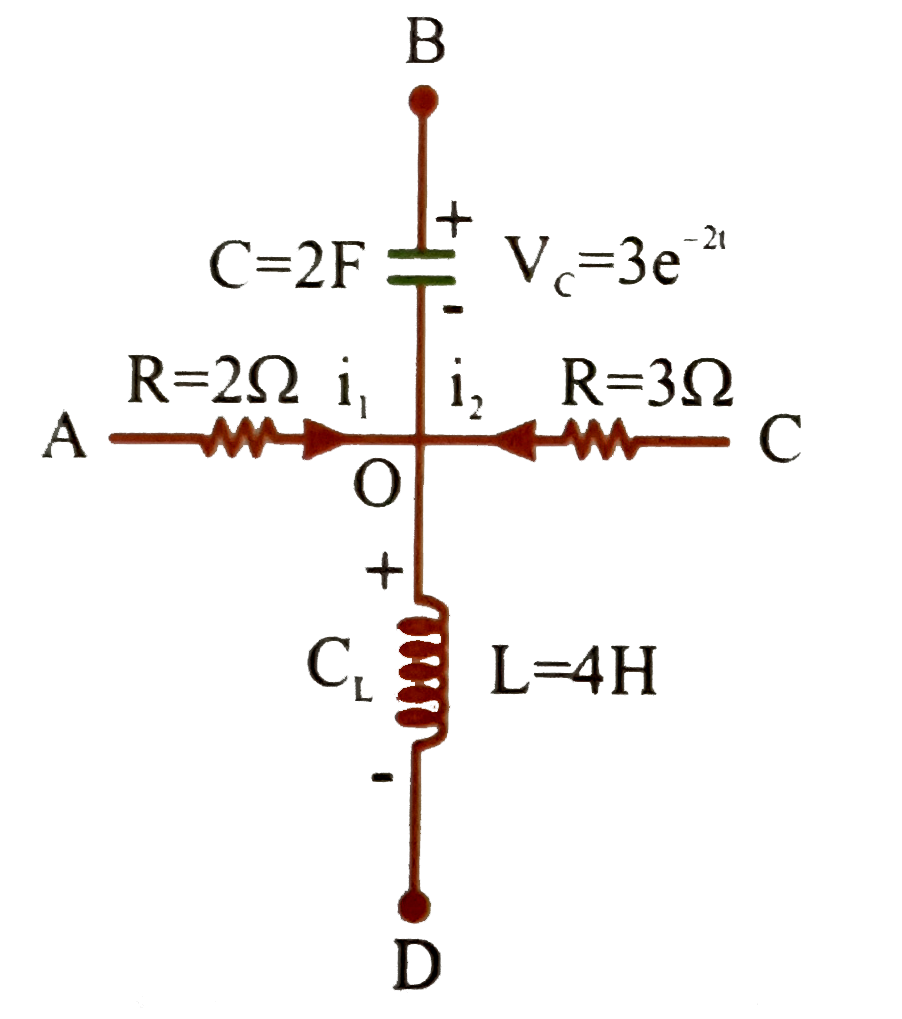
The potential difference across inductor VL is :
View Solution
