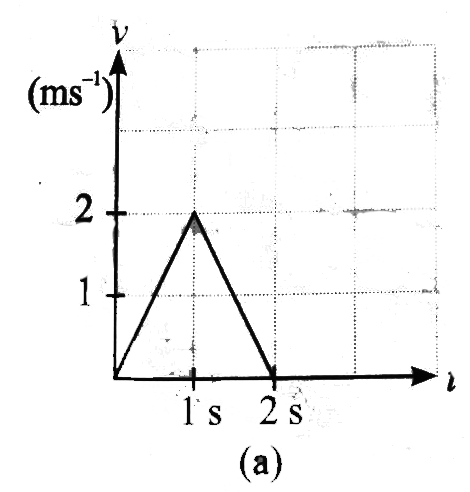

Figure shows (vx,t) and (vy,t) diagram for a body of unit mass. Find the force as a function of time.
Consider figure(a) vx=2t for 0<t<1s
= 2(2−t) for 1<t<1s
= 0 for t > 2s.
From figure (b) vy=t for 0<t<1s
= 1 for t > 1s
Fx=max=mdvxdt
=1×2 for0<t<1s
=1(−2) for1<t<2s
for2<t

Fy=may=mdvydt
=1×1 for 0<t<1s=0 for 1<t
Hence, F=Fxˆi+Fyˆj
=2ˆi+ˆj for0<t<1s
=−2ˆi for 1<t<2s
=0 for t>2s
Topper's Solved these Questions
- LAWS OF MOTIONBOOK - NCERT EXEMPLAR ENGLISHCHAPTER - LAWS OF MOTIONEXERCISE - Short answer type questions
6 Videos
- KINETIC THEORYBOOK - NCERT EXEMPLAR ENGLISHCHAPTER - KINETIC THEORYEXERCISE - Multiple Choice Questions (MCQs)
31 Videos
- MECHANICAL PROPERTIES OF FLUIDSBOOK - NCERT EXEMPLAR ENGLISHCHAPTER - MECHANICAL PROPERTIES OF FLUIDSEXERCISE - Long Answer Type Questions
3 Videos
Similar Questions
Knowledge Check
Figure shows (x,t) (y,t) diagram of a particle moving in 2-dimensions.
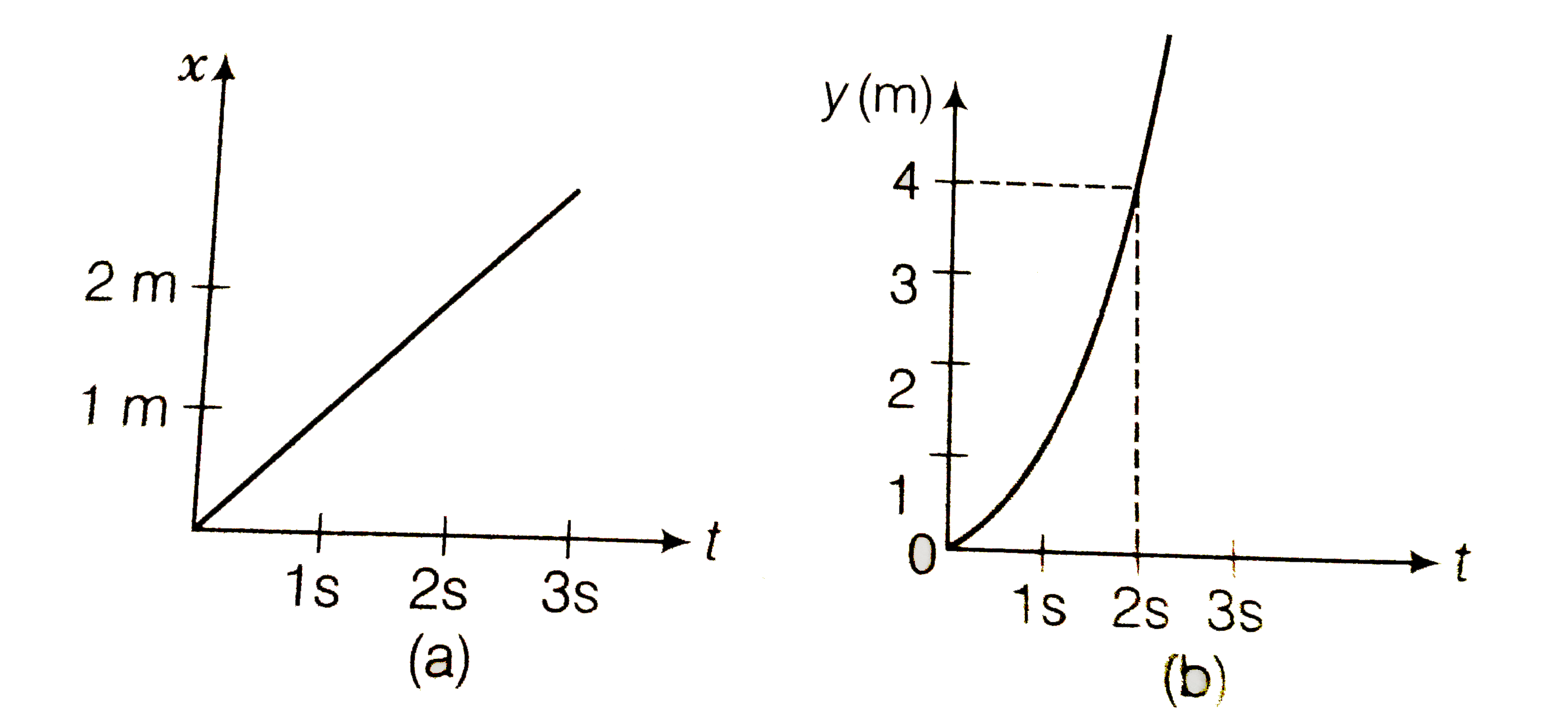
If the particle has a mass of 500 g , find the force (direction and magnitude) acting on the particle .A1N along y-axisB1N along x-axisC0.5N along x-axisD0.5N along y-axis
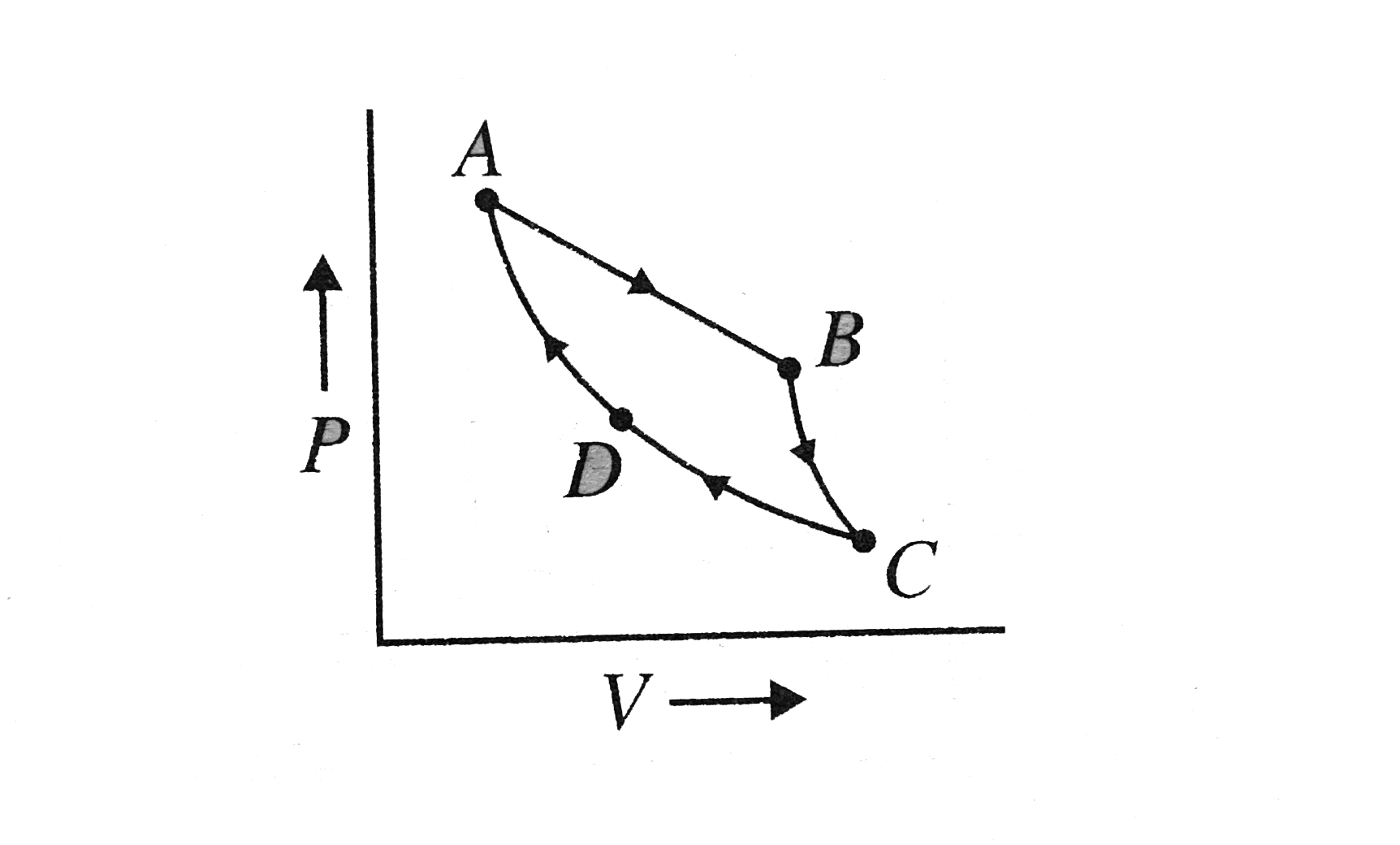
Figure. Shows the P−V diagram for a Carnot cycle. In this diagram
View SolutionWork done in time t on a body of mass m which is accelerated from rest to a speed v in time t1 as a function of time t is given by
View SolutionThe graph in figure shows the velocity v versus time t for a body. Which of the graphs shown in figure represents the correesponding acceleration versus time graphs ?
View SolutionA body of mass m is acceleratad uniformaly from rest to a speed v in a time T . The instanseous power delivered to the body as a function of time is given by
View Solution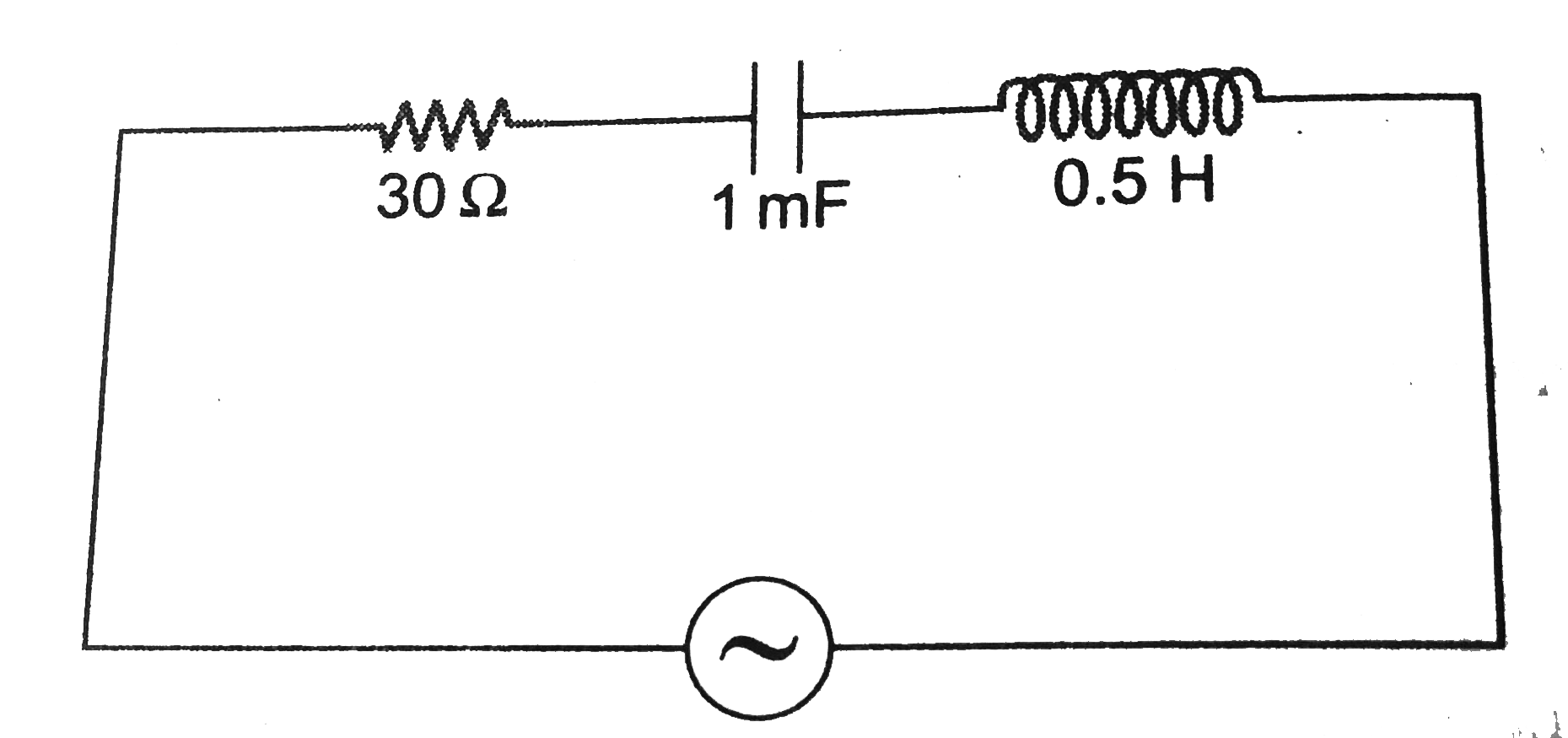
In the diagram shown in figure, V function is given. Find other four functions of time I,VC,VR and VL. Also, find power consumed in the circuit, V is given in volts and ω in rad/s.
View SolutionA body of mass m, accelerates uniformly from rest to V1 in time t1. The instantaneous power delivered to the body as a function of time t is.
View SolutionThe motion of a body is given by the equation dvdt=4−2v, where v is the speed in m/s and t in second. If the body was at rest at t = 0, then find
(i) The magnitude of initial acceleration
(ii) Speed of body as a function of time
View Solution