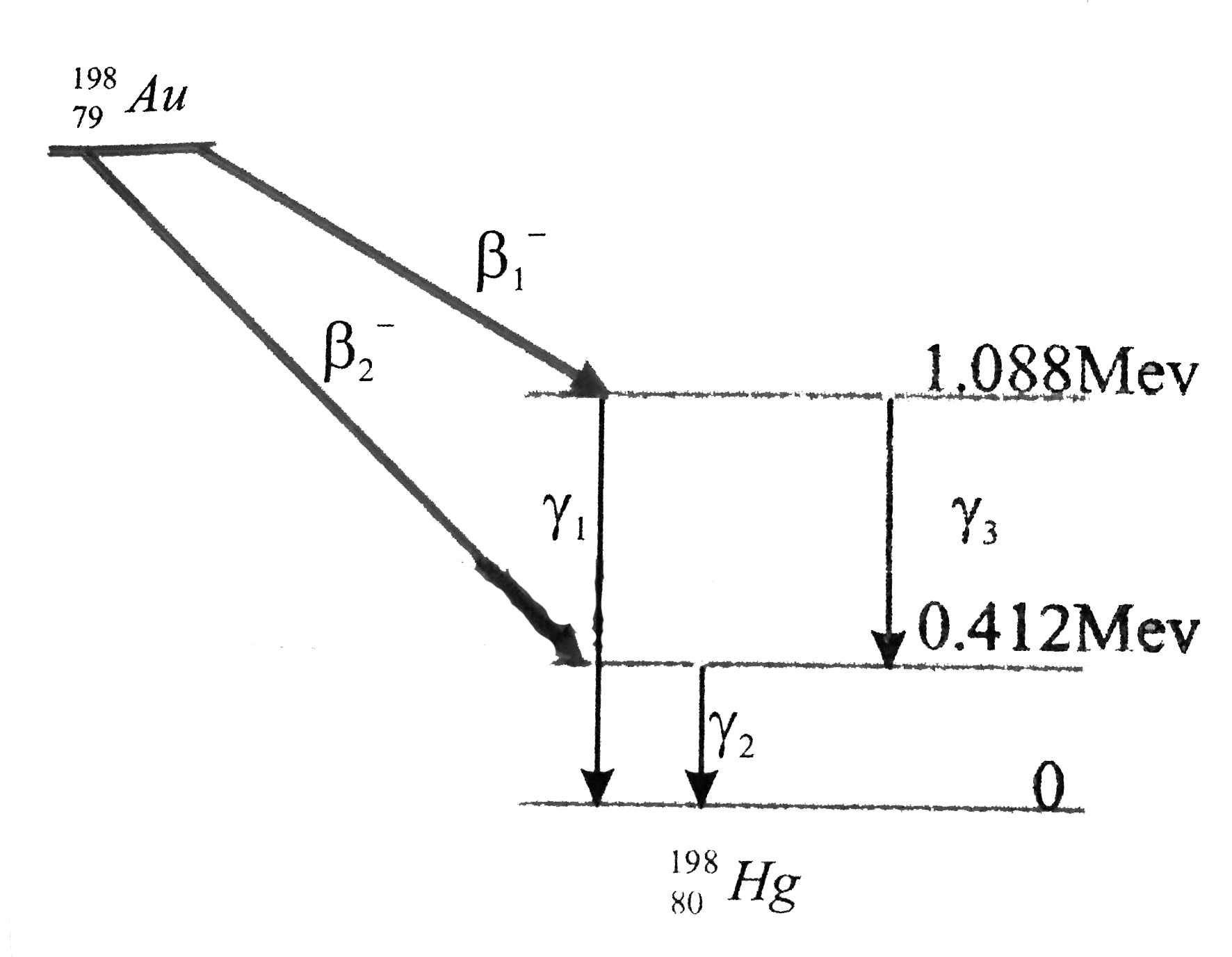
Obtain the maximum kinetic energy of β- particle and the radiation frequencies corresponding to γ− decays in the figure given below. (rest mass of au -198.968233 u and r.m. of Hg=198=197.966760 u)
Energies of γ- photons γ1,γ2,γ3 are
E1=(1.088−0)MeV=1.088MeV=1.088×1.6×10−13=1.7408×10−13J
E2=(0.412−0)MeV=0.412MeV=0.412MeV=0.412×1.6×10−13=0.6592×10−13J
E3=(1.088−0.452)MeV=0.676MeV=0.672×1.6×10−13=1.0816×10−13J
but frequency of radiation v=Eh

∴ v1=1.7408×10−136.625×10−34=0.2628×1021Hz=2.628×1024Hz
v2=0.6592×10−136.625×10−34=0.0955×1021Hz=9.55×1019Hz
v3=1.0816×10−136.625×10−34=0.1633×1021Hz=1.633×20Hz
β−1 decay, _(79)198Au→19880Hg+0−1e+Q2(¯β1)+Q1(γ1)
The maximum kinetic energy of β−1=[197.968233−197.966760]×931.5MeV=0.284MeV
β−2 decay, _(79)198Au→19880Hg+0−1e+Q2(¯β2)+Q2(γ2)
The maximum kinetic energy of β−2=[197.96822233−197.966760]×931.5MeV−0.412MeV=0.96MeV
Topper's Solved these Questions
- NUCLEIBOOK - SUBHASH PUBLICATIONCHAPTER - NUCLEIEXERCISE - FIVE MARKS QUESTIONS WITH ANSWERS
10 Videos
- MOVING CHARGES AND MAGNETISMBOOK - SUBHASH PUBLICATIONCHAPTER - MOVING CHARGES AND MAGNETISMEXERCISE - NUMERICALS WITH SOLUTIONS
23 Videos
- PUE BOARD MODEL QUESTION PAPER 1BOOK - SUBHASH PUBLICATIONCHAPTER - PUE BOARD MODEL QUESTION PAPER 1EXERCISE - QUESTION
41 Videos
Similar Questions
Obtain the maximum kinetic energy of β-particles, and the radiation frequencies of γ decays in the decay scheme shown in Fig. 14.6. You are given that m(.198Au)=197.968233u,m(.198Hg)=197.966760u
View SolutionObtain the maximum kinetic energy of β-particles, and the radiation frequencies of γ decays in the decay scheme shown in Fig. 14.6. You are given that m(.198Au)=197.968233u,m(.198Hg)=197.966760u

View Solution
Knowledge Check
The kinetic energy of α-particle emitted in the α-decay of 88Ra266 is [given, mass number of Ra = 222 u]
A5.201 Me VB3.301 Me VC6.023 Me VD4.871 Me V
Calculate the kinetic energy of β-particles and the radiation frequencies corresponding to the γ-decays shown in figure.
Given, mass of .12Mg27 atom =26.991425 amu and mass of .13Al27 atom = 26.990080 amu
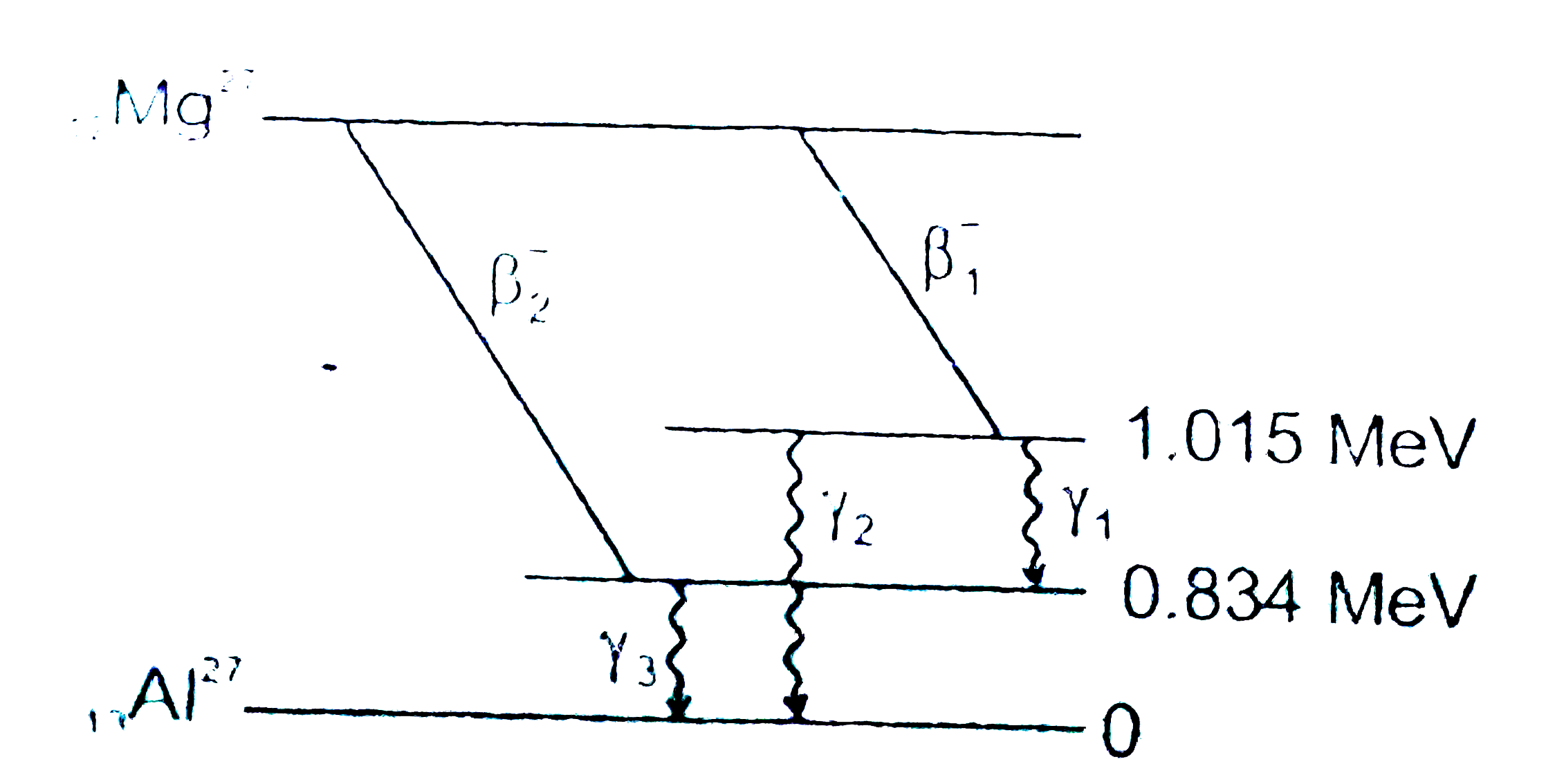
View SolutionCalculate the kinetic energy of β-particles and the radiation frequencies corresponding to the γ-decays shown in figure.
Given, mass of .12Mg27 atom =26.991425 amu and mass of .13Al27 atom = 26.990080 amu

View SolutionConsider the beta decay
^198Au→198Hg∗+Bη−1+→v.
where ^198Hg∗ represents a mercury nucleus in an excited state at energy 1.088MeV above the ground state. What can be the maximum kinetic energy of the electron emitted? The atomic mass of ^198Au is 197.968233u and that of ^198Hg is 197.966760u.
View SolutionConsider the beta decay
^198Au→198Hg∗+Bη−1+→v.
where ^198Hg∗ represents a mercury nucleus in an excited state at energy 1.088MeV above the ground state. What can be the maximum kinetic energy of the electron emitted? The atomic mass of ^198Au is 197.968233u and that of ^198Hg is 197.966760u.
View SolutionFind the maximum energy that a beta particle can have in the following decay
^176Lu→176Hf+e+→v.
Alomic mass of ^176Lu is 175.942694u and that of ^176Hf is 175.941420u.
View SolutionFind the maximum energy that a beta particle can have in the following decay
^176Lu→176Hf+e+→v.
Alomic mass of ^176Lu is 175.942694u and that of ^176Hf is 175.941420u.
View SolutionA nucleus 10Ne23 undergoes β- decay and becomes 11Na23 . Calculate the maximum kinetic energy of electrons emitted assuming that the daughter nucleus and antineutrino carry negligible kinetic energy. Given mass of 10Ne23 = 22.994466 u and mass of 11Na23 = 22.989770 m
View Solution
