On heating one end of a rod the temperature of the whole rod will be uniform when .
K = 1
K = 0
K = 100
K = oo
The correct Answer is:D
To determine when the temperature of the whole rod will be uniform upon heating one end, we can follow these steps:
Step 1: Understand the concept of thermal conductivity
Thermal conductivity (K) is a measure of a material's ability to conduct heat. For a rod to have a uniform temperature throughout, it must conduct heat perfectly.
Hint: Recall that thermal conductivity is a property of materials that indicates how well they can transfer heat.
Step 2: Define the conditions for uniform temperature
For the temperature to be uniform across the rod, the heat supplied must be distributed evenly throughout the rod. This means that the temperature difference (dT) between any two points in the rod must be zero.
Hint: Think about what it means for temperature to be uniform. If dT = 0, then every point in the rod is at the same temperature.
Step 3: Apply the heat conduction equation
The heat conduction equation is given by:
Q=K⋅A⋅dTdx
where:
- Q is the heat transferred,
- K is the thermal conductivity,
- A is the cross-sectional area,
- dTdx is the temperature gradient.
For uniform temperature, dT=0, which leads to:
Q=K⋅A⋅0
This means that no heat is lost or gained across the rod.
Hint: Consider what happens to the equation when dT approaches zero.
Step 4: Analyze the implications of dT = 0
If dT=0, then the equation implies that the thermal conductivity K must be infinite for the heat transfer to occur without any temperature difference. This is a theoretical scenario, as no real material can achieve infinite thermal conductivity.
Hint: Think about the physical meaning of infinite thermal conductivity. What would that imply about the material?
Step 5: Conclusion
Thus, for the temperature of the whole rod to be uniform when one end is heated, the thermal conductivity of the rod must be infinite. In practical terms, this means that the rod would need to be an ideal conductor of heat.
Hint: Reflect on the options given in the question and select the one that corresponds to this conclusion.
Final Answer
The temperature of the whole rod will be uniform when the thermal conductivity K is infinite.
Thermal conductivity (K) is a measure of a material's ability to conduct heat. For a rod to have a uniform temperature throughout, it must conduct heat perfectly.
For the temperature to be uniform across the rod, the heat supplied must be distributed evenly throughout the rod. This means that the temperature difference (dT) between any two points in the rod must be zero.
The heat conduction equation is given by:
Q=K⋅A⋅dTdx
where:
- Q is the heat transferred,
- K is the thermal conductivity,
- A is the cross-sectional area,
- dTdx is the temperature gradient.
Q=K⋅A⋅0
This means that no heat is lost or gained across the rod.
If dT=0, then the equation implies that the thermal conductivity K must be infinite for the heat transfer to occur without any temperature difference. This is a theoretical scenario, as no real material can achieve infinite thermal conductivity.
Thus, for the temperature of the whole rod to be uniform when one end is heated, the thermal conductivity of the rod must be infinite. In practical terms, this means that the rod would need to be an ideal conductor of heat.
The temperature of the whole rod will be uniform when the thermal conductivity K is infinite.
Topper's Solved these Questions
- CALORIMETRY AND HEAT TRANSFERBOOK - DC PANDEY ENGLISHCHAPTER - CALORIMETRY AND HEAT TRANSFEREXERCISE - Check points 16.4
29 Videos
- CALORIMETRY AND HEAT TRANSFERBOOK - DC PANDEY ENGLISHCHAPTER - CALORIMETRY AND HEAT TRANSFEREXERCISE - Taking it together
51 Videos
- CALORIMETRY AND HEAT TRANSFERBOOK - DC PANDEY ENGLISHCHAPTER - CALORIMETRY AND HEAT TRANSFEREXERCISE - Check point 16.2
10 Videos
- CALORIMETRY & HEAT TRANSFERBOOK - DC PANDEY ENGLISHCHAPTER - CALORIMETRY & HEAT TRANSFEREXERCISE - Level 2 Subjective
14 Videos
- CENTRE OF MASSBOOK - DC PANDEY ENGLISHCHAPTER - CENTRE OF MASSEXERCISE - Medical entrances gallery
27 Videos
Similar Questions
A thin rod of negligible mass and area of cross section S is suspended vertically from one end. Length of the rod is L0 at T∘C . A mass m is attached to the lower end of the rod so that when temperature of the rod is reduced to 0∘C its length remains L0 Y is the Young’s modulus of the rod and α is coefficient of linear expansion of rod. Value of m is :
View SolutionAssertion : Heat is supplied at constant rate from one end of a conducting rod. In steady state, temperature of all points of the rod becomes uniform.
Reason : In steady state, temperature of rod does not increase.
View Solution
Knowledge Check
Three metal rods of the same material and identical in all respect are joined as shown in the figure. The temperatures at the ends are maintained as indicated. Assuming no loss of heat from the curved surface of the rods, the temperature at the junction X would be
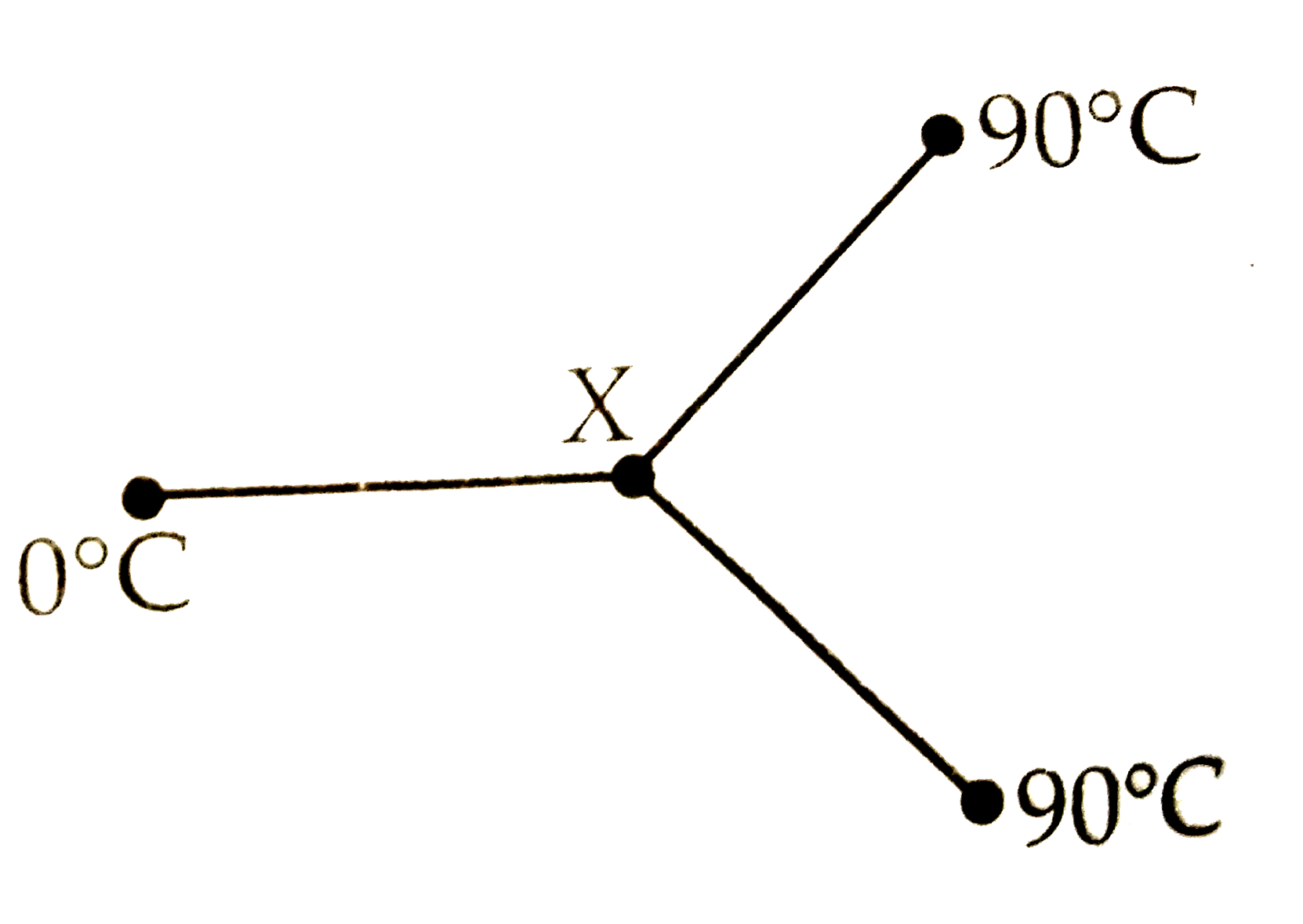 A45∘CB60∘CC30∘CD20∘C
A45∘CB60∘CC30∘CD20∘C
The temperature gradient in a rod of 0.5m length is 80∘C/m. It the temperature of hotter end of the rod is 30∘C, then the temperature of the cooler end is
View SolutionAssertion A metallic rod is fixed from two ends as shown in figure. When the temperature is increased compressive stresses are developed in the rod.
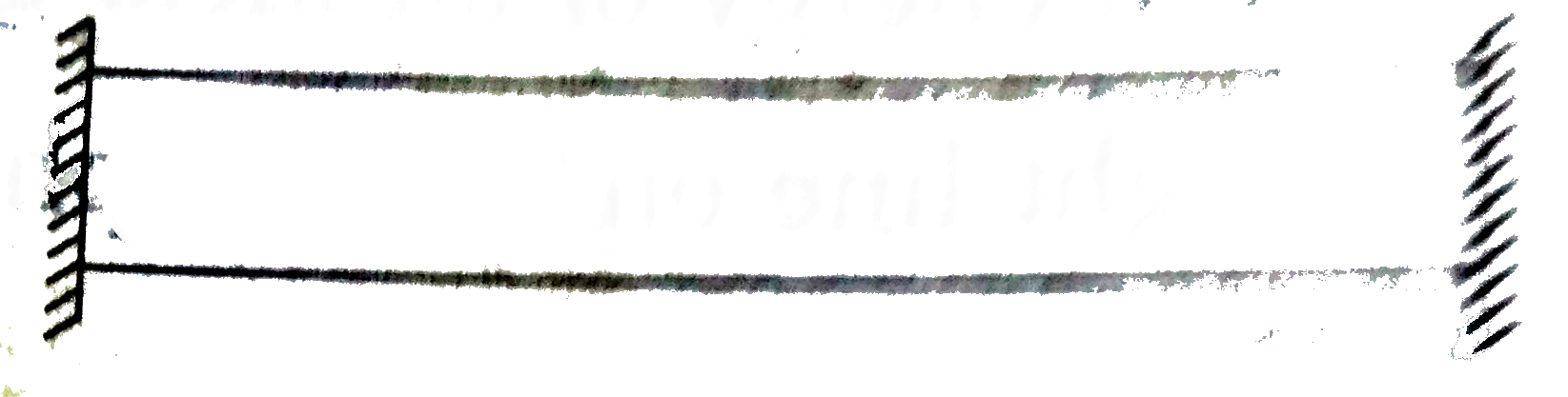
Reason At higher temperature, natural length of the rod will be more.
View SolutionA brass rod of length 1m is fixed to a vertical wall at one end, with the other end keeping free to expand. When the temperature of the rod is increased by 120∘C, the length increases by 3cm. What is the strain?
View SolutionOne end of a rod of length L and crosssectional area A is kept in a furnace at temperature T1. The other end of the rod is kept at a temperature T2. The thermal conductivity of the matrieal of the rod is K and emissivity of the rod is e. It is gives that T!=Ts+ΔT, where ΔT<<Ts,Ts is the temperature of the surroundings. If ΔT∝(T1−T2) find the proportional constant, consider that heat is lost only by rediation at the end where the temperature of the rod is T1.

View SolutionA uniform rod is placed vertically on a smooth surface and then released . Then,
View SolutionA slender rod of mass M and length L rests on a horizontal frictionless surface. The rod is pivoted about one of ends. The impulse of the force exerted on the rod by the pivot when the rod is struck by a blow of impulse J perpendicular to the rod at other end is
View SolutionTwo similar rods are joined as shown in figure. Then temperature of junction is (assume no heat loss through lateral surface of rod and temperatures at the ends are shown in steady state )
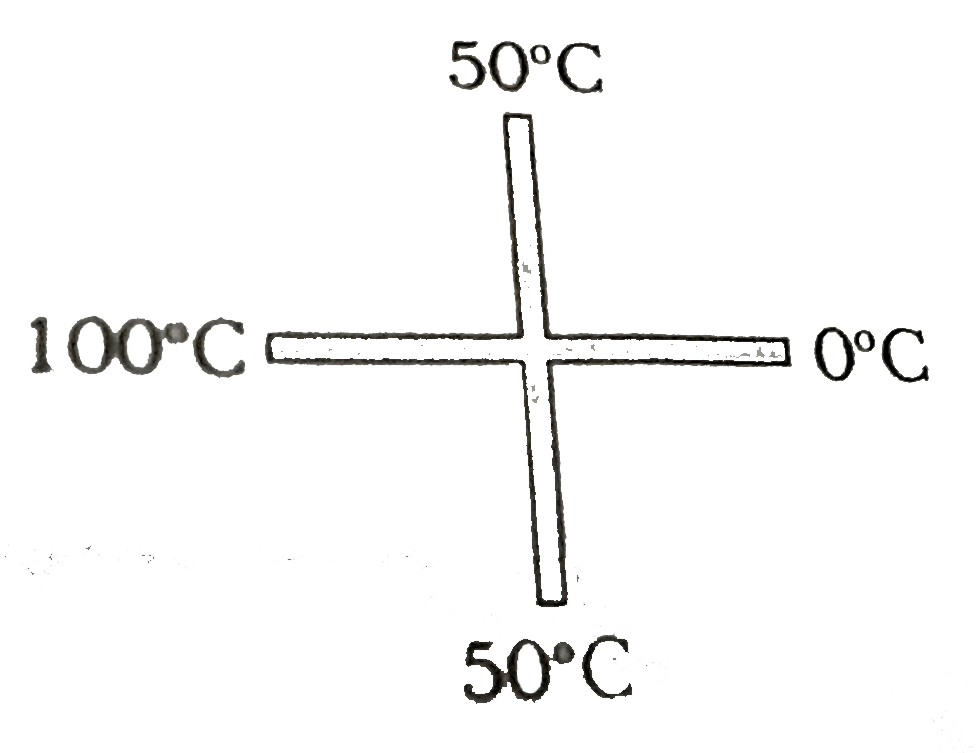
View Solution
