One mole of an ideal gas with the adiabatic exponent γ goes through a polytropic process as a result of which the absolute temperature of the gas increases τ−fold. The polytropic constant equal n. Find the entropy increment of the gas in this process.
For the polytropic process with index n
pVn=constant
Along this process (Sec 2.122)
C=R(1γ−1−1n−1)=n−γ(γ−1)(n−1).R
So ΔS=∫τT0T0CdTT=n−γ(γ−1)(n−1)R1nτ.
Topper's Solved these Questions
- THERMODYNAMICS AND MOLECULAR PHYSICSBOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - THERMODYNAMICS AND MOLECULAR PHYSICSEXERCISE - Liquids Capillary Effects
25 Videos
- THERMODYNAMICS AND MOLECULAR PHYSICSBOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - THERMODYNAMICS AND MOLECULAR PHYSICSEXERCISE - Phase Transformations
35 Videos
- THERMODYNAMICS AND MOLECULAR PHYSICSBOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - THERMODYNAMICS AND MOLECULAR PHYSICSEXERCISE - Kinetic Theory Of Gases
51 Videos
- PHYSICAL FUNDAMENTALS OF MECHANICSBOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - PHYSICAL FUNDAMENTALS OF MECHANICSEXERCISE - Relativistic Mechanics
49 Videos
Similar Questions
An ideal gas with the adiabatic exponent γ goes through a cycle (Fig. 2.3) within which the absolute temperature varies τ−fold. Find the efficiency of this cycle.
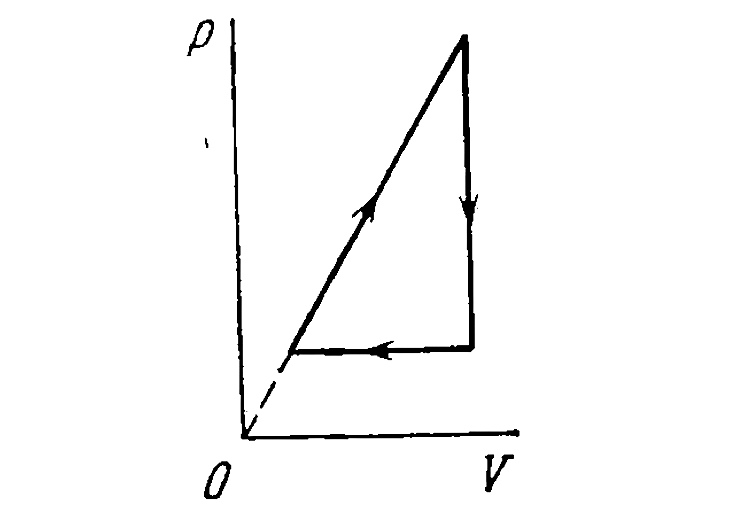 .
.
View SolutionFind the molar heat capacity of an ideal gas with adiabatic exponent γ for the polytorpic process PVn= Constant.
View Solution
Knowledge Check
The efficiency of an ideal gas with adiabatic exponent 'γ' for the shown cyclic process would be
 A(2ln2−1)γ/(γ−1)B(1−2ln2)γ/(γ−1)C(2ln2+1)γ/(γ−1)D(2ln2−1)γ/(γ+1)
A(2ln2−1)γ/(γ−1)B(1−2ln2)γ/(γ−1)C(2ln2+1)γ/(γ−1)D(2ln2−1)γ/(γ+1)The efficiency of an ideal gas with adiabatic exponent 'γ' for the shown cyclic process would be
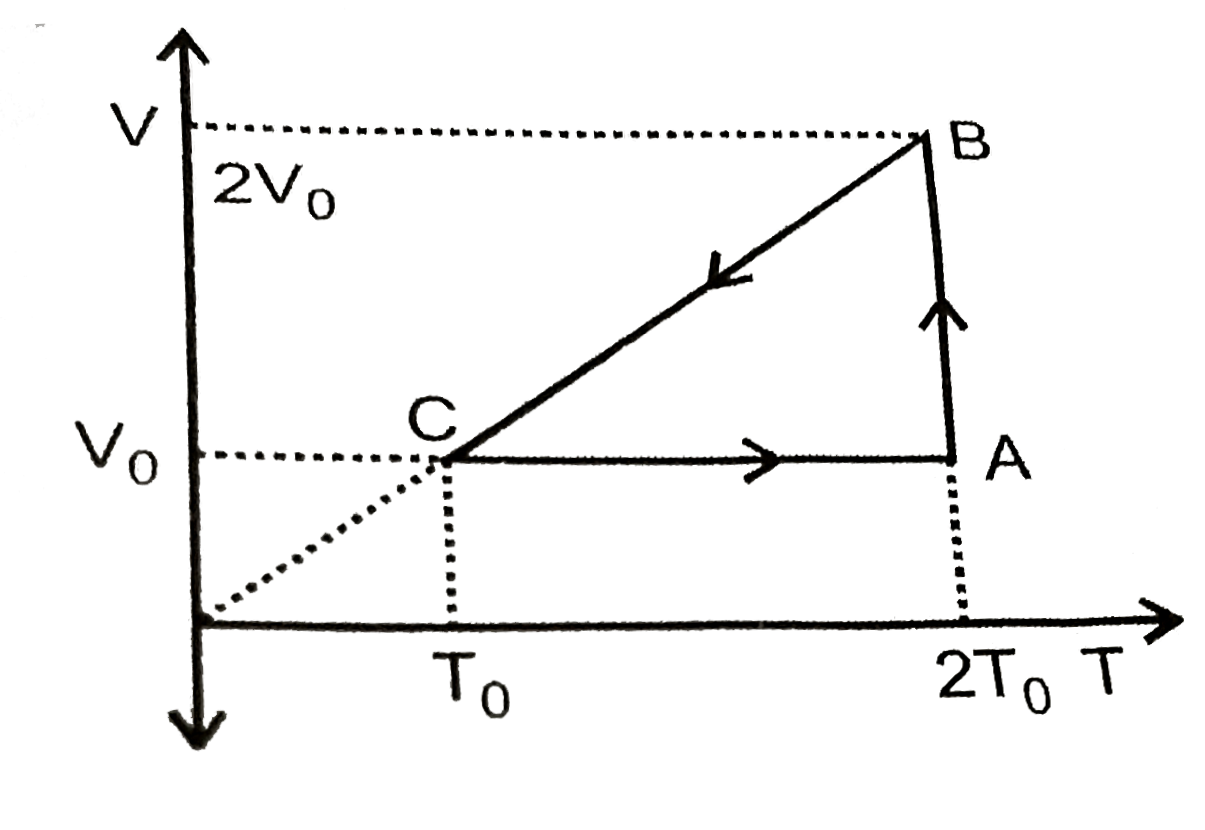 A(γ−1)(2 ln 2−1)1+(γ−1)2 ln 2B(γ−1)(1−2 ln 2)(γ−1)2 ln 2−1C(2 ln 2+1)(γ−1)(γ−1)2 ln 2+1D(2 ln 2−1)γ/(γ−1)
A(γ−1)(2 ln 2−1)1+(γ−1)2 ln 2B(γ−1)(1−2 ln 2)(γ−1)2 ln 2−1C(2 ln 2+1)(γ−1)(γ−1)2 ln 2+1D(2 ln 2−1)γ/(γ−1)One mole of an ideal monoatomic gas is taken through a polytropic process P2T = constant. The heat required to increase the temperature of the gas by ΔT is
ARΔTB3RΔTC5RΔTD6RΔT
One mole of an ideal gas with adiabatic exponent γ undergoes the process
(a) P=P0+αV
(b) T=T0+αV
Find Molar heat capacity of the gas as a function of its volume.
View SolutionFind the molar heat capacity of an ideak gas in a polytropic process pVn=const if the adiabatic exponent of the gas is equal to γ. At what values of the polytropic constant n will the heat capacity of the gas be negative ?
View SolutionIn the polytropic process PV2 = constant, is the gas cooled'or heated with increase in volume.
View Solution4 moles of an ideal monoatomic gas is heated isobarically so that its absolute temperature increases 2 times. Then the entropy increment of the gas in this process is
View SolutionAn ideal gas with adiabatic exponent γ is heated at constant pressure. It absorbs Q amount of heat. Fraction of heat absorbed in increasing the temperature is
View Solution
