P is a fixed smooth cylinder of radius R and Q is a disc of mass M and radius R. A light thread is tightly wound on Q and its end is connected to a rope ABC. The rope has a mass m and length πR2 and is initially placed on the cylinder with its end A at the top. The system is released from rest. The rope slides down the cylinder as the disc rolls without slipping. The initial separation between the disc and the cylinder was L=πR2 (see fig). Find the speed with which the disc will hit the cylinder. Assume that the rope either remains on the cylinder or remains vertical, it does not fly off the cylinder.
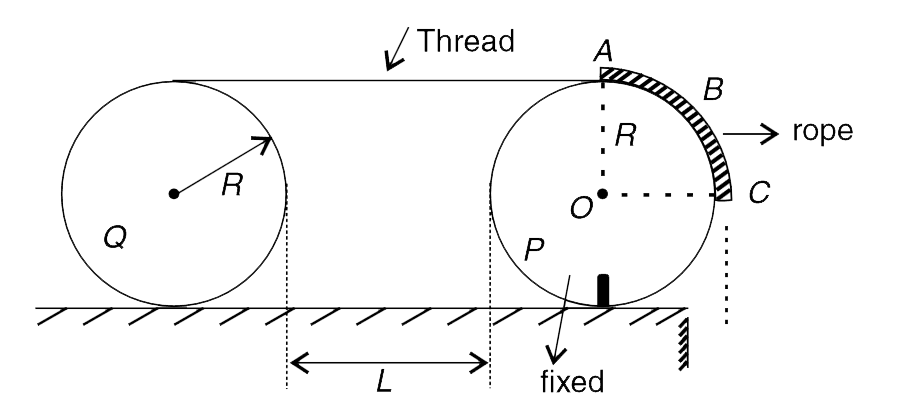
The correct Answer is:V=√(8+3π2π)(mgR3M+8m)
Topper's Solved these Questions
Similar Questions
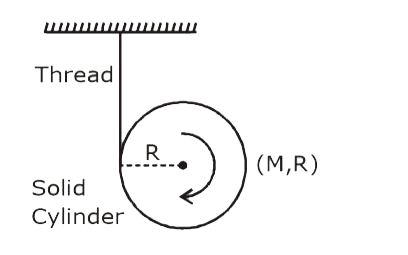
Thread would around cylinder of mass M and radius R. It is allowed to fall as shown. Find its acceleration.
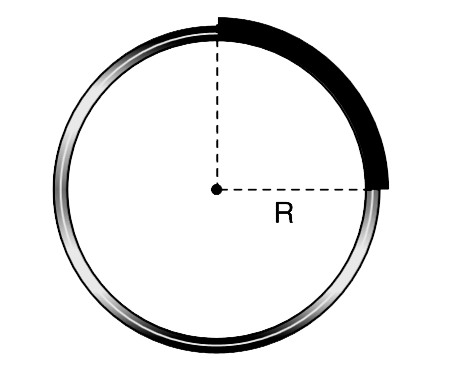
View SolutionA uniform rope of length πR2 has been placed on fixed cylinder of radius R as shown in the fig. One end of the rope is at the top of the cylinder. The coefficient of friction between the rope and the cylinder is just enough to prevent the rope from sliding. Mass of the rope is M.
(a) At what position, the tension in the rope is maximum?
(b) Calculate the value of maximum tension in the rope.
View Solution
Knowledge Check
A scylindrical rod of mass M, length L & radius R has cords wound around it , whose ends are attached to the ceioling .The rod is held borizontal and released .The liinear acceleration of the cylinder as it falls and tension in the cords is
Ag,Mg/2Bg/3,Mg/3C2g/3,Mg/6Dg/2,Mg/12A uniform solid cylinder of mass M and of radius R rotates about a frictionless axle. Two masses are suspended from a rope wrapped arround the cylinder. If the system is released from rest, the tension in each rope is?
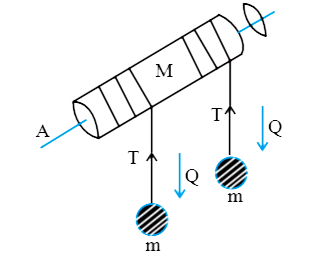 AMmgM+mBMmgM+2mCMmgM+3mDMmgM+4m
AMmgM+mBMmgM+2mCMmgM+3mDMmgM+4mA uniform of rope length L and mass M is placed on a smooth fixed wedge as shown. Both ends of rope are at same horizontal level. The rope is initially released from rest, then the magnitude of initial acceleration of rope is.
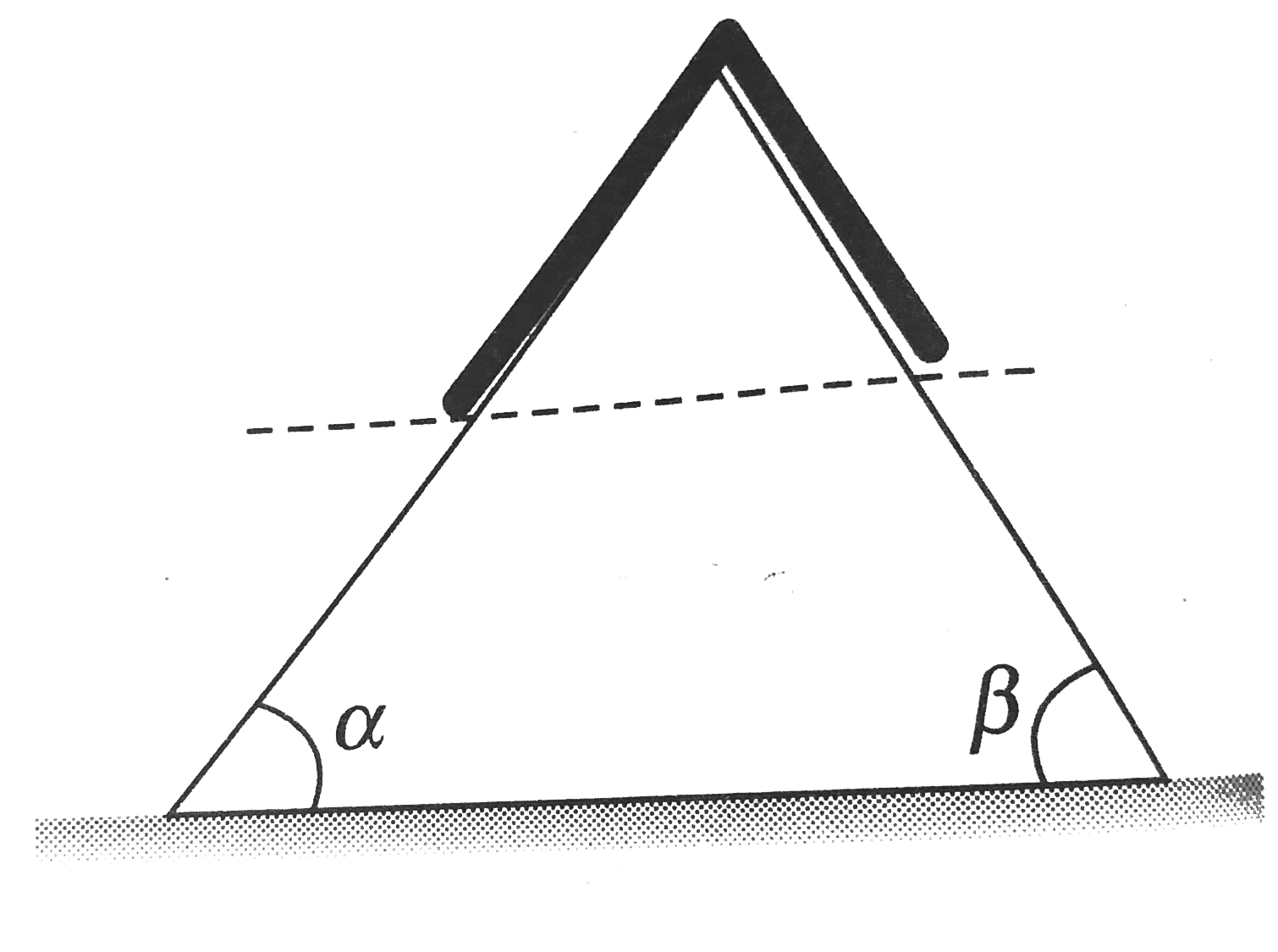 AzeroB(cosα−cosβ)gC(tanα−tanβ)gD(sinα−sinβ)g
AzeroB(cosα−cosβ)gC(tanα−tanβ)gD(sinα−sinβ)g
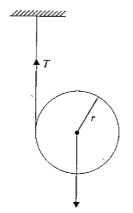
On the surface of a solid cylinder of mass 2 kg and radius 25 cm a string is wound several times and the end of the string is held stationary while the cylinder is released from rest. What is the acceleration of the cylinder and the tension in the string ?
View SolutionA light thread has been tightly wrapped around a disc of mass M and radius R. The disc has been placed on a smooth table, lying flat as shown.
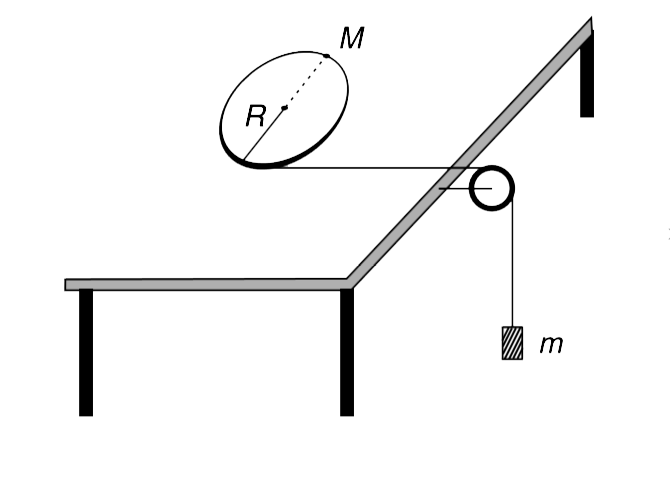
The other end of the string has been attached to a mass m as shown. The system is released from rest. If m=M, which point of the disc will have zero acceleration, immediately after the system is released?
View SolutionA uniform solid cylinder of mass M and of radius R rotates about a frictionless axle. Two masses are suspended from a rope wrapped arround the cylinder. If the system is released from rest, the tension in each rope is?

View Solution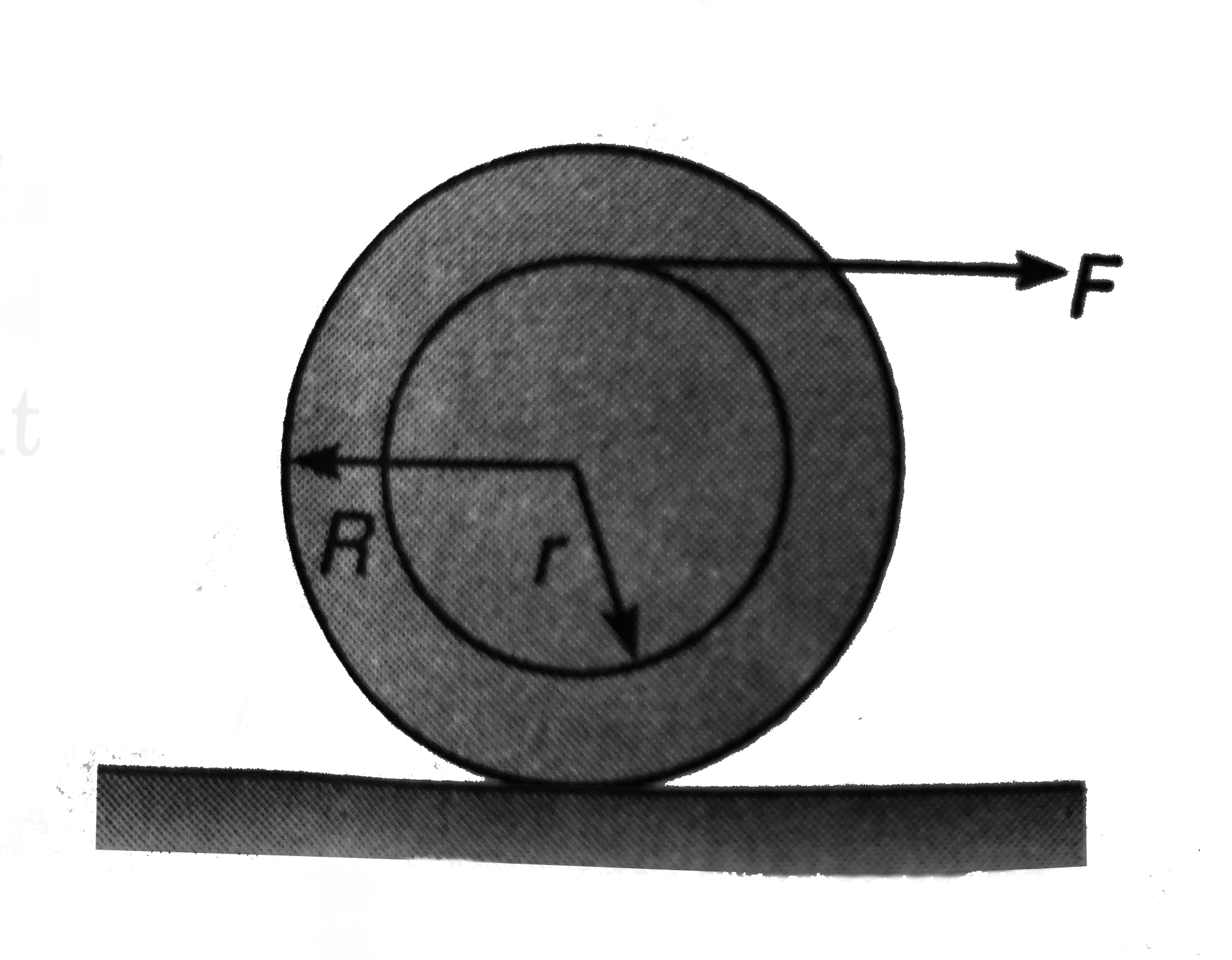
A heavy homogeneous cylinder has mass m and radius R. It is accelerated by a force F which is applied through a rope wound around a light drum of radius r attached to the cylinder (figure) the coefficient of static friction is sufficient for the cylinder to roll without slipping.
View SolutionTwo uniform cylinders, each of mass m=10 kg and radius r = 150 mm, are connected by a rough belt as shown. If the system is released from rest, determine
the tension in the portion of the belt connecting the two cylinders.
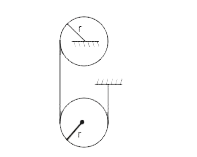
View Solution
