Show-that the trajectory of an object thrown at certain angle with horizontal is a parabola.
Projectile : A body thrown into the air same angle with the horizontal ,(other tan its motion under the influce of gravity is called projectile . The path followed by it is called trajectory .
Let a body is projected from point O , with velocity .u. at an angle with horizontal The velocity .u. can be resolved into two rectangular components along x- axis and y-axis
After time t, Horizontal distance travelled
..........(1)
After a time t. sec , vertical displacement
From
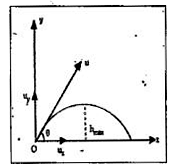
Let then
The above equation represents parabola .Hence the path of a projectile is a parabola.
Topper's Solved these Questions
- MODEL PAPER 3 BOOK - VGS PUBLICATION-BRILLIANTCHAPTER - MODEL PAPER 3 EXERCISE - SECTION-C
3 Videos
- MODEL PAPER 3 BOOK - VGS PUBLICATION-BRILLIANTCHAPTER - MODEL PAPER 3 EXERCISE - SECTION-C
3 Videos
- MODEL PAPER 2BOOK - VGS PUBLICATION-BRILLIANTCHAPTER - MODEL PAPER 2EXERCISE - SECTION C
3 Videos
- MODEL PAPER 4 BOOK - VGS PUBLICATION-BRILLIANTCHAPTER - MODEL PAPER 4 EXERCISE - SECTION -C
3 Videos
Similar Questions
Knowledge Check
The trajectory of an object is determined by
AIts locationBIts velocity at various momentsCBoth (1) and (2)DNone of the aboveThe trajectory of a projectile fired horizontally with velocity u is parabola given by-
Ay=g2u2x2By=−g2u2x2Cy=g2u2y2Dy=g2u2y2The angle of which the velocity vector of a projectile thrown with a velocity u at an angle θ to the horizontal will take with the horizontal after time t of its being thrown up is
AθBtan−1(θt)Ctan−1(vcosθvsinθ−gt)Dtan−1(vsinθ−gtvcosθ)
Show that trajectory of a projected at angle 'θ' with horizontal is parabolic in nature.
View SolutionA point P on the trajectory of a projectile projected at angle with θ with horizontal subtrends angles α and β at the point of landing them
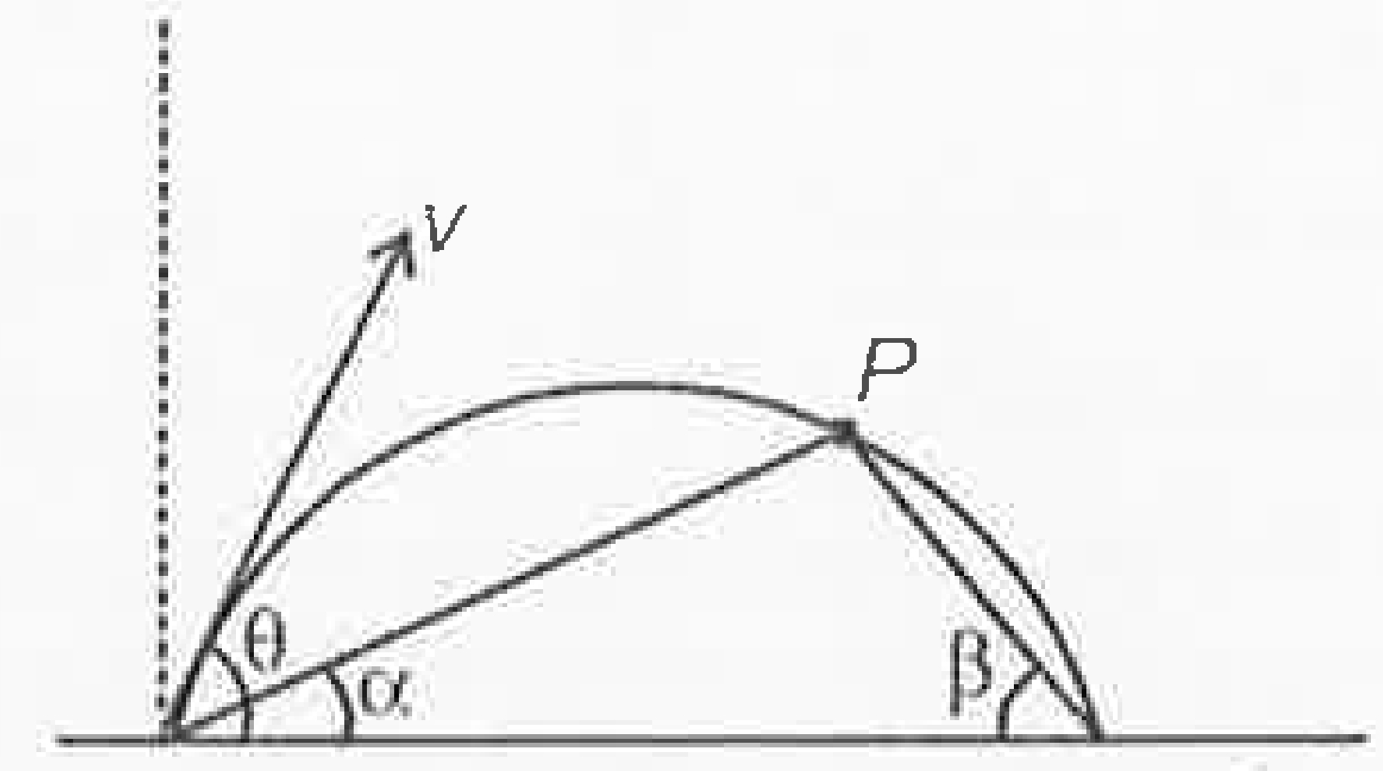
View SolutionWhat is the angle made by the trajectory of a projectile with horizontal at maximum height ?
View SolutionThe angle which the velocity vector of a projectile thrown with a velocity v at an. angle θ to the horizontal Will make with the horizontal after time t of its being thrown up is
View SolutionThe angle of elevation of an object on a hill is observed from a certain point in the horizontal plane through its base, to be 30∘. After walking 120 m towards it on a level groud, the angle of elevation is found to be 60∘. Then the height of the object (in metres) is
View Solution
