Slit 1 of a double slit is wider than slit 2, so that the light from slit 1 has an amplitudes three times that of the light from slit 2. Show that equation I=Imaxcos2ϕ2 is replaced by the equation,
I=(Imax4(1+3cos2(ϕ2)) .
To solve the problem, we need to derive the new intensity equation for a double-slit interference pattern when the amplitudes of the light from the two slits are different.
1. Define Amplitudes:
Let the amplitude of light from slit 2 be A2=a0. Since the amplitude from slit 1 is three times that of slit 2, we have:
A1=3A2=3a0
2. Calculate Maximum Amplitude:
The maximum amplitude Amax when both slits are open is the sum of the individual amplitudes:
Amax=A1+A2=3a0+a0=4a0
3. Relate Intensity to Amplitude:
The intensity I is proportional to the square of the amplitude:
I∝A2
Therefore, the maximum intensity Imax can be expressed as:
Imax∝(Amax)2=(4a0)2=16a20
4. Calculate Individual Intensities:
The intensities from each slit can be expressed as:
I1∝A21=(3a0)2=9a20
I2∝A22=(a0)2=a20
5. Use the Formula for Net Intensity:
The net intensity I when both slits are open is given by:
I=I1+I2+2√I1I2cosϕ
Substituting the values:
I=9a20+a20+2√9a20⋅a20cosϕ
I=10a20+6a20cosϕ
6. Substitute for Maximum Intensity:
Since Imax is proportional to 16a20, we can express a20 in terms of Imax:
a20=Imax16
Substituting this into the intensity equation:
I=10(Imax16)+6(Imax16)cosϕ
I=10Imax16+6Imax16cosϕ
I=Imax16(10+6cosϕ)
7. Factor Out the Common Terms:
Rewrite the equation:
I=Imax16(10+6cosϕ)
8. Express in Terms of Cosine Squared:
We can express 10+6cosϕ in terms of cos2(ϕ/2):
10+6cosϕ=5+3(2cos2(ϕ/2)−1)=2+3⋅2cos2(ϕ/2)=2+6cos2(ϕ/2)
Thus, we can write:
I=Imax4(1+3cos2(ϕ/2))
Final Result:
The final expression for the intensity is:
I=Imax4(1+3cos2(ϕ/2))
Let the amplitude of light from slit 2 be A2=a0. Since the amplitude from slit 1 is three times that of slit 2, we have:
A1=3A2=3a0
The maximum amplitude Amax when both slits are open is the sum of the individual amplitudes:
Amax=A1+A2=3a0+a0=4a0
The intensity I is proportional to the square of the amplitude:
I∝A2
Therefore, the maximum intensity Imax can be expressed as:
Imax∝(Amax)2=(4a0)2=16a20
The intensities from each slit can be expressed as:
I1∝A21=(3a0)2=9a20
I2∝A22=(a0)2=a20
The net intensity I when both slits are open is given by:
I=I1+I2+2√I1I2cosϕ
Substituting the values:
I=9a20+a20+2√9a20⋅a20cosϕ
I=10a20+6a20cosϕ
Since Imax is proportional to 16a20, we can express a20 in terms of Imax:
a20=Imax16
Substituting this into the intensity equation:
I=10(Imax16)+6(Imax16)cosϕ
I=10Imax16+6Imax16cosϕ
I=Imax16(10+6cosϕ)
Rewrite the equation:
I=Imax16(10+6cosϕ)
We can express 10+6cosϕ in terms of cos2(ϕ/2):
10+6cosϕ=5+3(2cos2(ϕ/2)−1)=2+3⋅2cos2(ϕ/2)=2+6cos2(ϕ/2)
Thus, we can write:
I=Imax4(1+3cos2(ϕ/2))
The final expression for the intensity is:
I=Imax4(1+3cos2(ϕ/2))
Topper's Solved these Questions
- INTERFERENCE AND DIFFRACTION OF LIGHTBOOK - DC PANDEY ENGLISHCHAPTER - INTERFERENCE AND DIFFRACTION OF LIGHTEXERCISE - Level 1 Assertion And Reason
10 Videos
- INTERFERENCE AND DIFFRACTION OF LIGHTBOOK - DC PANDEY ENGLISHCHAPTER - INTERFERENCE AND DIFFRACTION OF LIGHTEXERCISE - Level 1 Objective
11 Videos
- INTERFERENCE AND DIFFRACTION OF LIGHTBOOK - DC PANDEY ENGLISHCHAPTER - INTERFERENCE AND DIFFRACTION OF LIGHTEXERCISE - Exercise 32.1
3 Videos
- GRAVITATIONBOOK - DC PANDEY ENGLISHCHAPTER - GRAVITATIONEXERCISE - All Questions
135 Videos
- MAGNETIC FIELD AND FORCESBOOK - DC PANDEY ENGLISHCHAPTER - MAGNETIC FIELD AND FORCESEXERCISE - Medical entrance s gallery
59 Videos
Similar Questions
Slit 1 of Young's double-slit experiment is wider then slit 2, so that the light from slits are given as A1=A0sinωtandA2=3A0sin(ω+π3),, The resultant amplitude and intensity, at a point where the path difference between them is zero, are A and I, respectively. Then
View SolutionTwo transparent slabs having equal thickness but different refractive indices μ1andμ2, are pasted side by side to form a composite slab. This slab is placed just after the double slit in a Young's experiment so that the light from one slit goes through one material and the light from the other slit goes through the other material. What should be the minimum thickness of the slab so that there is a minimum at the point P0 which is equidistant from the slits ?
View Solution
Knowledge Check
Two slits in Young's double slit experiment have widths in the ratio 81:1. What is the the ratio of amplitudes of light waves coming from them ?
A3:1B3:2C9:1D6:1In Young's double slit experiment, one of the slit is wider than other, so that amplitude of the light from one slit is double of that from other slit. If Im be the maximum intensity, the resultant intensity I when they interfere at phase difference ϕ is given by:
AIm3(1+2cos2ϕ2)BIm5(1+4cos2ϕ2)CIm9(1+8cos2ϕ2)DIm9(8+cos2ϕ2)
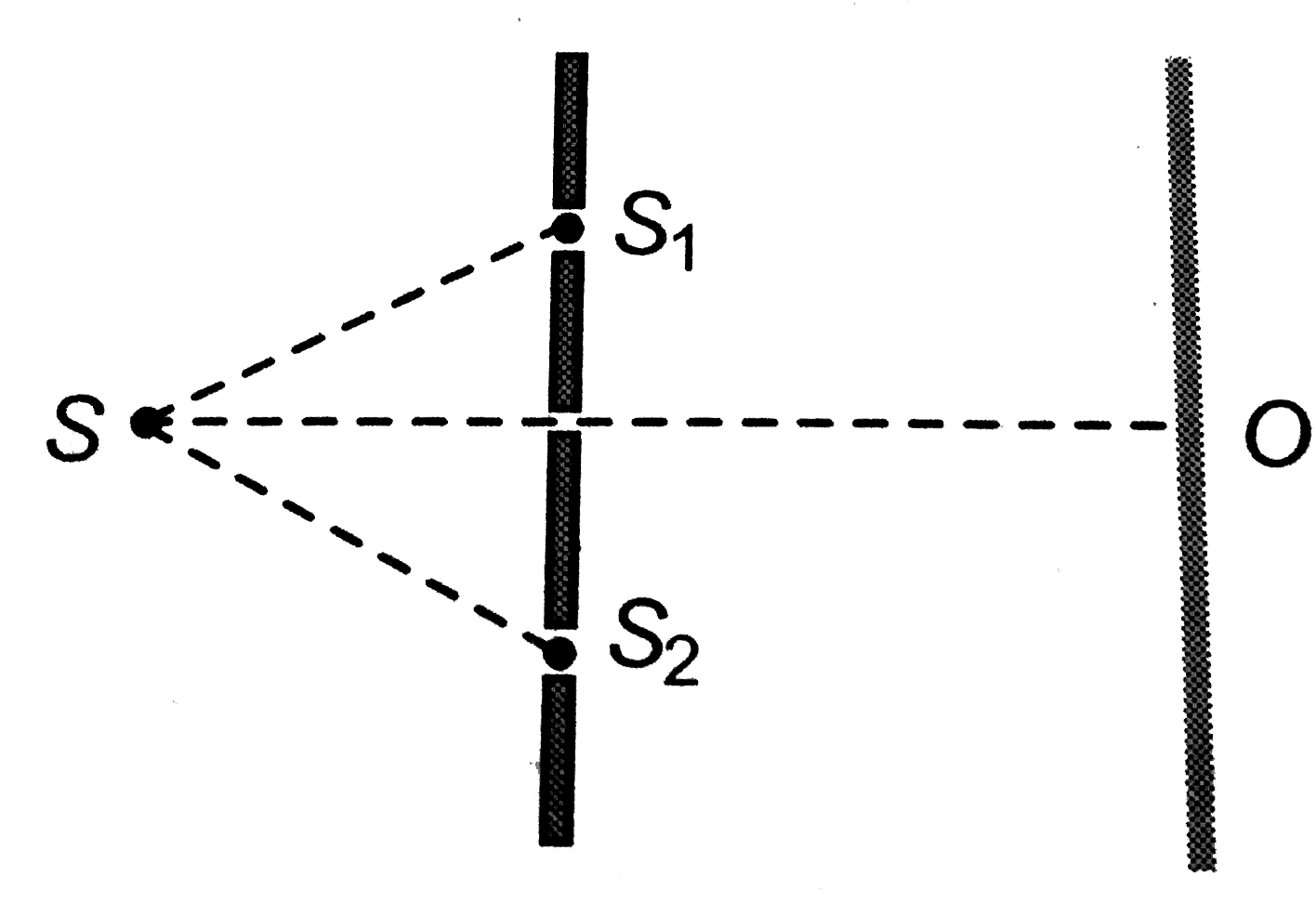
In the set up shown in figure, the two slits S1 and S2 are not equidistant from the slit S. The central fringe at O is then
View SolutionLight of wavelength 5000 Å is incident on a slit of width 0.1 mm. Find out the width of the central bright line on a screen distance 2m from the slit?
View SolutionIn Young's double slit experiment, one of the slit is wider than other, so that amplitude of the light from one slit is double of that from other slit. If Im be the maximum intensity, the resultant intensity I when they interfere at phase difference ϕ is given by:
View SolutionIn a Young's double slit experiment, the separation between the two slits is d and the wavelength of the light is λ. The intensity of light falling on slit 1 is four times the intensity of light falling on slit 2. Choose the correct choice (s).
View SolutionIn a Young's double slit experiment, the slit separation is 1mm and the screen is 1m from the slit. For a monochromatic light of wavelength 500nm, the distance of 3rd minima from the central maxima is
View SolutionIn double slit experiment what should be the width of each slit to obtain 10 maxima of the double slit pattern within the central maxima of single slit pattern with d=2 mm.
View Solution
