State Gauss's theorem. Obtain an expression for elactric field at any point outside a charged spherical hollow conductor (shell).Solution in Kannada
Gauss.s theorem: "The total outward electric flux passing through a closed surface in air is (1ε0) times the total charge enclosed by it..
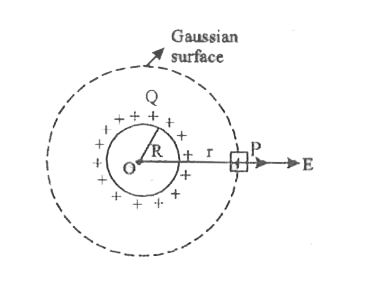
Let +Q. be the charge enclosed by a hollow conductor of radius .R..
Let .p. be a point at a distance .r. from the centre of the conductor Let .ds be a small element of area surrounding the point. A normal drawn from the point coincides with the direction of E. Hence cos 0∘=1.
From Gauss. theorem,
TOEF=ϕ=(1ε0)Q ...(1)
by definition
ϕ=Ecosθ∑ds.
where ∑ds=4πr2,cosθ=cos0∘=1
Hence , ϕ=E.4πr2 ...(2)
Comparing (1) and (2)
we write E=(14πε0)Qr2
For a point on the surface , r =R .
E=(14πε0)(QR2) .
This electric field intensity is maximum. Since electric flux depends on the charge enclosed, and electric field intensity depends on the electric flux, electric field remains zero at all points inside the spherical hollow conductor.
Topper's Solved these Questions
Similar Questions
A spherical hollow conductor is charged to -250V. If the radius of the conductor is 0.15m, then calculate
(i) Charge on the conductor.
(ii) Electric files intensity on the surface
(iii) Electric field intensity at the centre.
(iv) Electric potential difference between A and B at distances of 0.20 m & 0.25m from the centre respectively.
View SolutionFor a short magnetic dipole of magnetic moment 0.5 Am2. Find the magnetic field at a point ( a) 1 m on the axis and from the cetre of the magnet ( dipole ) ( b) 1 m on the equatorial line from the centre ( c) at an angle of 60∘ w.r. to the line joining the point and the centre of the dipole at distance 1 m.
View SolutionState Gauss's theorem in electrostatiics. State the expression for electric field intensity at a point outside an infinitely long charged conducting cylinder.
View SolutionState Gauss's theorem. Obtain an expression for elactric field at any point outside a charged spherical hollow conductor (shell).
View SolutionUsing Gauss's law in electrostatics, obtain an expression for electric field due to a uniformly charged thin spherical shell at a point (i) Outside the shell and (ii) Inside the shell
View SolutionUsing Gauss's law in electrostatics, obtain an expression for electric field due to a uniformly charged thin spherical shell at a point
(i) Outside the shell and
(ii) Inside the shell
View SolutionState Gauss's theorem. Obtain an expression for elactric field at any point outside a charged spherical hollow conductor (shell).
View SolutionUsing Gauss's theorem derive an expression for the electric field at any point outside a charged spherical shell of radius R and of charge density sigma C//m^(2)
View SolutionUsing Gauss's theorem show mathematically that for any point outside the shell , the field due to a uniformaly charged thin spherical shell is the same as if the entire charge of the shell is concentrated at the centre? Why do you expect the electric field inside the shell to be zero according to this theorem?
View SolutionObtain the expression of electric field by thir spherical shell with uniform charge distribution at a point outside it.
View SolutionState Gauss's theorem in electrostatics. Apply this theorem to derive an expression for electric field intensity at a point outside a uniformly charged thin spherical shell.
View SolutionState Gauss's theorem with the help of diagram, derive an expression for the electric field intensity due to uniformly charged thin spherical shell at a point outside
View SolutionState Gauss's theorem with the help of diagram, derive an expression for the electric field intensity due to uniformly charged thin spherical shell at a point inside
View SolutionState Gauss's theorem with the help of diagram, derive an expression for the electric field intensity due to uniformly charged thin spherical shell at a point on the surface of the spherical shell.
View SolutionApply Gauss's theorem to show that for a charged spherical shell, the electric field outside it is as if all the charge is concentrated at the centre.
View SolutionUsing Gauss's theorem find the filed due to a charged thin spherical shell at a point outside the shell.
View Solution
