Suppose the block of the previous problem is pushed down the incine witas force of 4N. How far will the block move in the first two seconds after starting from rest? The mass of the block is 4 kg.
To solve the problem step by step, we will follow these instructions:
Step 1: Determine the acceleration of the block.
The block is subjected to a downward force of 4 N in addition to the gravitational force acting on it. The net force acting on the block can be expressed as:
Fnet=Fapplied+Fgravity−Ffriction
Where:
- Fapplied=4N
- Fgravity=mgsin(θ)
- Ffriction=μkmgcos(θ)
Given:
- Mass m=4kg
- Gravitational acceleration g=9.8m/s2
- Angle of inclination θ=30∘
Calculating the gravitational forces:
- Fgravity=4×9.8×sin(30∘)=4×9.8×0.5=19.6N
- Ffriction=μk×4×9.8×cos(30∘)=μk×4×9.8×√32
The net force can then be expressed as:
Fnet=4+19.6−μk×4×9.8×√32
Step 2: Calculate the acceleration.
Using Newton's second law, F=ma:
a=Fnetm
Substituting the values:
a=4+19.6−μk×4×9.8×√324
Step 3: Calculate the coefficient of kinetic friction μk.
From the previous problem, we found that the block covered 8 m in the first 2 seconds with an acceleration of 4 m/s². Thus, we can find μk by rearranging the acceleration equation from the previous problem.
Step 4: Substitute μk back into the acceleration equation.
Once we have μk, we can substitute it back into the equation for acceleration to find the new acceleration when the block is pushed down with the 4 N force.
Step 5: Calculate the distance moved in the first 2 seconds.
Using the formula for displacement under constant acceleration:
s=ut+12at2
Where:
- u=0 (initial velocity, starting from rest)
- t=2s
Substituting the values:
s=0+12a(2)2=2a
Final Calculation
After calculating a from the previous steps, we can compute s.
Solution Summary
The distance moved by the block in the first 2 seconds after starting from rest, when pushed down with a force of 4 N, is found to be 10 meters.
---
The block is subjected to a downward force of 4 N in addition to the gravitational force acting on it. The net force acting on the block can be expressed as:
- Fapplied=4N
- Fgravity=mgsin(θ)
- Ffriction=μkmgcos(θ)
- Mass m=4kg
- Gravitational acceleration g=9.8m/s2
- Angle of inclination θ=30∘
- Fgravity=4×9.8×sin(30∘)=4×9.8×0.5=19.6N
- Ffriction=μk×4×9.8×cos(30∘)=μk×4×9.8×√32
Fnet=4+19.6−μk×4×9.8×√32
Using Newton's second law, F=ma:
a=Fnetm
a=4+19.6−μk×4×9.8×√324
From the previous problem, we found that the block covered 8 m in the first 2 seconds with an acceleration of 4 m/s². Thus, we can find μk by rearranging the acceleration equation from the previous problem.
Once we have μk, we can substitute it back into the equation for acceleration to find the new acceleration when the block is pushed down with the 4 N force.
Using the formula for displacement under constant acceleration:
s=ut+12at2
Where:
- u=0 (initial velocity, starting from rest)
- t=2s
s=0+12a(2)2=2a
After calculating a from the previous steps, we can compute s.
The distance moved by the block in the first 2 seconds after starting from rest, when pushed down with a force of 4 N, is found to be 10 meters.
Topper's Solved these Questions
- FRICTIONBOOK - HC VERMA ENGLISHCHAPTER - FRICTIONEXERCISE - Questions for short Answer
11 Videos
- FRICTIONBOOK - HC VERMA ENGLISHCHAPTER - FRICTIONEXERCISE - Objective -2
5 Videos
- FLUID MECHANICSBOOK - HC VERMA ENGLISHCHAPTER - FLUID MECHANICSEXERCISE - All Questions
90 Videos
- GRAVITATIONBOOK - HC VERMA ENGLISHCHAPTER - GRAVITATIONEXERCISE - Question for short Answers
18 Videos
Similar Questions
A block of mass 2.0 kg is pushed down an inclined plane of inclination 370 with a force of 20N acting parallel to the incline. It is found that the block moves on the incline with an acceleration of 10ms2. If the block started from rest, find the work done a. by the applied force in the first second b. by the weight of the block in the first second and c. by the frictional force acting on the block in the first second. Take g=10ms2.
View SolutionA block of mass 2.0 kg is pushed down an inclined plane of inclination 370 with a force of 20N cting parallelto the incline. It is found tht the block moves on the incline with an acceleration of 10ms2. If the blcok started from rest, find the work done a. by the applied force in the first second b. by the weight of the block in the first second and c. by the frictional force acting on the block in the first second. Take g=10ms2.
View SolutionA block of mass 2kg is pushed down an inclined plane of angle 37∘ above horizontal with a force parallel to inclined plane. The coefficient of friction is 3/4. The block moves 10m in 2 seconds starting form rest. The magnitude of the force is
View SolutionA man wants to slide down a block of mass m which is kept on a fixed inclined plane of inclination 30∘ as shown in the figure .Initially the block is not sliding .
To just start sliding the man pushed the block down the inclined with a force F. Now , the block starts accelerating. To move it downwards with conatant speed the man starts pulling the block with same force. Surface are such that ratio of maximum static friction to kinetic friction is 2 Now, answer the following questions.
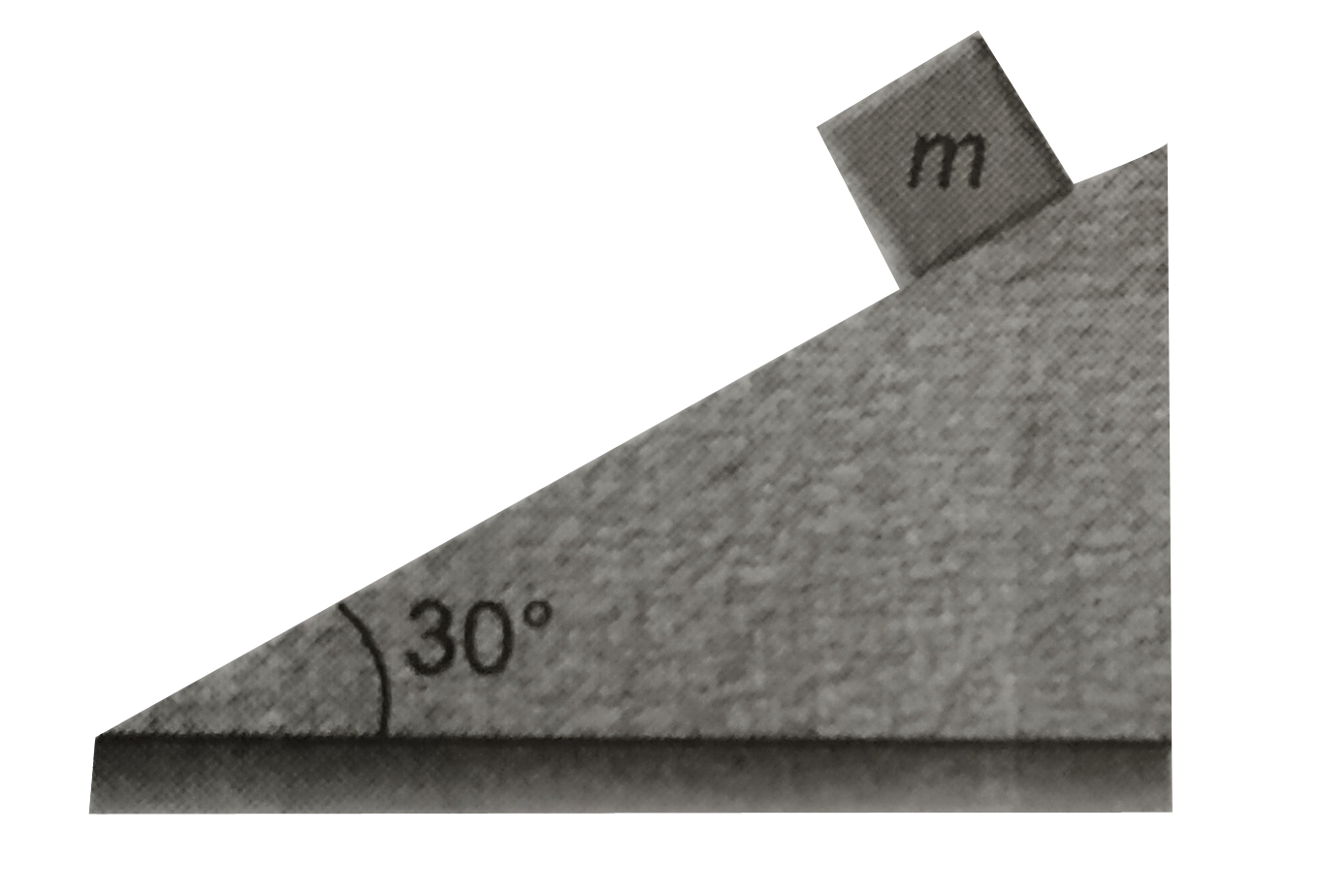
If the man continues pushing the block by force F its acceleration would be
View SolutionA man wants to slide down a block of mass m which is kept on a fixed inclined plane of inclination 30∘ as shown in the figure .Initially the block is not sliding .
To just start sliding the man pushed the block down the inclined with a force F. Now , the block starts accelerating. To move it downwards with conatant speed the man starts pulling the block with same force. Surface are such that ratio of maximum static friction to kinetic friction is 2 Now, answer the following questions.
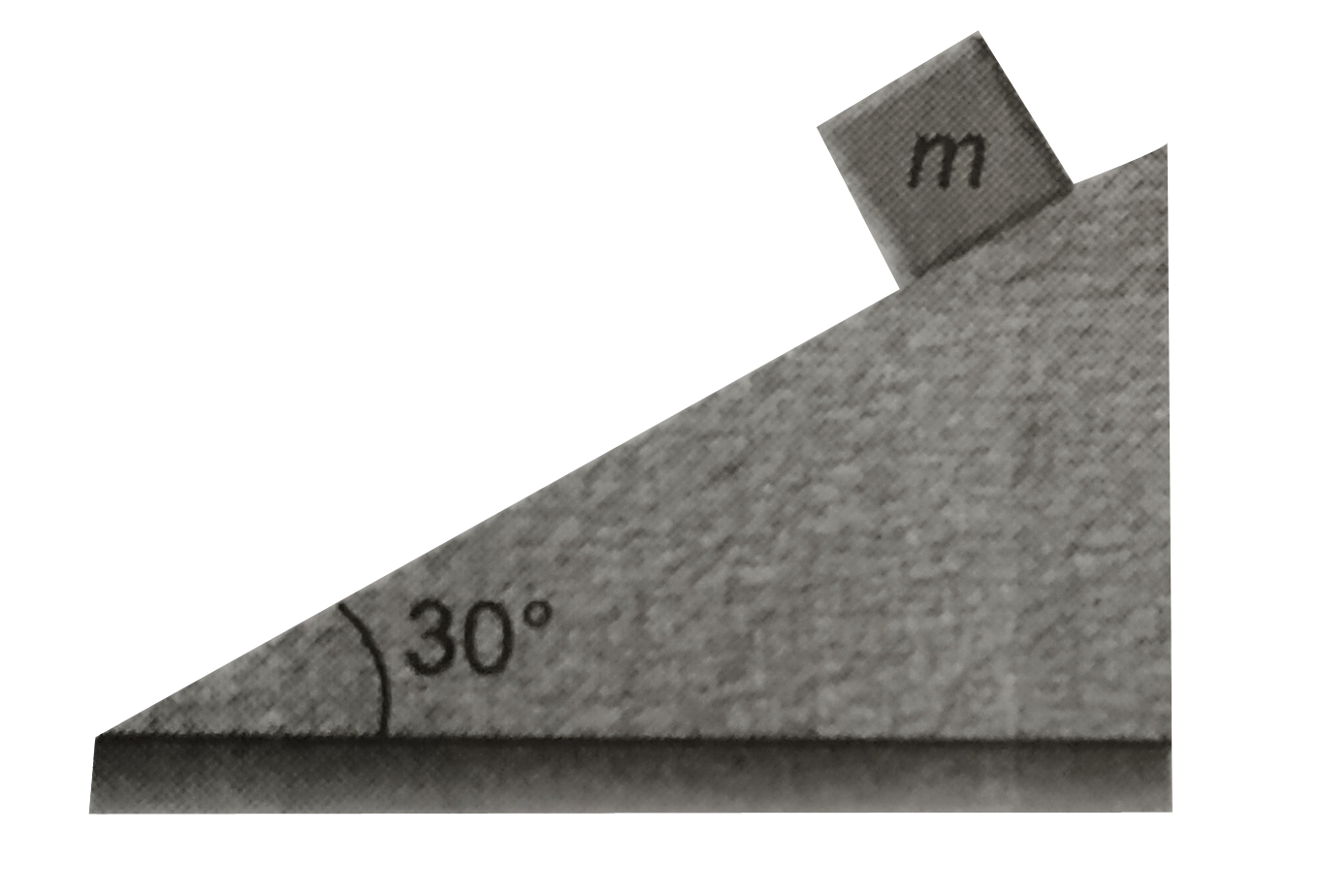
What minimum force is required to move it up the incline with constant speed?
View SolutionA man wants to slide down a block of mass m which is kept on a fixed inclined plane of inclination 30∘ as shown in the figure .Initially the block is not sliding .
To just start sliding the man pushed the block down the inclined with a force F. Now , the block starts accelerating. To move it downwards with conatant speed the man starts pulling the block with same force. Surface are such that ratio of maximum static friction to kinetic friction is 2 Now, answer the following questions.
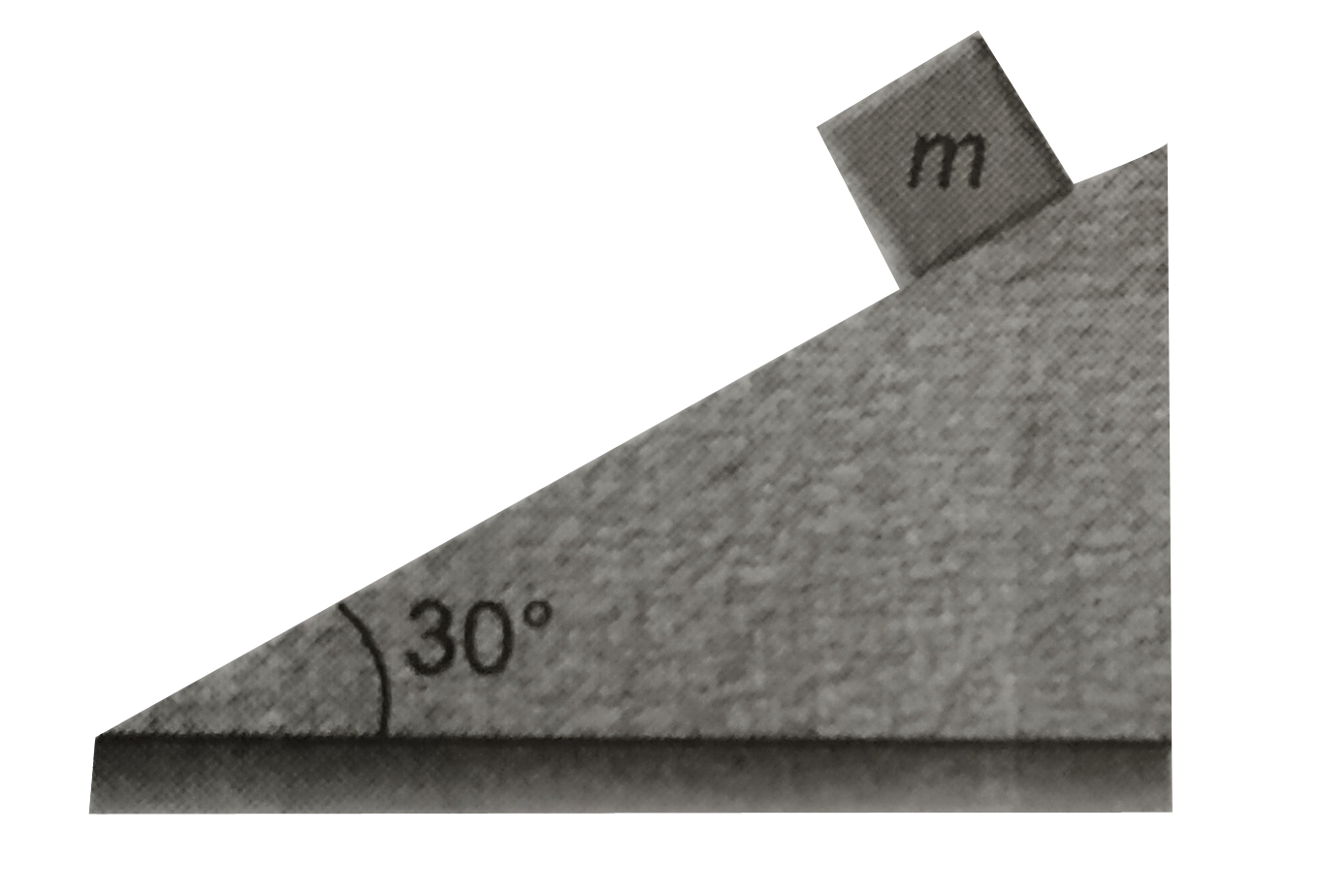
What is the value of F?
View SolutionA man wants to slide down a block of mass m which is kept on a fixed inclined plane of inclination 30∘ as shown in the figure .Initially the block is not sliding .
To just start sliding the man pushed the block down the inclined with a force F. Now , the block starts accelerating. To move it downwards with conatant speed the man starts pulling the block with same force. Surface are such that ratio of maximum static friction to kinetic friction is 2 Now, answer the following questions.
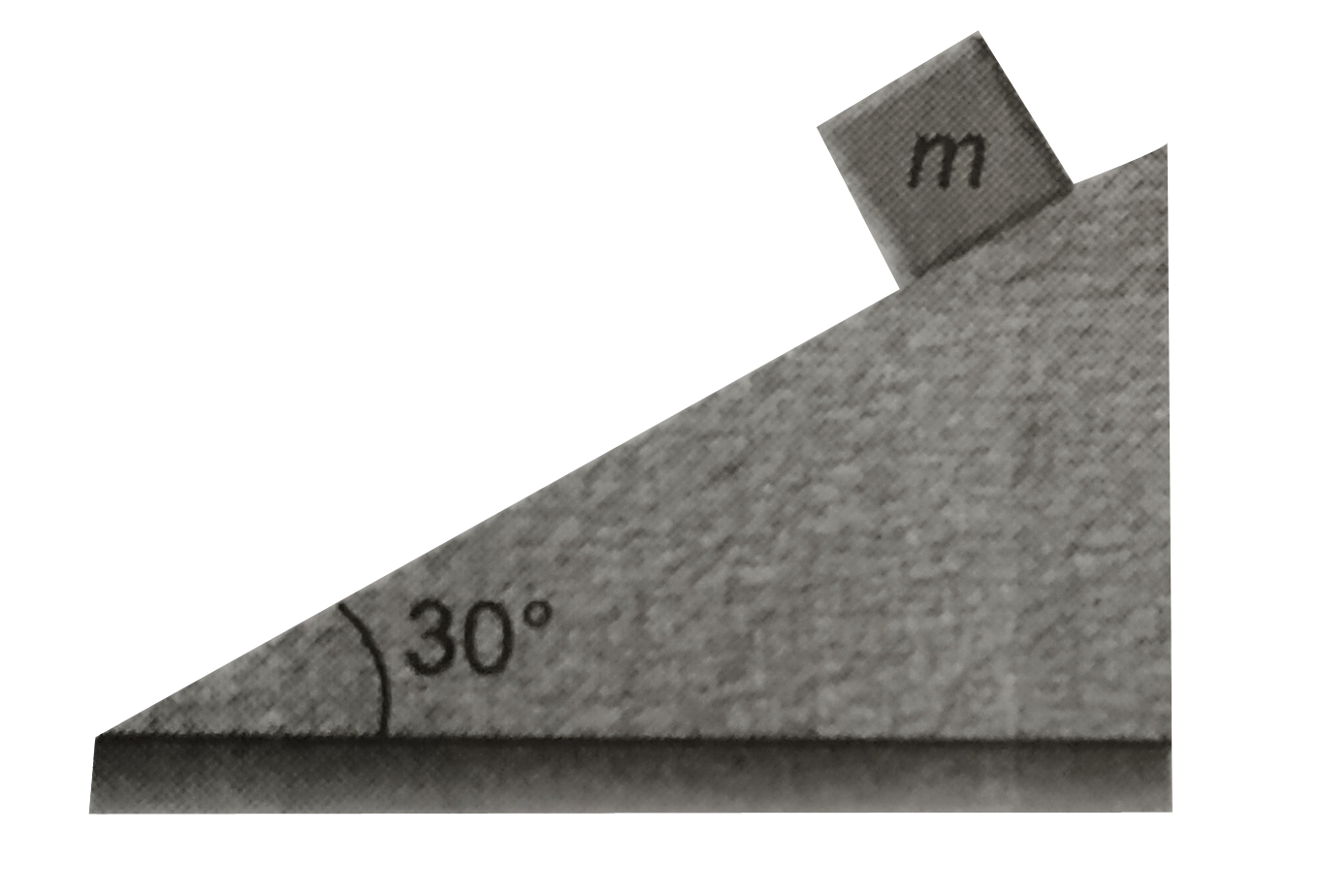
What is the value of μs, the coefficient of static friction?
View SolutionA man wants to slide down a block of mass m which is kept on a fixed inclined plane of inclination 30∘ as shown in the figure .Initially the block is not sliding .
To just start sliding the man pushed the block down the inclined with a force F. Now , the block starts accelerating. To move it downwards with conatant speed the man starts pulling the block with same force. Surface are such that ratio of maximum static friction to kinetic friction is 2 Now, answer the following questions.
If the man wants to move the block up the inclined , what minimum force is required to start the motion?
View Solution
A man wants to slide down a block of mass m which is kept on a fixed inclined plane of inclination 30∘ as shown in figure initially, the block is not sliding. To just start sliding the man pushes the block down the inclined with a force F. Now the block starts accelerating. To move it downwards with constant speed, the man starts pulling the block with same force. Surfaces are such that ratio of maximum static friction to kinetic friction is 2. Now, answer the following questions.
If man continues pushing the block by force F, its acceleration would be
View SolutionA block of mass 2 kg is pushed against a rough vertical wall with a force is 40 N. Coefficient of static friction being 0.5. Another horizontal force is 15 N, is applied on the block in a direction parallel to the wall. Will the block move? If yes in which direction? If no, find the frictional force exerted by the wall on the block.
View Solution
