The angle made by the string of a simple pendulum with the vertical depends on time as θ=π90sin[(πs−1)t]. Find the length of the pendulum if g=π2ms−2
The correct Answer is:lenght of the pendulum is 1m.
To solve the problem, we need to find the length of the pendulum given the angle as a function of time and the acceleration due to gravity. Let's break it down step by step.
Step 1: Identify the given information
We are given the angle θ as:
θ=π90sin(πt−1)
and the value of g as:
g=π2m/s2
Step 2: Determine the angular frequency
From the equation of motion, we can identify the angular frequency ω from the sine function. The general form of simple harmonic motion is:
θ(t)=θ0sin(ωt)
Comparing this with our equation, we find:
ω=π
Step 3: Calculate the time period
The time period T of a simple pendulum is given by:
T=2πω
Substituting the value of ω:
T=2ππ=2seconds
Step 4: Relate the time period to the length of the pendulum
The time period T of a simple pendulum is also related to its length l and the acceleration due to gravity g by the formula:
T=2π√lg
Substituting the known value of T:
2=2π√lg
Step 5: Simplify the equation
Dividing both sides by 2:
1=π√lg
Now, squaring both sides:
1=π2lg
Step 6: Solve for the length l
Rearranging the equation gives:
l=gπ2
Substituting the value of g:
l=π2π2=1meter
Final Answer
The length of the pendulum is:
l=1meter
We are given the angle θ as:
θ=π90sin(πt−1)
and the value of g as:
g=π2m/s2
From the equation of motion, we can identify the angular frequency ω from the sine function. The general form of simple harmonic motion is:
θ(t)=θ0sin(ωt)
Comparing this with our equation, we find:
ω=π
The time period T of a simple pendulum is given by:
T=2πω
Substituting the value of ω:
T=2ππ=2seconds
The time period T of a simple pendulum is also related to its length l and the acceleration due to gravity g by the formula:
T=2π√lg
Substituting the known value of T:
2=2π√lg
Dividing both sides by 2:
1=π√lg
Now, squaring both sides:
1=π2lg
Rearranging the equation gives:
l=gπ2
Substituting the value of g:
l=π2π2=1meter
The length of the pendulum is:
l=1meter
Topper's Solved these Questions
- SIMPLE HARMONIC MOTIONBook:HC VERMAChapter:SIMPLE HARMONIC MOTIONExercise:Objective-2
Explore 2 Videos
- ROTATIONAL MECHANICSBook:HC VERMAChapter:ROTATIONAL MECHANICSExercise:Exercises
Explore 86 Videos
- SOME MECHANICAL PROPERTIES OF MATTERBook:HC VERMAChapter:SOME MECHANICAL PROPERTIES OF MATTERExercise:Exercises
Explore 32 Videos
Similar Questions
Knowledge Check
Time period of a simple pendulum only depends on the _______________ of the string.
AOscillationBfrequencyClengthDamplitudeAssertion : If the length of the simple pendulum is equal to the radius of the earth, the period of the pendulum is T=2π√R/g
Reason : Length of this pendulum is equal to radius of earth.Aboth, Assertion and Reason are true and Reason is the correct explanation of the Assertion.Bboth, Assertion and Reason are true but Reason is not a correct explanation of the Assertion.CAssertion is flase but the Reason is true.Dboth,Assertion and Reason are false.While measuring the acceleration due to gravity by a simple pendulum , a student makes a positive error of 1% in the length of the pendulum and a negative error of 3% in the value of time period . His percentage error in the measurement of g by the relation g=4π2(l/T2) will be
A2%B4%C7%D10%
Find the length of seconds pendulum at a place where g=π2m/s2.
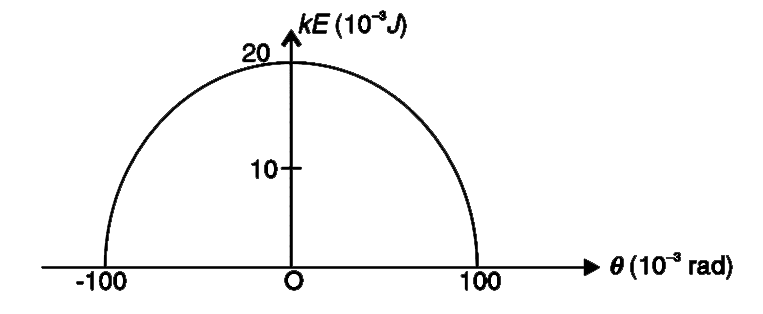
View SolutionThe given figure shows the variation of the kinetic energy of a simple pendulum with its angular displacement (θ) from the vertical. Mass of the pendulum bob is m = 0.2 kg. Find the time period of the pendulum. Take g=10ms−2.
View SolutionShow that the angle made by the string with the vertical in a conical pendulum is given by cosθ=gLω2 , where L is the string and ω is the angular speed.
View SolutionCalculate the tiem period of a simple pendulum of length one meter. The acceleration due ot gravity at the place is π2ms−2.
View SolutionThe time period of a simple pendulum is 1.2 s. If the length of the pendulum is doubled, the new time period will be
View Solution
