The capacitance of a parallel plate capacitor is 12μF. If the distance between the plates is doubled and area is halved, then new capacitance will be
8μF
6μF
4μF
3μF
The correct Answer is:D
To find the new capacitance of a parallel plate capacitor when the distance between the plates is doubled and the area is halved, we can follow these steps:
Step 1: Understand the formula for capacitance
The capacitance C of a parallel plate capacitor is given by the formula:
C=Aϵ0d
where:
- C is the capacitance,
- A is the area of the plates,
- ϵ0 is the permittivity of free space,
- d is the distance between the plates.
Step 2: Identify the initial values
From the problem, we know:
- Initial capacitance C=12μF
- Let the initial area be A and the initial distance be d.
Step 3: Determine the new values
According to the problem:
- The new distance d′=2d (the distance is doubled).
- The new area A′=A2 (the area is halved).
Step 4: Substitute the new values into the capacitance formula
Now, we can calculate the new capacitance C′:
C′=A′ϵ0d′
Substituting the new values:
C′=(A2)ϵ02d
Step 5: Simplify the expression
Now simplifying the expression:
C′=Aϵ02⋅2d=Aϵ04d
This shows that:
C′=C4
where C is the original capacitance.
Step 6: Calculate the new capacitance
Since the original capacitance C=12μF:
C′=12μF4=3μF
Final Answer
The new capacitance C′ is 3μF.
---
The capacitance C of a parallel plate capacitor is given by the formula:
C=Aϵ0d
where:
- C is the capacitance,
- A is the area of the plates,
- ϵ0 is the permittivity of free space,
- d is the distance between the plates.
From the problem, we know:
- Initial capacitance C=12μF
- Let the initial area be A and the initial distance be d.
According to the problem:
- The new distance d′=2d (the distance is doubled).
- The new area A′=A2 (the area is halved).
Now, we can calculate the new capacitance C′:
C′=A′ϵ0d′
Substituting the new values:
C′=(A2)ϵ02d
Now simplifying the expression:
C′=Aϵ02⋅2d=Aϵ04d
This shows that:
C′=C4
where C is the original capacitance.
Since the original capacitance C=12μF:
C′=12μF4=3μF
The new capacitance C′ is 3μF.
Topper's Solved these Questions
- ELECTROSTATIC POTENTIAL AND CAPACITORSBOOK - DC PANDEY ENGLISHCHAPTER - ELECTROSTATIC POTENTIAL AND CAPACITORSEXERCISE - Check point 2.5
20 Videos
- ELECTROSTATIC POTENTIAL AND CAPACITORSBOOK - DC PANDEY ENGLISHCHAPTER - ELECTROSTATIC POTENTIAL AND CAPACITORSEXERCISE - (A) Chapter exercises
227 Videos
- ELECTROSTATIC POTENTIAL AND CAPACITORSBOOK - DC PANDEY ENGLISHCHAPTER - ELECTROSTATIC POTENTIAL AND CAPACITORSEXERCISE - Check point 2.3
15 Videos
- ELECTROMAGNETIC WAVESBOOK - DC PANDEY ENGLISHCHAPTER - ELECTROMAGNETIC WAVESEXERCISE - Sec C
22 Videos
- ELECTROSTATICSBOOK - DC PANDEY ENGLISHCHAPTER - ELECTROSTATICSEXERCISE - Medical entrances gallery
37 Videos
Similar Questions
To reduce the capacitance of parallel plate capacitor, the space between the plate is
View SolutionA parallel plate capacitor with air between the plates has a capacitance C. If the distance between the plates is doubled and the space between the plates is filled with a dielectric of dielectric constant 6, then the capacitance will become.
View Solution
Knowledge Check
The capacitance of a parallel plate capacitor with air as medium is 3μF. with the introduction of a dielectric medium between the plates, the capacitance becomes 15μF. The permittivity of the medium is
A5C2N−1M−2B15C2N−1m−2C0.44×10−10C2N−1m−2D8.854×10−11C2N−1m−2As the distance between the plates of a parallel plate capacitor decreased
AIf both assertion and reason are ture and reason is the correct explanation of assertion.BIf both assertin and reason are ture but reason is not the correct explanation of assertion .CIf assertion is true but reason is false.DIf both assertion and reason are false.
The capacitance of a parallel plate capacitor is 2μF and the charge on its positive plate is 2μC. If the charge on its plates is doubled, the capacitance of the capacitor
View SolutionThe' distance between the plates of a parallel plate capacitor is d. A metal plate of thickness d/2 is placed between the plates. What will e its effect on the capacitance.
View SolutionDerive an expression for the capacitance of a parallel plate capacitor in terms of the areas of the plates and the distance between them. Assume there is a vacuum in between the plates with the electric field between the plates E=σε0 (where the symbols have their usual meaning).
View SolutionCalculate the capacitance of the parallel plate capacitor shown in the following figure between point X and Y.
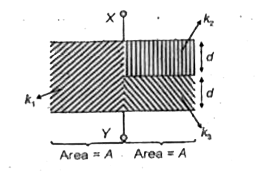
View SolutionFind out the capacitance of parallel plate capacitor of plate area A and plate separation d.
View SolutionThe plates of a parallel plate capacitance 1.0 F are separated by a distance d =1 cm. Find the plate area .
View Solution
