The capacity of a spherical conductor is
R4πε0
4πε0R
4πε0R
4πε0R2
The correct Answer is:C
To find the capacitance of a spherical conductor, we can follow these steps:
1. Understanding Capacitance:
- Capacitance (C) is defined as the ability of a conductor to store electric charge per unit potential difference (V) across it. Mathematically, it is given by:
C=QV
where Q is the charge on the conductor and V is the potential difference.
2. Electric Field of a Spherical Conductor:
- For a spherical conductor with charge Q, the electric field E at a distance r from the center is given by:
E=kQr2
where k is Coulomb's constant.
3. Potential Difference Calculation:
- To find the potential V at the surface of the conductor, we need to calculate the potential difference from infinity to the surface (at distance R):
V=−∫R∞Edr
- Substituting the expression for E:
V=−∫R∞kQr2dr
- This integral evaluates to:
V=−(−kQr)R∞=(0−(−kQR))=kQR
4. Substituting into Capacitance Formula:
- Now, substituting V back into the capacitance formula:
C=QV=QkQR=Rk
5. Using the Value of k:
- Recall that k=14πϵ0, where ϵ0 is the permittivity of free space. Therefore:
C=R14πϵ0=4πϵ0R
6. Final Expression for Capacitance:
- Thus, the capacitance of a spherical conductor is:
C=4πϵ0R
- Capacitance (C) is defined as the ability of a conductor to store electric charge per unit potential difference (V) across it. Mathematically, it is given by:
C=QV
where Q is the charge on the conductor and V is the potential difference.
- For a spherical conductor with charge Q, the electric field E at a distance r from the center is given by:
E=kQr2
where k is Coulomb's constant.
- To find the potential V at the surface of the conductor, we need to calculate the potential difference from infinity to the surface (at distance R):
V=−∫R∞Edr
- Substituting the expression for E:
V=−∫R∞kQr2dr
- This integral evaluates to:
V=−(−kQr)R∞=(0−(−kQR))=kQR
- Now, substituting V back into the capacitance formula:
C=QV=QkQR=Rk
- Recall that k=14πϵ0, where ϵ0 is the permittivity of free space. Therefore:
C=R14πϵ0=4πϵ0R
- Thus, the capacitance of a spherical conductor is:
C=4πϵ0R
Topper's Solved these Questions
- ELECTROSTATIC POTENTIAL AND CAPACITORSBOOK - DC PANDEY ENGLISHCHAPTER - ELECTROSTATIC POTENTIAL AND CAPACITORSEXERCISE - Check point 2.5
20 Videos
- ELECTROSTATIC POTENTIAL AND CAPACITORSBOOK - DC PANDEY ENGLISHCHAPTER - ELECTROSTATIC POTENTIAL AND CAPACITORSEXERCISE - (A) Chapter exercises
227 Videos
- ELECTROSTATIC POTENTIAL AND CAPACITORSBOOK - DC PANDEY ENGLISHCHAPTER - ELECTROSTATIC POTENTIAL AND CAPACITORSEXERCISE - Check point 2.3
15 Videos
- ELECTROMAGNETIC WAVESBOOK - DC PANDEY ENGLISHCHAPTER - ELECTROMAGNETIC WAVESEXERCISE - Sec C
22 Videos
- ELECTROSTATICSBOOK - DC PANDEY ENGLISHCHAPTER - ELECTROSTATICSEXERCISE - Medical entrances gallery
37 Videos
Similar Questions
if the capacity of a spherrical conductor is 1 μF, the diameter of the conductor will be
View SolutionA hollow spherical conductor of radius 1m has a charge of 250μC then electric intensity at a point distance of 0.5m from the centre of the spherical conductor is
View SolutionCapacitance (in F) of a spherical conductor with radius 1m is
View SolutionThe adjacent diagram shows a charge +Q held on an insulating support S and enclosed by a hollow spherical conductor,O represent the center of the spherical conductors and P is a point such that OP=x and SP=r .The electric field at point P will be
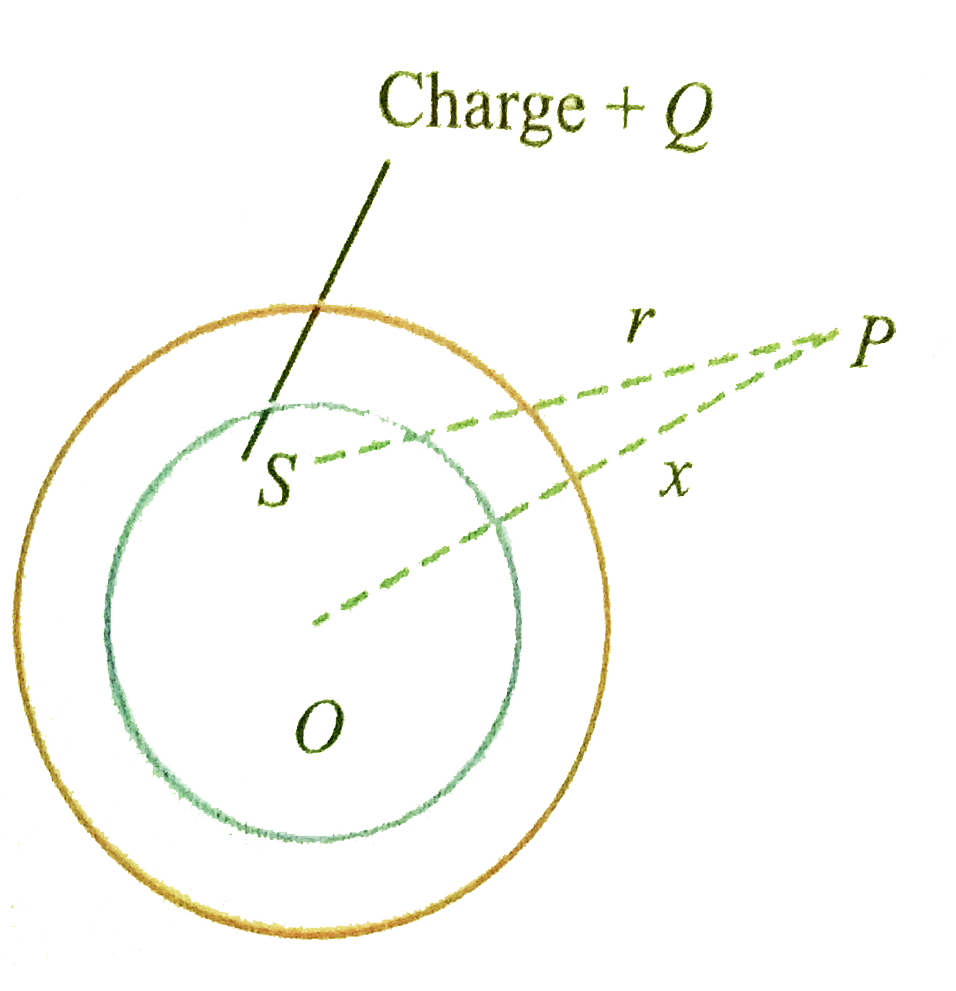
View SolutionA spherical conductor A contains two spherical cavities as shown in Fig.2.127. The total charge on conductor itself is zero . However , there is a point charge q1 at the center of one cavity and q2 at the center of the other cavity . Another charge q3 is placed at a large distance r from the center of the spherical conductor.
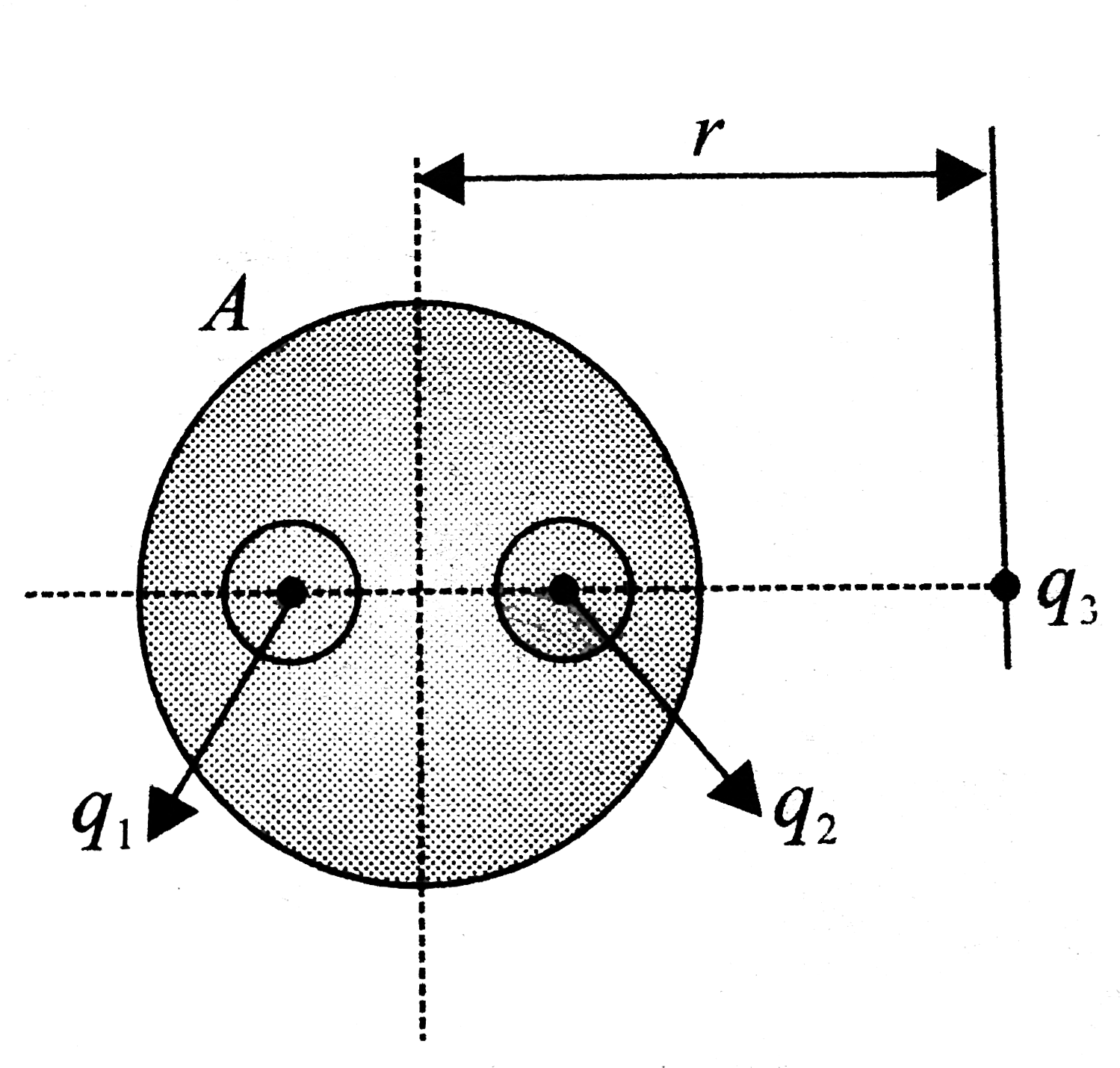
The force acting on conductor A will be
View SolutionUsing the concept of energy density, find the total energy stored in a
a. parallel plate capacitor
b. charged spherical conductor.
View SolutionA spherical conductor A contains two spherical cavities as shown in Fig.2.127. The total charge on conductor itself is zero . However , there is a point charge q1 at the center of one cavity and q2 at the center of the other cavity . Another charge q3 is placed at a large distance r from the center of the spherical conductor.
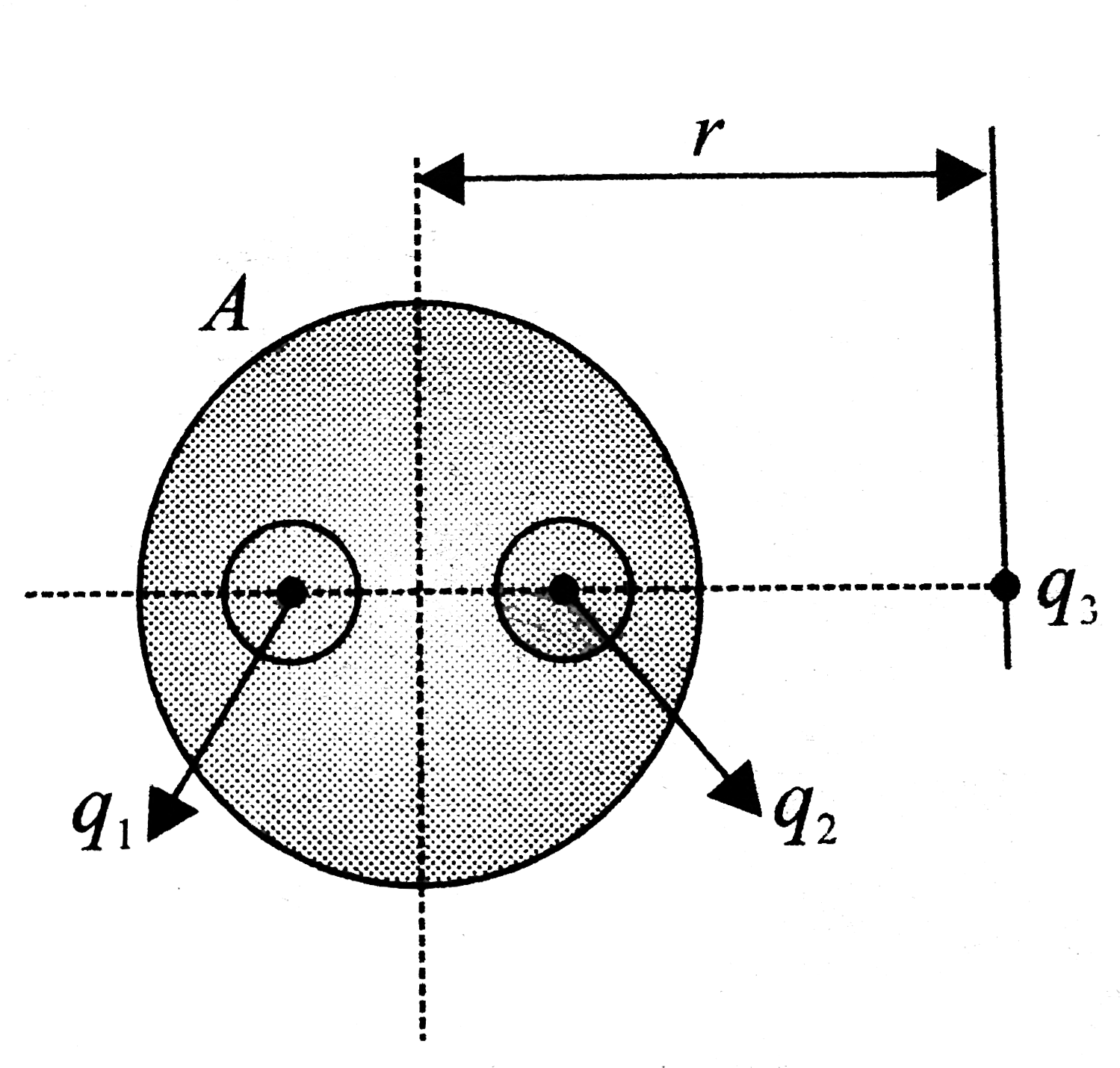
If q1 is displaced from its center slightly (being always inside the same cavity ), then the correct representation of field lines inside the same cavity is
View SolutionA spherical conductor A contains two spherical cavities as shown in Fig.2.127. The total charge on conductor itself is zero . However , there is a point charge q1 at the center of one cavity and q2 at the center of the other cavity . Another charge q3 is placed at a large distance r from the center of the spherical conductor.
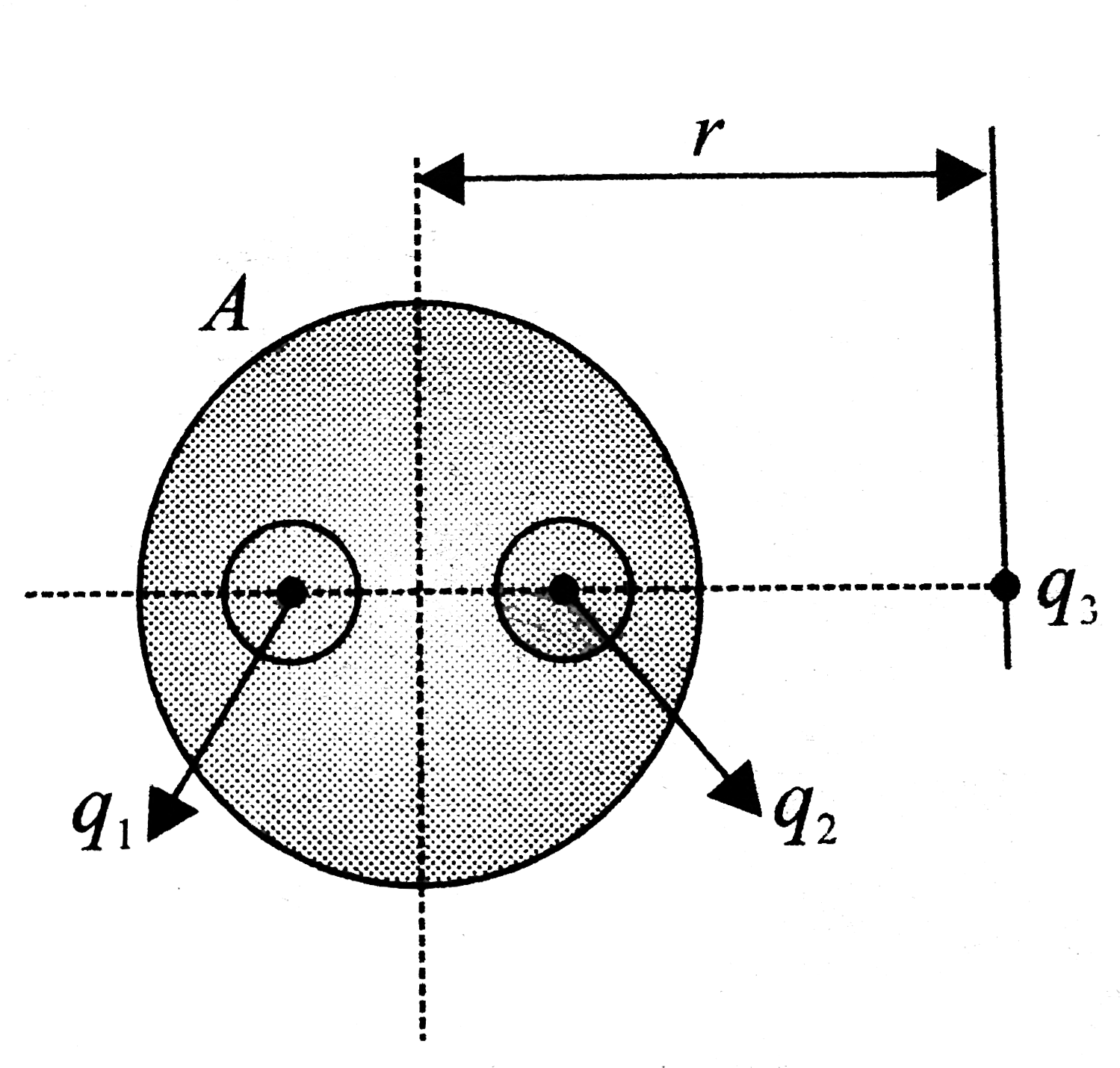
Which of the following statements are true ?
View SolutionA spherical conductor is placed near another positively charged conductor. The net charge acquired by spherical conductor will be
View SolutionFind the electrostatic force of interaction between two halves of a spherical conductor of radius R carrying a charge Q.
View Solution
