A parallel plate capacitor with air between the plates has capacitance of 9pF. The separation between its plates is 'd'. The space between the plates is now filled with two dielectrics. One of the dielectrics has dielectric constant k1=3 and thickness d3 while the other one has dielectric constant k2=6 and thickness 2d3. Capacitance of the capacitor is now
45 pF
40.5 pF
20.25 pF
1.8 pF
The correct Answer is:B
To solve the problem, we need to find the capacitance of a parallel plate capacitor that has been modified by filling the space between the plates with two different dielectrics. Here’s a step-by-step solution:
Step 1: Understand the Initial Conditions
The initial capacitance of the parallel plate capacitor with air between the plates is given as:
C0=9 pF
Step 2: Identify the Dielectrics
The space between the plates is filled with two dielectrics:
- Dielectric 1: Dielectric constant k1=3 and thickness d3
- Dielectric 2: Dielectric constant k2=6 and thickness 2d3
Step 3: Calculate the Capacitance of Each Section
The capacitance of a capacitor filled with a dielectric can be calculated using the formula:
C=k⋅Aϵ0d
Where:
- k is the dielectric constant
- A is the area of the plates
- ϵ0 is the permittivity of free space
- d is the separation between the plates
Capacitance of Dielectric 1 (C1):
For the first dielectric:
- Thickness = d3
- Dielectric constant = k1=3
Using the formula:
C1=k1⋅Aϵ0d3=3⋅Aϵ0d3=9⋅Aϵ0d
Since Aϵ0d=C0=9 pF:
C1=9⋅9 pF=81 pF
Capacitance of Dielectric 2 (C2):
For the second dielectric:
- Thickness = 2d3
- Dielectric constant = k2=6
Using the formula:
C2=k2⋅Aϵ02d3=6⋅Aϵ02d3=9⋅Aϵ0d
Again, since Aϵ0d=C0=9 pF:
C2=9⋅9 pF=81 pF
Step 4: Combine the Capacitances
The two capacitors C1 and C2 are in series. The formula for the equivalent capacitance Ceq of capacitors in series is:
1Ceq=1C1+1C2
Substituting C1 and C2:
1Ceq=181 pF+181 pF=281 pF
Step 5: Calculate the Equivalent Capacitance
Now, we can find Ceq:
Ceq=81 pF2=40.5 pF
Final Answer
The capacitance of the capacitor after filling it with the two dielectrics is:
Ceq=40.5 pF
---
The initial capacitance of the parallel plate capacitor with air between the plates is given as:
C0=9 pF
The space between the plates is filled with two dielectrics:
- Dielectric 1: Dielectric constant k1=3 and thickness d3
- Dielectric 2: Dielectric constant k2=6 and thickness 2d3
The capacitance of a capacitor filled with a dielectric can be calculated using the formula:
C=k⋅Aϵ0d
Where:
- k is the dielectric constant
- A is the area of the plates
- ϵ0 is the permittivity of free space
- d is the separation between the plates
For the first dielectric:
- Thickness = d3
- Dielectric constant = k1=3
C1=k1⋅Aϵ0d3=3⋅Aϵ0d3=9⋅Aϵ0d
C1=9⋅9 pF=81 pF
For the second dielectric:
- Thickness = 2d3
- Dielectric constant = k2=6
C2=k2⋅Aϵ02d3=6⋅Aϵ02d3=9⋅Aϵ0d
C2=9⋅9 pF=81 pF
The two capacitors C1 and C2 are in series. The formula for the equivalent capacitance Ceq of capacitors in series is:
1Ceq=1C1+1C2
1Ceq=181 pF+181 pF=281 pF
Now, we can find Ceq:
Ceq=81 pF2=40.5 pF
The capacitance of the capacitor after filling it with the two dielectrics is:
Ceq=40.5 pF
Topper's Solved these Questions
- ELECTROSTATIC POTENTIAL AND CAPACITORSBOOK - DC PANDEY ENGLISHCHAPTER - ELECTROSTATIC POTENTIAL AND CAPACITORSEXERCISE - Check point 2.5
20 Videos
- ELECTROSTATIC POTENTIAL AND CAPACITORSBOOK - DC PANDEY ENGLISHCHAPTER - ELECTROSTATIC POTENTIAL AND CAPACITORSEXERCISE - (A) Chapter exercises
227 Videos
- ELECTROSTATIC POTENTIAL AND CAPACITORSBOOK - DC PANDEY ENGLISHCHAPTER - ELECTROSTATIC POTENTIAL AND CAPACITORSEXERCISE - Check point 2.3
15 Videos
- ELECTROMAGNETIC WAVESBOOK - DC PANDEY ENGLISHCHAPTER - ELECTROMAGNETIC WAVESEXERCISE - Sec C
22 Videos
- ELECTROSTATICSBOOK - DC PANDEY ENGLISHCHAPTER - ELECTROSTATICSEXERCISE - Medical entrances gallery
37 Videos
Similar Questions
The ratio between masses of two planets is 3 : 5 and the ratio between their radii is 5 : 3. The ratio between their acceleration due to gravity will be
View SolutionA parallel plate capacitor with air between the plates has a capacitance C. If the distance between the plates is doubled and the space between the plates is filled with a dielectric of dielectric constant 6, then the capacitance will become.
View Solution
Knowledge Check
A parallel plate capacitor with air between the plates has a capacitance of 10 pF. The capacitance, if the distance bgetween the plates is reduced by half and the space between tehm is filled with a substance of dielectric constant 4 is
A80pFB96pFC100pFD120pF
A parallel plate capacitor with air between the plates has a capacitance of 8 pF.(1pF=10−12F) What will be the capacitance if the distance between the plates is reduced by half and the space between them is filled with a substance of dielectric constant 6 ?
View SolutionAn air filled parallel capacitor has capacity of 2pF. The separation of the plates is doubled and the interspaces between the plates is filled with wax. If the capacity is increased to 6pF, the dielectric constant of was is
View SolutionA parallel plate capacitor of capacitance C is charged to a potential V and then disconnected with the battery. If separation between the plates is decreased by 50% and the space between the plates is filled with a dielectric slab of dielectric constant K=10. then
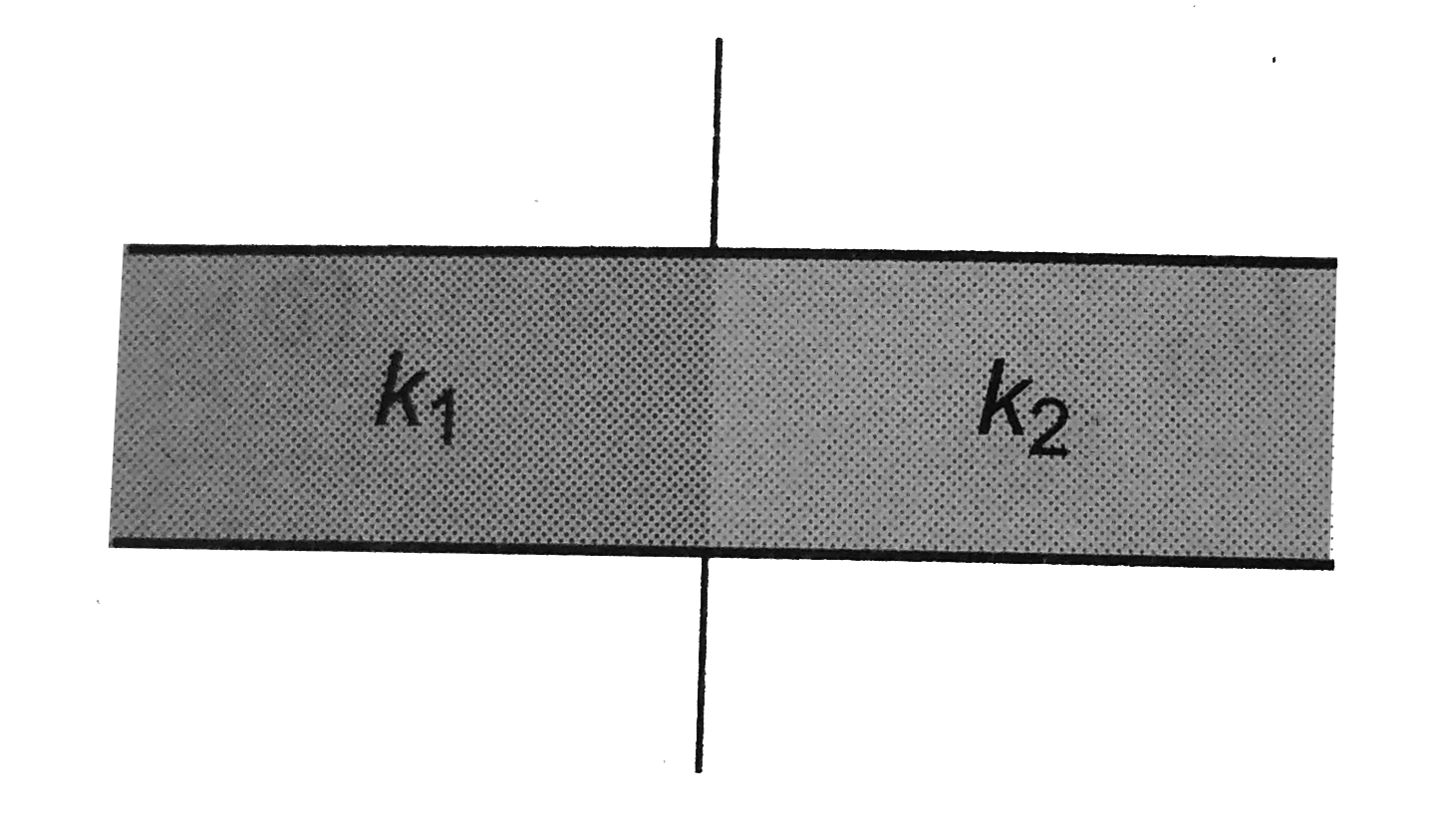
View SolutionA parallel plate capacitor with air as medium between the plates has a capacitance of 10μF. The area of capacitor is divided into two equal halves and filled with two media as shown in the figure having dielectric constnt k1=2 and k2=4. the capacitance of the system will now be
View SolutionA parallel plate capacitor has plate area A and plate separation d. The space betwwen the plates is filled up to a thickness x (ltd) with a dielectric constant K. Calculate the capacitance of the system.

View SolutionTwo parallel plate capacitors, each of capacitance 40 muF, are connected is series. The space between the plates of one capacitor is filled with a dielectric material of dielectric constant K =4. Find the equivalent capacitacne of the system.
View SolutionA parallel plate air capacitor has a capacitance of 5μF. It becomes 50μF when a dielectric medium occupies the entire space between its two plates. What is the dielectric constant of the medium ?
View Solution